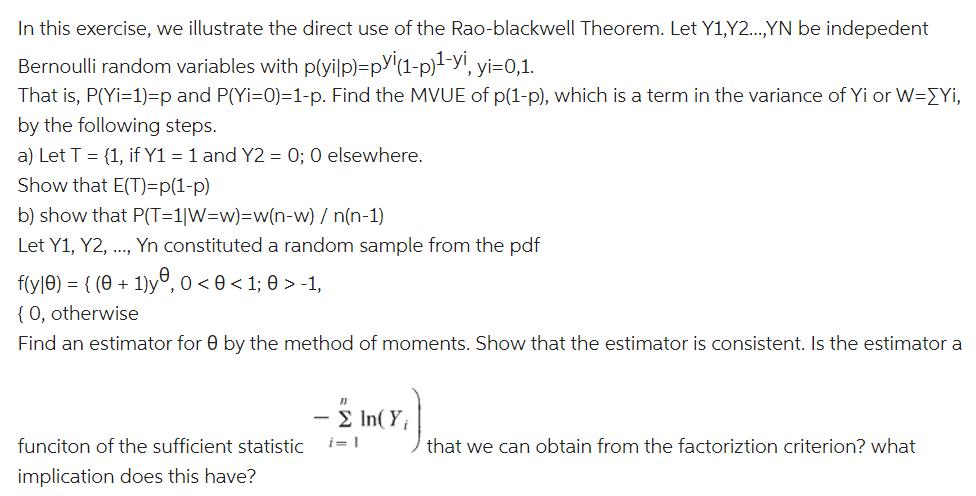
In this exercise, we illustrate the direct use of the Rao-blackwell Theorem. Let Y1,Y2.,YN be indepedent Bernoulli random variables with p(yilp)=pY(1-p)-Y!, yi=0,1. That is, P(Yi=1)=p and P(Yi=0)=1-p. Find the MVUE of p(1-p), which is a term in the variance of Yi or W=EYi, by the following steps. a) Let T = {1, if Y1 = 1 and Y2 = 0; 0 elsewhere. Show that E(T)=p(1-p) b) show that P(T=1|W=w)%3Dw(n-w) / n(n-1) Let Y1, Y2, ., Yn constituted a random sample from the pdf f(y|0) = { (0 + 1)y, 0 -1, {0, otherwise Find an estimator for 0 by the method of moments. Show that the estimator is consistent. Is the estimator a - E In( Y funciton of the sufficient statistic that we can obtain from the factoriztion criterion? what implication does this have?