Question
#include #include //srand, rand #include //clock_t, clock, CLOCKS_PER_SEC using namespace std; // implementing hash tables as an array of linked lists class Node{ private :
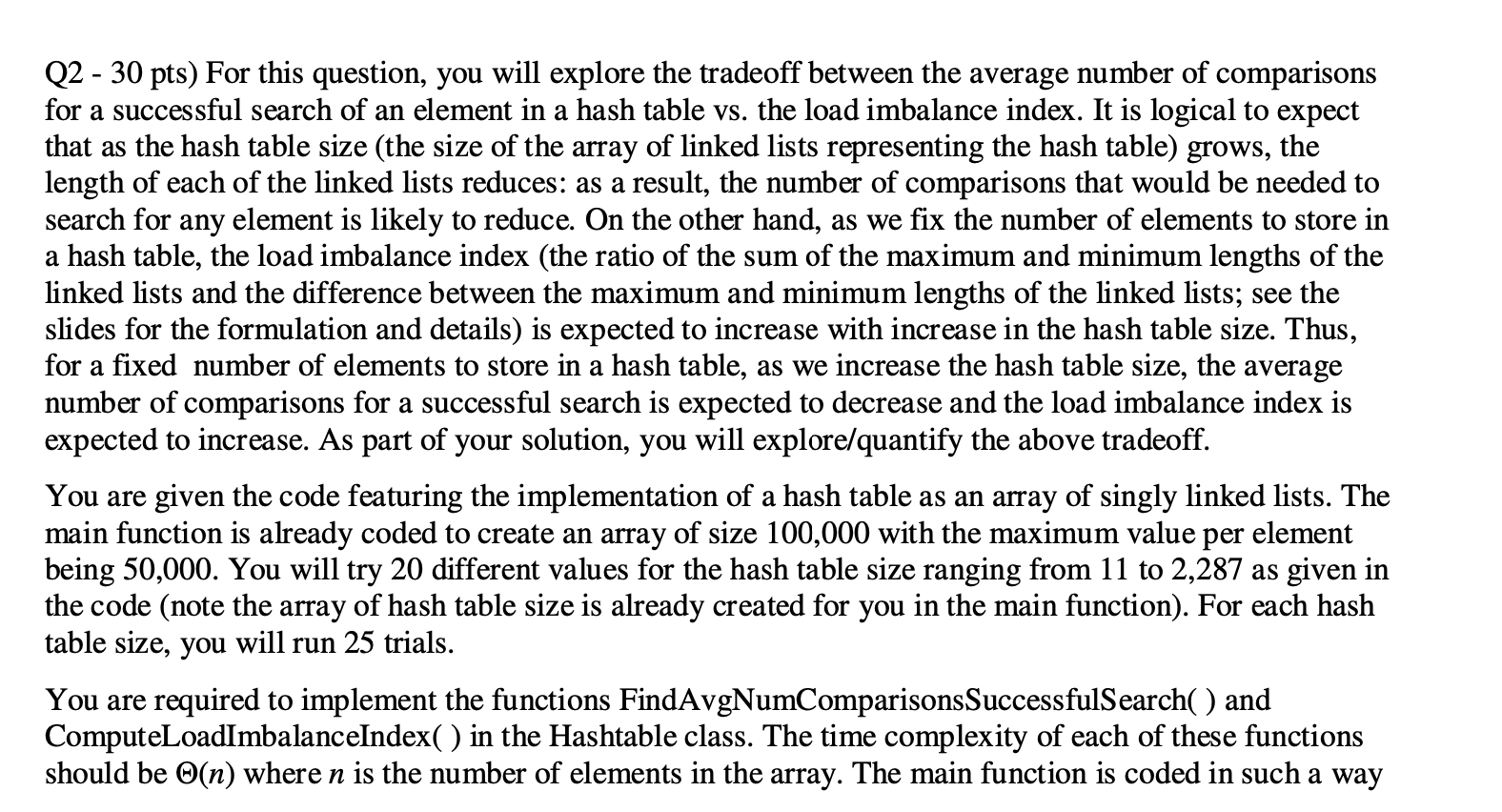
#include
#include
#include
using namespace std;
// implementing hash tables as an array of linked lists
class Node{
private:
int data;
Node* nextNodePtr;
public:
Node(){}
void setData(int d){
data = d;
}
int getData(){
return data;
}
void setNextNodePtr(Node* nodePtr){
nextNodePtr = nodePtr;
}
Node* getNextNodePtr(){
return nextNodePtr;
}
};
class List{
private:
Node *headPtr;
public:
List(){
headPtr = new Node();
headPtr->setNextNodePtr(0);
}
Node* getHeadPtr(){
return headPtr;
}
bool isEmpty(){
if (headPtr->getNextNodePtr() == 0)
return true;
return false;
}
void insert(int data){
Node* currentNodePtr = headPtr->getNextNodePtr();
Node* prevNodePtr = headPtr;
while (currentNodePtr != 0){
prevNodePtr = currentNodePtr;
currentNodePtr = currentNodePtr->getNextNodePtr();
}
Node* newNodePtr = new Node();
newNodePtr->setData(data);
newNodePtr->setNextNodePtr(0);
prevNodePtr->setNextNodePtr(newNodePtr);
}
void insertAtIndex(int insertIndex, int data){
Node* currentNodePtr = headPtr->getNextNodePtr();
Node* prevNodePtr = headPtr;
int index = 0;
while (currentNodePtr != 0){
if (index == insertIndex)
break;
prevNodePtr = currentNodePtr;
currentNodePtr = currentNodePtr->getNextNodePtr();
index++;
}
Node* newNodePtr = new Node();
newNodePtr->setData(data);
newNodePtr->setNextNodePtr(currentNodePtr);
prevNodePtr->setNextNodePtr(newNodePtr);
}
int read(int readIndex){
Node* currentNodePtr = headPtr->getNextNodePtr();
Node* prevNodePtr = headPtr;
int index = 0;
while (currentNodePtr != 0){
if (index == readIndex)
return currentNodePtr->getData();
prevNodePtr = currentNodePtr;
currentNodePtr = currentNodePtr->getNextNodePtr();
index++;
}
return -1; // an invalid value indicating
// index is out of range
}
bool deleteElement(int deleteData){
Node* currentNodePtr = headPtr->getNextNodePtr();
Node* prevNodePtr = headPtr;
Node* nextNodePtr = headPtr;
while (currentNodePtr != 0){
if (currentNodePtr->getData() == deleteData){
nextNodePtr = currentNodePtr->getNextNodePtr();
prevNodePtr->setNextNodePtr(nextNodePtr);
return true;
}
prevNodePtr = currentNodePtr;
currentNodePtr = currentNodePtr->getNextNodePtr();
}
return false;
}
int countList(){
Node* currentNodePtr = headPtr->getNextNodePtr();
int numElements = 0;
while (currentNodePtr != 0){
numElements++;
currentNodePtr = currentNodePtr->getNextNodePtr();
}
return numElements;
}
void IterativePrint(){
Node* currentNodePtr = headPtr->getNextNodePtr();
while (currentNodePtr != 0){
cout getData()
currentNodePtr = currentNodePtr->getNextNodePtr();
}
cout
}
bool containsElement(int searchData){
Node* currentNodePtr = headPtr->getNextNodePtr();
while (currentNodePtr != 0){
if (currentNodePtr->getData() == searchData)
return true;
currentNodePtr = currentNodePtr->getNextNodePtr();
}
return false;
}
};
class Hashtable{
private:
List* listArray;
int tableSize;
public:
Hashtable(int size){
tableSize = size;
listArray = new List[size];
}
int getTableSize(){
return tableSize;
}
void insert(int data){
int hashIndex = data % tableSize;
listArray[hashIndex].insert(data);
}
void deleteElement(int data){
int hashIndex = data % tableSize;
while (listArray[hashIndex].deleteElement(data));
}
bool hasElement(int data){
int hashIndex = data % tableSize;
return listArray[hashIndex].containsElement(data);
}
void printHashTable(){
for (int hashIndex = 0; hashIndex
cout
listArray[hashIndex].IterativePrint();
}
}
double FindAvgNumComparisonsSuccessfulSearch(){
// Implement the function to determine and return the
// average number of comparisons for a successful search
}
double ComputeLoadImbalanceIndex(){
// Implement the function to determine and return the load imbalance index
}
};
int main(){
int numElements = 100000;
//the number of elements you want to store in the hash table
int maxValue = 50000;
//the maximum value for an element
int numTrials = 25;
//the number of trials
int numTableSizeValues = 20;
int* hashTableSize = new int[numTableSizeValues];
hashTableSize[0] = 11;
hashTableSize[1] = 29;
hashTableSize[2] = 47;
hashTableSize[3] = 71;
hashTableSize[4] = 173;
hashTableSize[5] = 281;
hashTableSize[6] = 409;
hashTableSize[7] = 541;
hashTableSize[8] = 659;
hashTableSize[9] = 809;
hashTableSize[10] = 941;
hashTableSize[11] = 1069;
hashTableSize[12] = 1223;
hashTableSize[13] = 1373;
hashTableSize[14] = 1511;
hashTableSize[15] = 1657;
hashTableSize[16] = 1811;
hashTableSize[17] = 1987;
hashTableSize[18] = 2129;
hashTableSize[19] = 2287;
srand(time(NULL));
for (int tableSizeIndex = 0; tableSizeIndex
double totalAvgNumComparisons = 0;
double totalLoadImbalanceIndex = 0;
for (int trials = 1; trials
Hashtable hashTable(hashTableSize[tableSizeIndex]);
int *array = new int[numElements];
for (int index = 0; index
array[index] = rand() % maxValue;
hashTable.insert(array[index]);
}
totalAvgNumComparisons += hashTable.FindAvgNumComparisonsSuccessfulSearch();
totalLoadImbalanceIndex += hashTable.ComputeLoadImbalanceIndex();
}
cout
}// table size loop
system("pause");
return 0;
}
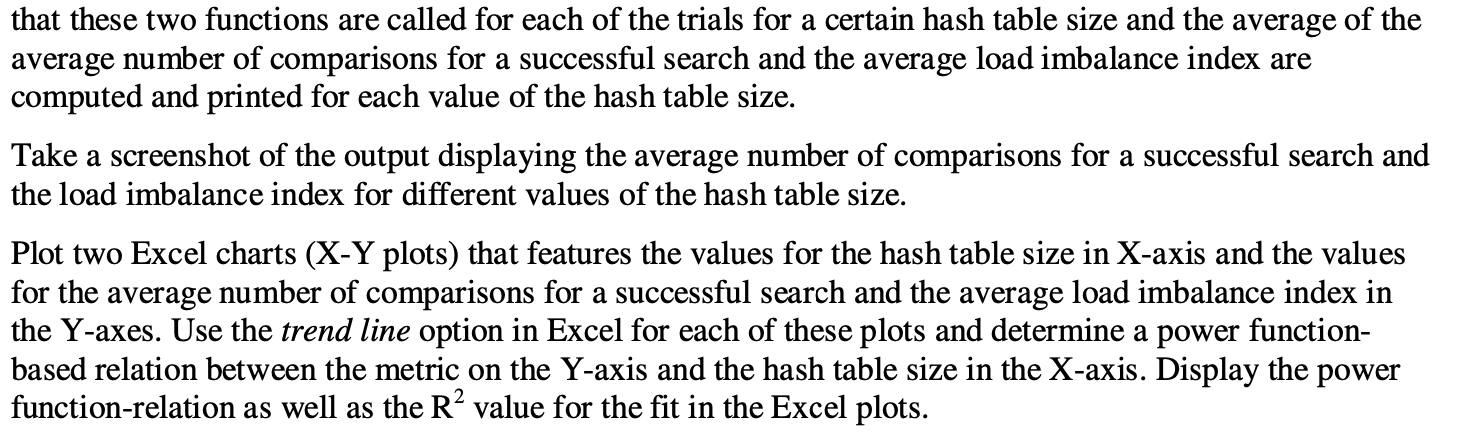
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


