Inductive proofs are challenging but have many interesting applications. In this discussion, consider problem No. 24 from Section 3.4 in your textbook. In chess, a knight moves by jumping to a square that is two units away in one direction and one unit away in another. For example, in Figure 3.18, the knight at K can move to any of the squares marked with an asterisk ().

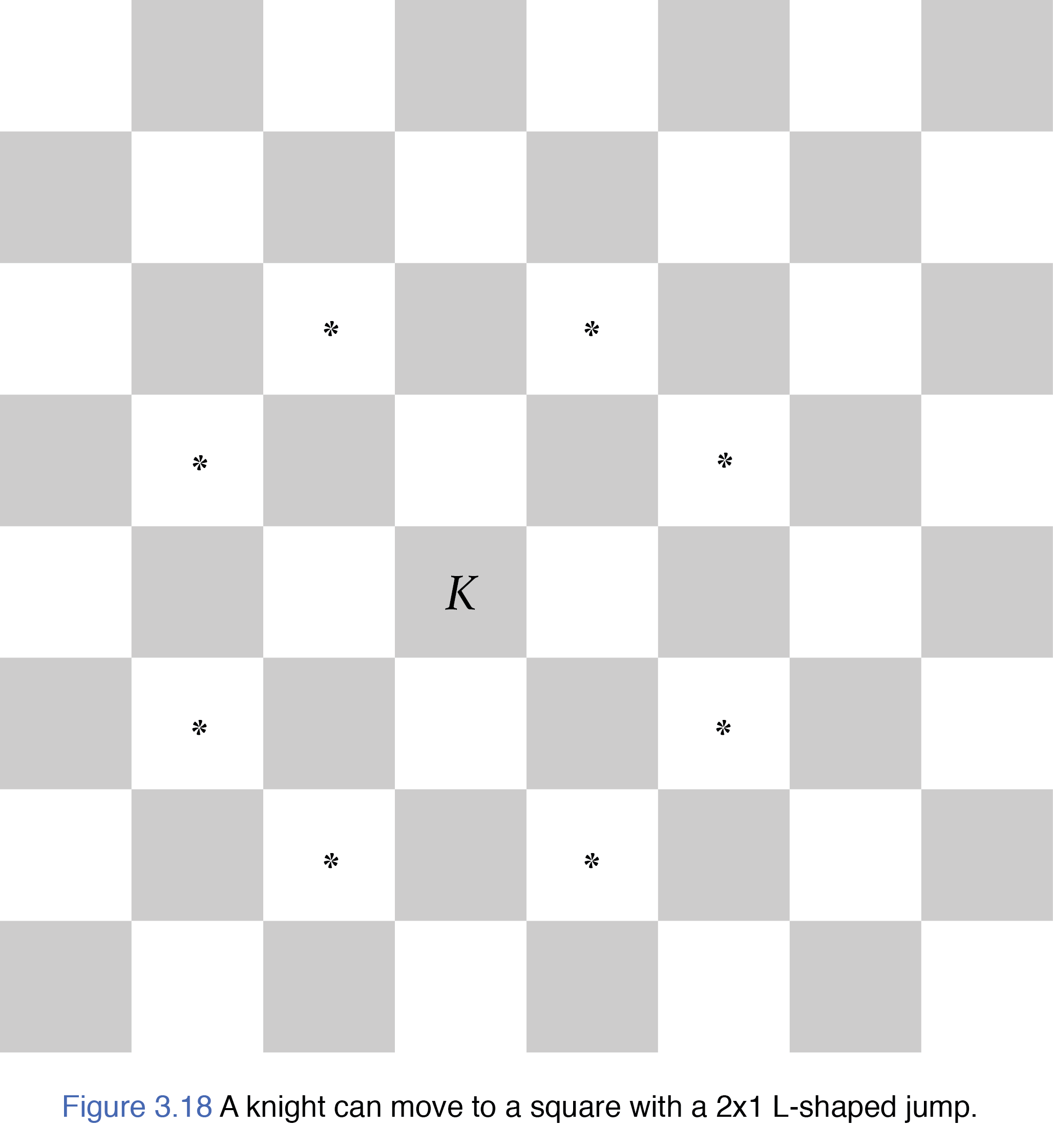
How would you explain to someone using normal written language that a knight can move from any square to any other square on a chessboard? Write your explanation and share it with your classmates.

Then, how can you use induction to prove that a knight can move from any square to any other square on an n n chessboard via a sequence of moves for all n 4?

Figure 3.18 A knight can move to a square with a 2x1 L-shaped jump