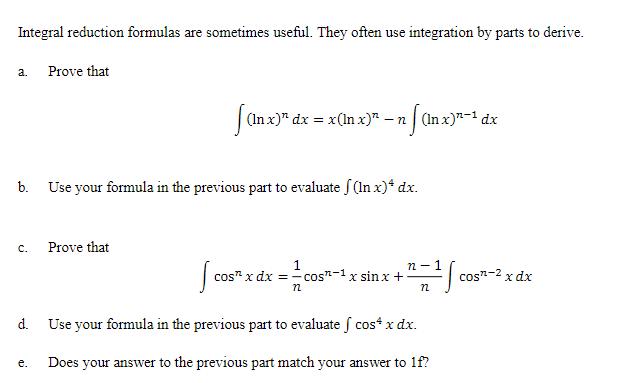
Integral reduction formulas are sometimes useful. They often use integration by parts to derive. a. b. C. d. e. Prove that [(lnx) dx = x(lnx)" - n [ (in x)-1 dx Use your formula in the previous part to evaluate (In x)* dx. Prove that n-1 + 12 = 1/ 72 1 I COST cos" x dx = -cos"-1 x sin x + n Use your formula in the previous part to evaluate / cos* x dx. Does your answer to the previous part match your answer to 1f? cos"-2 x dx