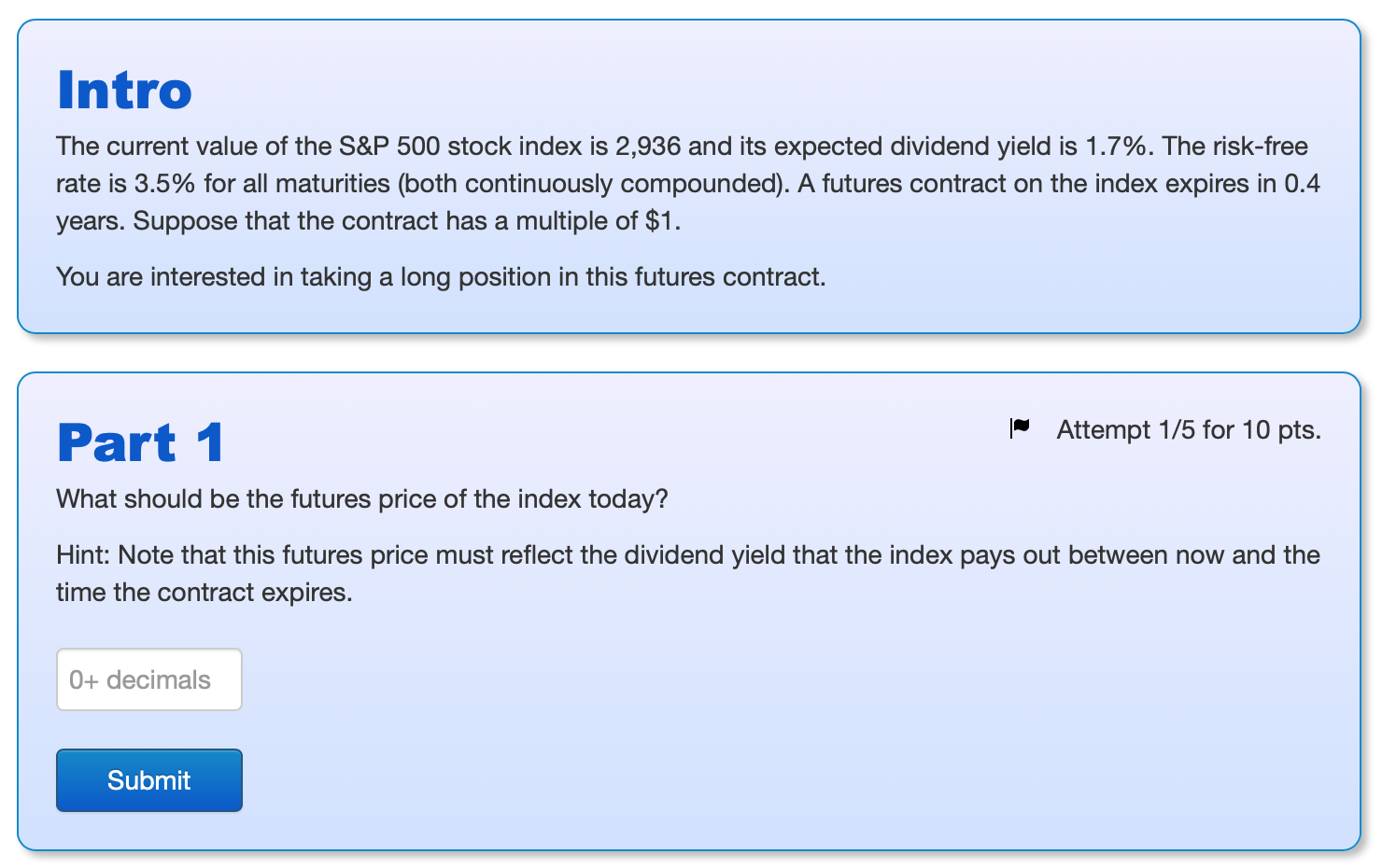
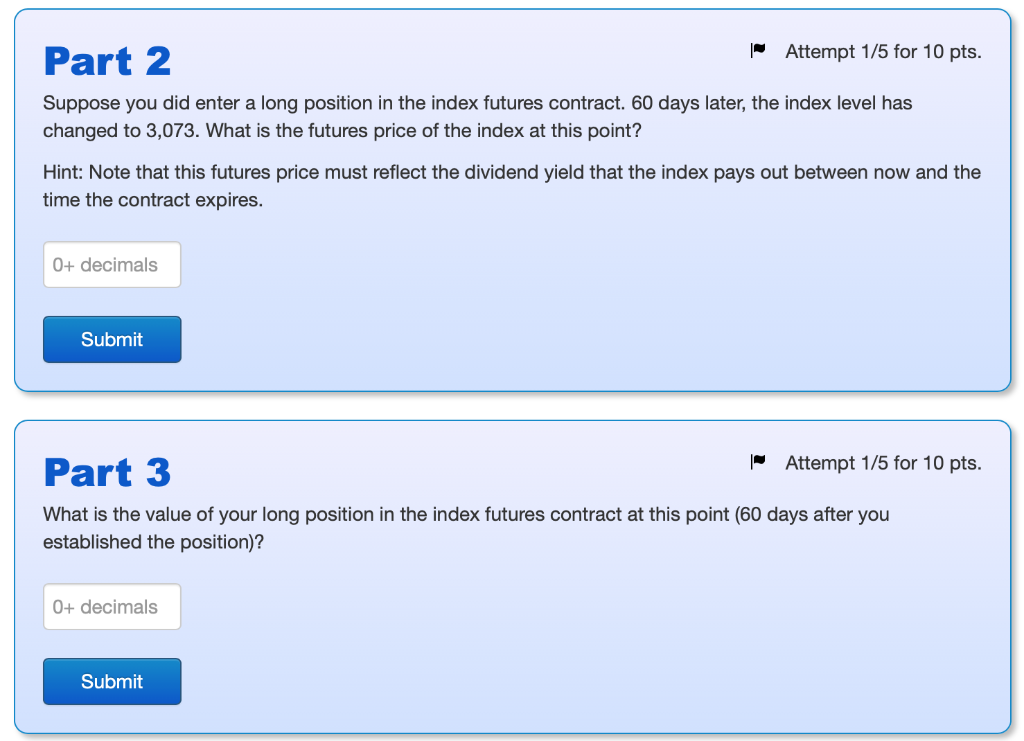
Intro The current value of the S&P 500 stock index is 2,936 and its expected dividend yield is 1.7%. The risk-free rate is 3.5% for all maturities (both continuously compounded). A futures contract on the index expires in 0.4 years. Suppose that the contract has a multiple of $1. You are interested in taking a long position in this futures contract. Part 1 | Attempt 1/5 for 10 pts. What should be the futures price of the index today? Hint: Note that this futures price must reflect the dividend yield that the index pays out between now and the time the contract expires. 0+ decimals Submit Part 2 Attempt 1/5 for 10 pts. Suppose you did enter a long position in the index futures contract. 60 days later, the index level has changed to 3,073. What is the futures price of the index at this point? Hint: Note that this futures price must reflect the dividend yield that the index pays out between now and the time the contract expires. 0+ decimals Submit 10 pts. - Attempt 1/5 Part 3 What is the value of your long position in the index futures contract at this point (60 days after you established the position)? 0+ decimals Submit Intro The current value of the S&P 500 stock index is 2,936 and its expected dividend yield is 1.7%. The risk-free rate is 3.5% for all maturities (both continuously compounded). A futures contract on the index expires in 0.4 years. Suppose that the contract has a multiple of $1. You are interested in taking a long position in this futures contract. Part 1 | Attempt 1/5 for 10 pts. What should be the futures price of the index today? Hint: Note that this futures price must reflect the dividend yield that the index pays out between now and the time the contract expires. 0+ decimals Submit Part 2 Attempt 1/5 for 10 pts. Suppose you did enter a long position in the index futures contract. 60 days later, the index level has changed to 3,073. What is the futures price of the index at this point? Hint: Note that this futures price must reflect the dividend yield that the index pays out between now and the time the contract expires. 0+ decimals Submit 10 pts. - Attempt 1/5 Part 3 What is the value of your long position in the index futures contract at this point (60 days after you established the position)? 0+ decimals Submit