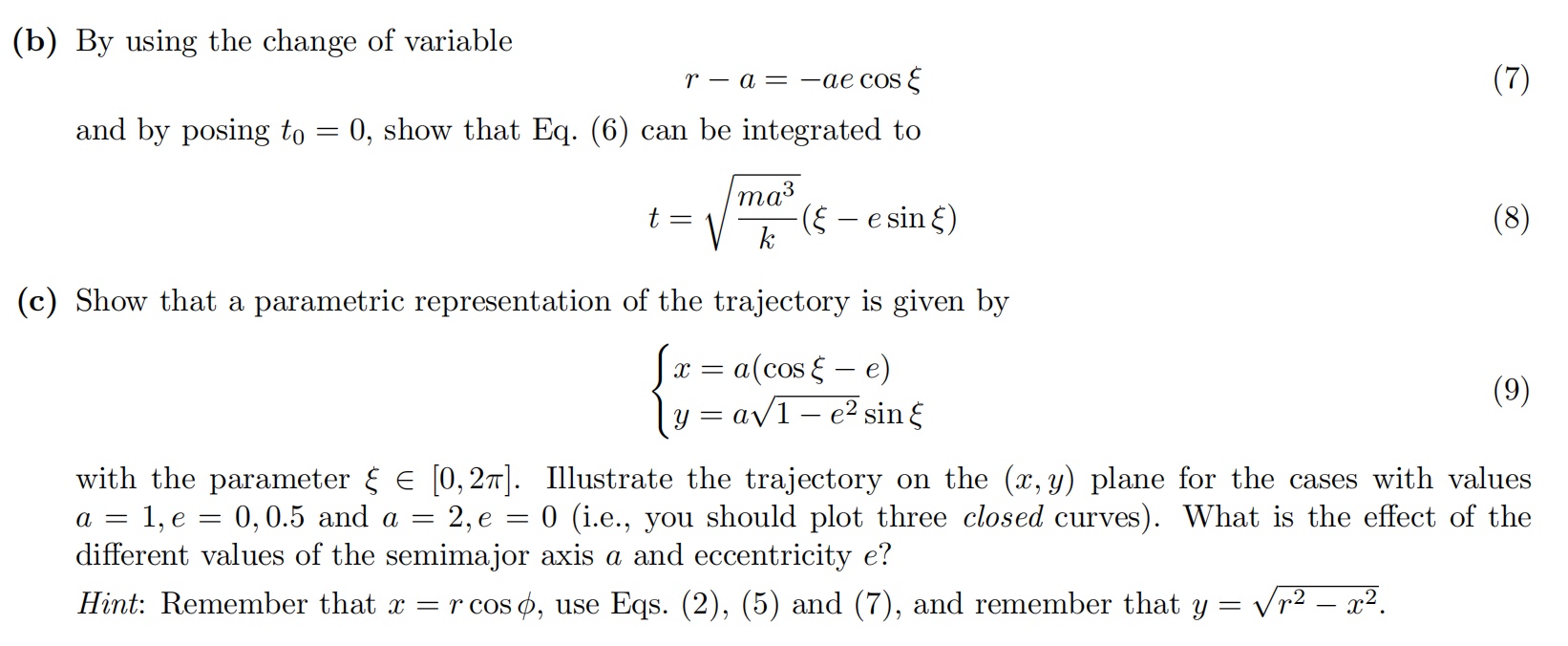
Introduction: From the study of the Kepler's problem, i.e. the motion of a material point of mass m in a gravitational field with potential energy U(r) = -k/r (see Week 2 lectures script and Chapter 3 of Landau's Mechanics), we know that: (i) the motion of the point is confined within a plane (x, y), which is orthogonal to the direction of the angular momentum of the point mass. (ii) on that plane, the relationship between the radial variable r = x + y and the scalar parameter t (time) is given by dr t- to = 2 m (B+) k m+2 (1) where E is the total energy of the point mass and lo is the value of its angular momentum. Note that both E and lo are constants because they are conserved during the motion ("integrals of motion", see Week 1 lectures). (iii) the trajectory of any orbit of the point mass can be expressed as a conic section defined by = 1 + e cos r (2) where we have introduced standard polar coordinates (r, o) on the orbital plane. Note that the parameter of the orbit and the eccentricity of the orbit p = mk e = 1+ 2E12 mk2 are also constant during the motion (because the values of E, lo, m, k are all constant). 1 Elliptic orbits Elliptic orbits are characterised by E < 0 (hence e < 1) and their semimajor axis is given by k a = = 1 - e 2|E| (a) Show that Eq. (1) can be expressed as ma t- to = k - rdr a) + a2e2 Hint: Note that E = -|E|, since E < 0 for elliptic orbits. Mind the signs! (3) (4) (5) (b) By using the change of variable ra = -ae cos and by posing to = 0, show that Eq. (6) can be integrated to ma t = ( - e sin ) (c) Show that a parametric representation of the trajectory is given by x = = a(cos - e) y = a1 e sin (7) (8) (9) with the parameter = [0,2]. Illustrate the trajectory on the (x, y) plane for the cases with values a = 1, e0, 0.5 and a = 2,e = 0 (i.e., you should plot three closed curves). What is the effect of the different values of the semimajor axis a and eccentricity e? Hint: Remember that x = r coso, use Eqs. (2), (5) and (7), and remember that = r2 = x.