Answered step by step
Verified Expert Solution
Question
1 Approved Answer
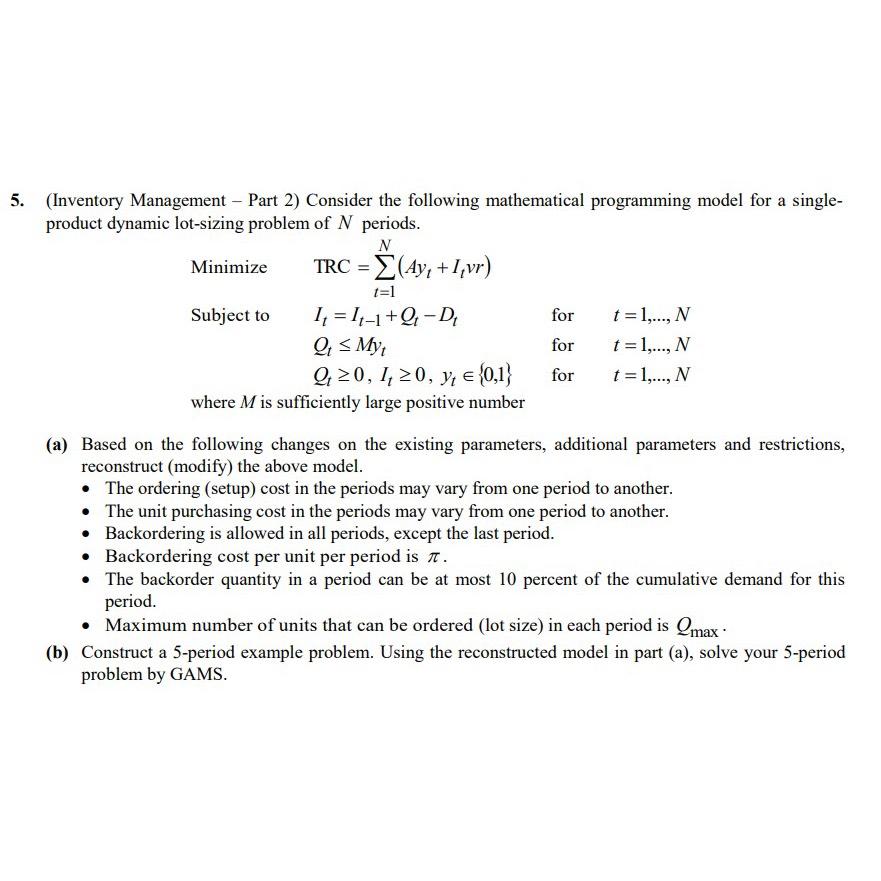
( Inventory Management - Part 2 ) Consider the following mathematical programming model for a singleproduct dynamic lot - sizing problem of N periods. Minimize
Inventory Management Part Consider the following mathematical programming model for a singleproduct dynamic lotsizing problem of periods.
Minimize
Subject for
for dots,
for dots,
where is sufficiently large positive number
a Based on the following changes on the existing parameters, additional parameters and restrictions, reconstruct modify the above model.
The ordering setup cost in the periods may vary from one period to another.
The unit purchasing cost in the periods may vary from one period to another.
Backordering is allowed in all periods, except the last period.
Backordering cost per unit per period is
The backorder quantity in a period can be at most percent of the cumulative demand for this period.
Maximum number of units that can be ordered lot size in each period is
b Construct a period example problem. Using the reconstructed model in part a solve your period problem by GAMS.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


