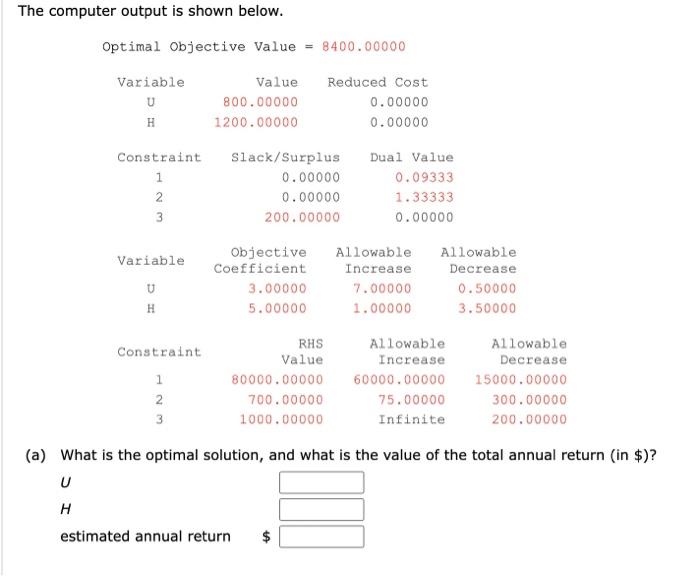
Investment Advisors, Inc., is a brokerage firm that manages stock portfolios for a number of clients. A particular pertfolio consists of U shares of U.S. Oil and H shares of Huber Steel. The annual retum for U.S. Oll is $3 per share and the annual return for Huber Steel is $5 per share. U.S. Oll sells for $25 per share and Huber Steel selis for $50 per share. The portfollo has $0,000 to be invested. The portfolio risk index (0.50 per share of U.S. Oil and 0.25 per share for Huber Steel) has a maximum of 700 , In addition, the portfolio is limited to a maximum of 1,000 shares of u.5, Of. The Inear programming formulation that will maximise the total annual return of the portfolio is as follows. return The computer output is shown below. Gptinalcojectivevalue-4400,00945 (a) What is the optimal solution, and what is the value of the total annual return (in $ )? U H estimated annual return (b) Which constraints are binding? What is your interpretation of these constraints in terms of the problem? (Select all that apply.) Constraint 1. All funds available are being utilized. Constraint 2. The maximum permissible risk is being incurred. Constraint 3. All available shares of U.S. Oil are being purchased. None of the constraints are binding. (c) What are the dual values for the constraints? Interpret each. (Round your answers to two decimal places.) constraint 1 Constraint 1 has a dual value of 3 . If an additional dollar is added to the available funds, the total annual return is predicted to increase by $3. Constraint 1 has a dual value of 5 . If an additional dollar is added to the available funds, the total annual return is predicted to increase by $5. Constraint 1 has a dual value of 0.09. If an additional dollar is added to the availabie funds, the total annual return is predicted to increase by $0.09. Constraint 1 has a slack of $200. Additional dellars added to the available funds will not improve the total annual return. Constraint 1 has a dual value of 1.33. If an additional dollar is added to the available funds, the total annual return is predicted to increase by $1.33. constraint 2 Constraint 2 has a dual value of 3 . If the risk index is increased by 1 , the total annual retum is predicted to increase by $3. Constraint 2 has a dual value of 5 . If the risk index is increased by 1 , the total annual retum is predicted to increase by $5. Constraint 2 has a dual value of 0.09. If the risk index is increased by 1 , the total annual return is predicted to increase by $0.09. Constraint 2 has a slack of 200. Allowing additional risk will not improve the total annual return. Constraint 2 has a dual value of 1,33. If the risk index is increased by 1 , the total annual return is predicted to increase by $1.33. constraint 3 Constraint 3 has a dual value of 3. If the maximum number of shares of U.S. Oil is increased by 1 , the total annual return is predicted to increase by $3. Constraint 3 has a dual value of 5 . If the maximum number of shares of U.S. Oil is increased by 1 , the total annual return is predicted to increase by $5. Constraint 3 has a dual value of 0,09 . If the maximum number of shares of U,5. Oit is increased by 1 , the total annual return is predicted to increase by 50.09. Constraint 3 has a slack of 200 shares. Raising the maximum number of shares of U.S. Oil will not improve the total annual return. Constraint 3 has a dual value of 1.33. If the maximum number of shares of U.S, Oil is increased by 1 , the total annual return is predicted to increase by $1.33, (d) Would it be beneficial to increase the maximum amount invested in U.S. Oil? Why or why not? Yes, each additional share increases the profit by $1.33. Yes, each additional share increases the profit by $0.09. Yes, each additional share increases the profit by $200.00. No, increasing the maximum shares does not affect the optimal value. Investment Advisors, Inc., is a brokerage firm that manages stock portfolios for a number of clients. A particular portfolio consists of U shares of U.S. Oil and H shares of Huber Steel. The annual return for U.S. Oil is $3 per share and the annual return for Huber $ teel is $5 per share. U.S. Oil sells for $25 per share and Huber $ teel sells for $50 per share. The portfolio has $80,000 to be invested. The portfolio risk index (0.50 per share of U.S. Oil and 0.25 per share for Huber $ teel) has a maximum of 700 . In addition, the portfolio is limited to a maximum of 1,000 shares of U.S, Oil. The linear programming formulation that will maximize the total annual retum of the portfolio is as follows. Max3U+SHS.t.25U+50H80,0000.50U+0.25H1UU,H0MaximizetotalannualretumFundsavailable700S1,000RiskmaximumU.S.Oilmaximum The computer output is shown below. (b) Which constraints are binding? What is your interpretation of these constraints in terms of the problem? (Select all that apply.) Constraint 1. All funds available are being utilized. Constraint 2 . The maximum permissible risk is being incurred. Constraint 3. All available shares of U.S. Oll are being purchased. None of the constraints are binding. (c) What are the dual values for the constraints? Interpret each. (Round your answers to two decimal places.) constraint 1 Constraint 1 has a dual value of 3 . If an additional dollar is added to the available funds, the total annual return is predicted to increase by $3. Constraint 1 has a dual value of 5 . If an additional dollar is added to the avaliable funds, the total annual return is predicted to increase by $5. Constraint 1 has a dual value of 0.09. If an additional dollar is added to the available funds, the total annual return is predicted to increase by $0.09. Constraint 1 has a slack of $200. Additional dollars added to the available funds will not improve the total annual return. Constraint 1 has a dual value of 1.33. If an additional dollar is added to the available funds, the total annual return is predicted to increase by $1.33. constraint 2 Constraint 2 has a dual value of 3 . If the risk index is increased by 1 , the total annual return is predicted to increase by $3. Constraint 2 has a dual value of 5 . If the risk index is increased by 1 , the total annual return is predicted to increase by $5. Constraint 2 has a dual value of 0.09. If the risk index is increased by 1 , the total annual return is predicted to increase by $0.09. Constraint 2 has a slack of 200 . Allowing additional risk will not improve the total annual return. Constraint 2 has a dual value of 1.33. If the risk index is increased by 1 , the total annual return is predicted to increase by $1.33. constraint 3 Constraint 3 has a dual value of 3 . If the maximum number of shares of U.S. OH is increased by 1 , the total annual return is predicted to increase by $3. Constraint 3 has a dual value of 5 . If the maximum number of shares of U.5. Oil is increased by 1 , the total annual return is predicted to increase by $5. Constraint 3 has a dual value of 0.09. If the maximum number of shares of U.S. Oil is increased by 1 , the total annual return is predicted to increase by $0.09. Constraint 3 has a slack of 200 shares. Raising the maximum number of shares of U.S. Oil will not improve the total annual return. Constraint 3 has a dual value of 1.33. If the maximum number of shares of U.S. Oil is increased by 1 , the total annual return is predicted to increase by $1.33. 1) Would it be beneficial to increase the maximum amount invested in U.S. Oil? Why or why not? Yes, each additional share increases the profit by $1.33. Yes, each additional share increases the profit by $0.09. Yes, each additional share increases the profit by $200.00. No, increasing the maximum shares does not affect the optimal value