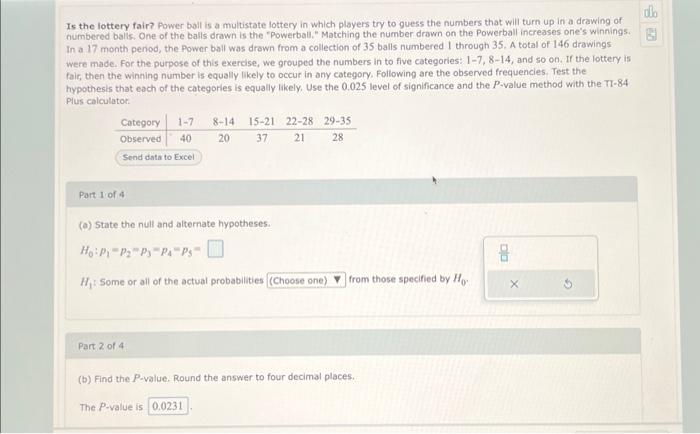
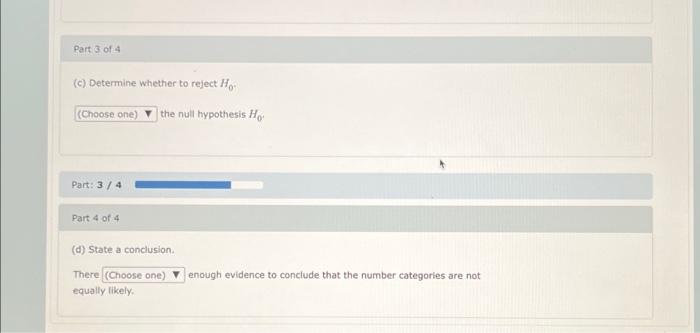
Is the lottery fair? Power ball is a multistate lottery in which players try to guess the numbers that will turn up in a drawing of numbered balls. One of the balls drawn is the "Powerball." Matching the number drawn on the Powerball increases one's winnings. In a 17 month period, the Power ball was drawn from a collection of 35 balls numbered 1 through 35 . A total of 146 drawings were made. For the purpose of this exercise, we grouped the numbers in to five categories: 1-7, 8-14, and so on. If the lottery is faic, then the winning number is equally likely to occur in any category. Following are the observed frequencies. Test the hypothests that each of the categories is equally likely. Use the 0.025 level of significance and the P-value method with the TI-84 Plus calculator. Part 1 of 4 (a) State the null and alternate hypotheses. H0:p1=p2=p3=p4=p5= H1: Some or all of the actual probabilities from those specified by H0. Part 2 of 4 (b) Find the P-value. Round the answer to four decimal places. The P-value is (c) Determine whether to reject H0 : the null hypothesis H0 Part: 3/4 Part 4 of 4 (d) State a conclusion. There enough evidence to conclude that the number categorles are not equally likely. Is the lottery fair? Power ball is a multistate lottery in which players try to guess the numbers that will turn up in a drawing of numbered balls. One of the balls drawn is the "Powerball." Matching the number drawn on the Powerball increases one's winnings. In a 17 month period, the Power ball was drawn from a collection of 35 balls numbered 1 through 35 . A total of 146 drawings were made. For the purpose of this exercise, we grouped the numbers in to five categories: 1-7, 8-14, and so on. If the lottery is faic, then the winning number is equally likely to occur in any category. Following are the observed frequencies. Test the hypothests that each of the categories is equally likely. Use the 0.025 level of significance and the P-value method with the TI-84 Plus calculator. Part 1 of 4 (a) State the null and alternate hypotheses. H0:p1=p2=p3=p4=p5= H1: Some or all of the actual probabilities from those specified by H0. Part 2 of 4 (b) Find the P-value. Round the answer to four decimal places. The P-value is (c) Determine whether to reject H0 : the null hypothesis H0 Part: 3/4 Part 4 of 4 (d) State a conclusion. There enough evidence to conclude that the number categorles are not equally likely