Kindly help me to solve this question. Thank you

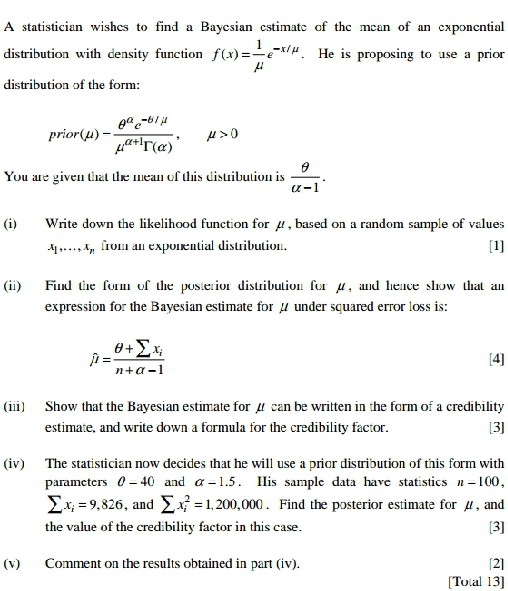
A statistician wishes to find a Bayesian estimate of the mean of an exponential distribution with density function f(x)=x. He is proposing to use a prior distribution of the form: prior(u)- 4a+T() P>0 You are given that the mean of this distribution is -1 (i) Write down the likelihood function for, based on a random sample of values from an exponential distribution. [1] (ii) Find the form of the posterior distribution for u, and hence show that an expression for the Bayesian estimate for under squared error loss is: + = n+a-l [4] (iii) Show that the Bayesian estimate for can be written in the form of a credibility estimate, and write down a formula for the credibility factor. [3] (iv) The statistician now decides that he will use a prior distribution of this form with parameters 0-40 and a -1.5. His sample data have statistics n-100, x=9,826, and x = 1,200,000. Find the posterior estimate for , and (v) the value of the credibility factor in this case. Comment on the results obtained in part (iv). [3] [2] [Total 13]