Question: Let 0 < t < 1 be a fixed real number. Let B E M (R) be the matrix M-P) 1-t B = t

Let 0 < t < 1 be a fixed real number. Let

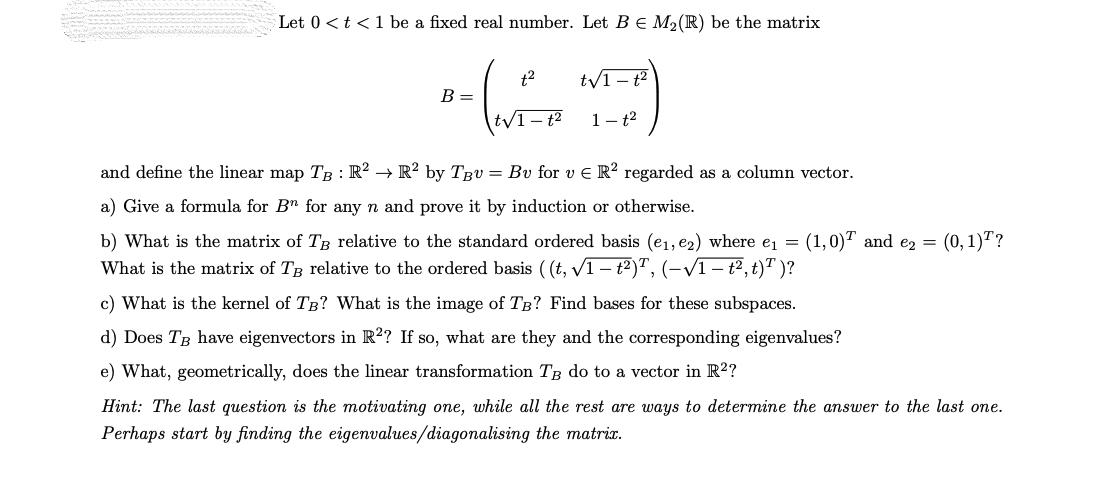
Let 0 < t < 1 be a fixed real number. Let B E M (R) be the matrix M-P) 1-t B = t t1-t and define the linear map TB: R2 R by TBv = Bu for v E R regarded as a column vector. a) Give a formula for Bn for any n and prove it by induction or otherwise. b) What is the matrix of TB relative to the standard ordered basis (e,e2) where e = What is the matrix of T relative to the ordered basis ((t, 1 1), (-1-t, t))? c) What is the kernel of TB? What is the image of TB? Find bases for these subspaces. d) Does TB have eigenvectors in R2? If so, what are they and the corresponding eigenvalues? e) What, geometrically, does the linear transformation TB do to a vector in R? (1,0) and e = (0, 1)T? Hint: The last question is the motivating one, while all the rest are ways to determine the answer to the last one. Perhaps start by finding the eigenvalues/diagonalising the matrix.

Step by Step Solution

★★★★★

3.39 Rating (161 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

a To find a formula for Bn we can use the concept of diagonalization Lets diagonalize the matrix B b... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Let Î¸ be a fixed real number and let (a) Show that {x1, x2) is an orthonormal basis for R2. (b) Verify that c21 + c22 = ||y||2 = y2 + y22 cos sin -sin x,-| cos Xi and

The following additional information is available for the Dr. Ivan and Irene Incisor family from Chapters 1-5. Ivan's grandfather died and left a portfolio of municipal bonds. In 2012, they pay Ivan...

Determine the following integrals: Jsin (30)d0 0

On a computer multiplications have traditionally been more costly than additions, so people have tried to in reduce the number of multiplications used to compute a matrix product. (a) How many real...

Let a, b denote fixed real numbers and suppose that R R is defined by f(x) = a (x +b) - b, x R. (a) Determine f2(x) and f3(x). (b) Conjecture a formula for fn(x), where n Z+. Now establish the...

All good experiments start with a scientific question. What was the scientific question that Art Fry was trying to answer when he embarked on finding the adhesive for the Post-it Note?

Consider the ellipsoid $E=\left\{\boldsymbol{x} \in \mathbb{R}^{d}: x \boldsymbol{\Sigma}^{-1} \boldsymbol{x}=1 ight\}$ in (4.42). Let $\mathbf{U D}^{2} \mathbf{U}^{\top}$ be an SVD of...

An iron core shaped as a tore with mean radius R = 250 mm supports a winding with the total number of turns N = 1000. The core has a cross-cut of width b = 1.00 ram. With a current I = 0.85 A flowing...

Let f(x) = ax(1 - x), where a is a real number and 0 x 1. Recall that the fixed point of a function is a value of x such that f(x) = x. a. Without using a calculator, find the values of a, with 0

A box containing a particle is divided into a right and left compartment by a thin partition. If the particle is known to be on the right (left) side with certainty, the state is represented by the...

Halcyon Lines is considering the purchase of a new bulk carrier for $6.6 million. The forecasted revenues are $7.0 million a year and operating costs are $6.0 million. A major refit costing $4.0...

You have a conference room that contains a Teams Rooms on Windows device. You need to update the background image of the device with new company branding. What should you do? Select only one answer....

How many moving trucks, professional movers, and moving cost would it take to support supporting a Sensitive Compartmented Information Facility (SCIF) move to another building? Use the following...

What is the speedup of the basic pipeline (without bypassing) shown in the Introduction slides (Tsequential / Tpipelined) if 1/5 of all instructions contain a data dependence? Can you give a general...

Write a one hundred and fifty word response about which resource(s) you found to be most useful in terms of not only researching the different types of networks that exist, but which might be best...

Person is a named tuple with fields: first_name, last_name, and age. Variable person_data is a Person object created with two strings and one integer read from input as attributes. Output the...

Which of the following is NOT an example of a company's transaction processing system? A . UPS package tracking system B . Marriott reservation system C . Delta flight reservation system D ....

Reduction in sales All of the above 29. Belt of an electric motor is broken, it needs a. Corrective maintenance b. Scheduled maintenance c. Preventive maintenance d. Timely maintenance. 30. The...

If Q is an n x n orthogonal matrix and x and y are nonzero vectors in Rn, then how does the angle between Qx and Qy compare to the angle between x and y? Prove your answer.

Set A = magic(4) and t = trace(A). The scalar t should be an eigenvalue of A, and the remaining eigenvalues should add up to zero. Why? Explain. Use MATLAB to verify that A - tl is singular. Compute...

Prove the Frobenius theorem in the case where A is a 2 2 matrix.

Nowjuice, Inc., produces Shakewell fruit juice. A planner has developed an aggregate forecast for demand (in cases) for the next six months. Develop an aggregate plan using each of the following...

Manager Chris Channing of Fabric Mills, Inc., has developed the forecast shown in the table for bolts of cloth. The figures are in hundreds of bolts. The department has a regular output capacity of...

Refer to Solved Problem 1. Prepare two additional aggregate plans. Call the one in the solved problem plan A. For plan B, hire one more worker at a cost of $ 200. Make up any shortfall using...