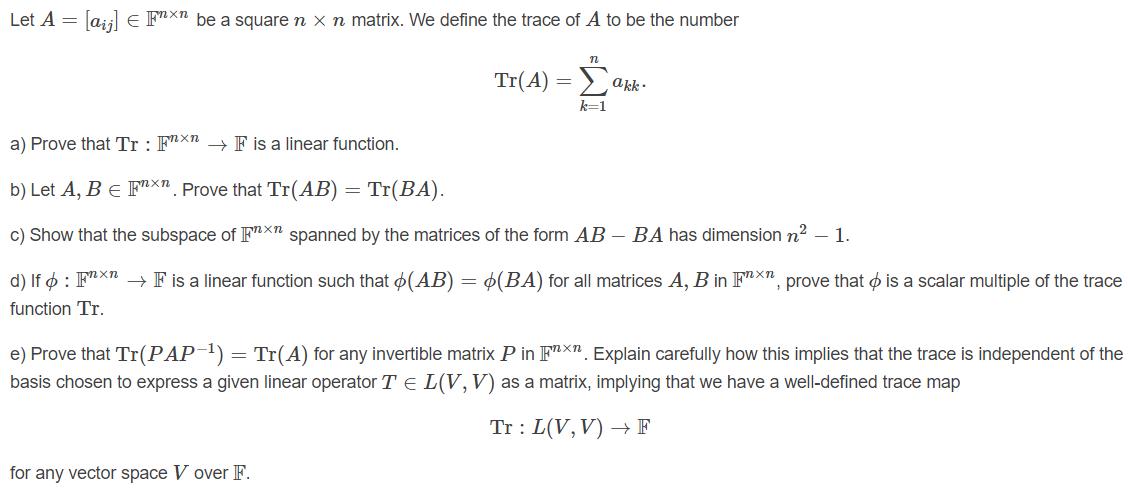
Let A = [a;l E Fxn be a square n x n matrix. We define the trace of A to be the number Tr(A) = akk- k=1 a) Prove that Tr : FXn F is a linear function. b) Let A, B e F"X". Prove that Tr(AB) = Tr(BA). c) Show that the subspace of F"xn spanned by the matrices of the form AB - BA has dimension n? 1. d) If o : F"Xn F is a linear function such that o(AB) = o(BA) for all matrices A, B in F"X", prove that o is a scalar multiple of the trace function Tr. e) Prove that Tr(PAP1) = Tr(A) for any invertible matrix P in FXn. Explain carefully how this implies that the trace is independent of the basis chosen to express a given linear operator TE L(V, V) as a matrix, implying that we have a well-defined trace map Tr : L(V, V) F for any vector space V over F.