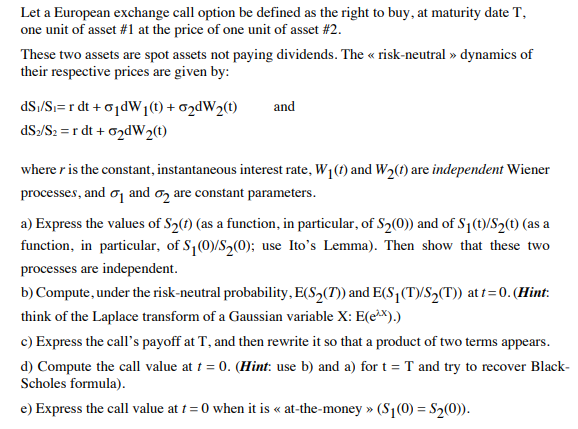
Let a European exchange call option be defined as the right to buy, at maturity date T, one unit of asset #1 at the price of one unit of asset #2. These two assets are spot assets not paying dividends. The risk-neutral dynamics of their respective prices are given by: dS /S=r dt+010W1(t) + O2dW2(t) and dS,/S2 =r dt +620W 2(t) where r is the constant, instantaneous interest rate, W7 (1) and W2(t) are independent Wiener processes, and 1 and 2 are constant parameters. a) Express the values of S2(1) (as a function, in particular, of S2(0)) and of Sj(t)/S2(t) (as a function, in particular, of S70/520); use Ito's Lemma). Then show that these two processes are independent. b) Compute, under the risk-neutral probability, E(S2(T)) and E(S_(T)S_(T)) at t=0. (Hint: think of the Laplace transform of a Gaussian variable X: E(**).) c) Express the call's payoff at T, and then rewrite it so that a product of two terms appears. d) Compute the call value at t = 0. (Hint: use b) and a) for t = T and try to recover Black- Scholes formula). e) Express the call value at t=0 when it is at-the-money (S1(0) = S200)). Let a European exchange call option be defined as the right to buy, at maturity date T, one unit of asset #1 at the price of one unit of asset #2. These two assets are spot assets not paying dividends. The risk-neutral dynamics of their respective prices are given by: dS /S=r dt+010W1(t) + O2dW2(t) and dS,/S2 =r dt +620W 2(t) where r is the constant, instantaneous interest rate, W7 (1) and W2(t) are independent Wiener processes, and 1 and 2 are constant parameters. a) Express the values of S2(1) (as a function, in particular, of S2(0)) and of Sj(t)/S2(t) (as a function, in particular, of S70/520); use Ito's Lemma). Then show that these two processes are independent. b) Compute, under the risk-neutral probability, E(S2(T)) and E(S_(T)S_(T)) at t=0. (Hint: think of the Laplace transform of a Gaussian variable X: E(**).) c) Express the call's payoff at T, and then rewrite it so that a product of two terms appears. d) Compute the call value at t = 0. (Hint: use b) and a) for t = T and try to recover Black- Scholes formula). e) Express the call value at t=0 when it is at-the-money (S1(0) = S200))