Let A Rx3 and suppose that 3 = (v, U2, U3) is an ordered basis for R consisting of eigenvectors for A. So Av
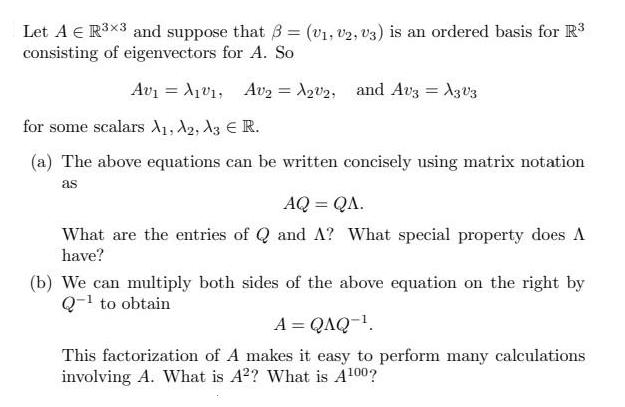
Let A Rx3 and suppose that 3 = (v, U2, U3) is an ordered basis for R consisting of eigenvectors for A. So Av = A1v, Av2 = 22, and Av3 = A3V3 for some scalars A, A2, A3 R. (a) The above equations can be written concisely using matrix notation as AQ = QA. What are the entries of Q and A? What special property does A have? (b) We can multiply both sides of the above equation on the right by Q- to obtain A = QAQ-. This factorization of A makes it easy to perform many calculations involving A. What is A2? What is A100?
Step by Step Solution
3.47 Rating (150 Votes )
There are 3 Steps involved in it
Step: 1
a Entries of Q and and the special property of Matrix Q Q is a 3x3 matrix wh...
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2
Step: 3
Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started
Authors: Gerald E. Whittenburg, Martha Altus Buller, Steven L Gill
31st Edition
1111972516, 978-1285586618, 1285586611, 978-1285613109, 978-1111972516
