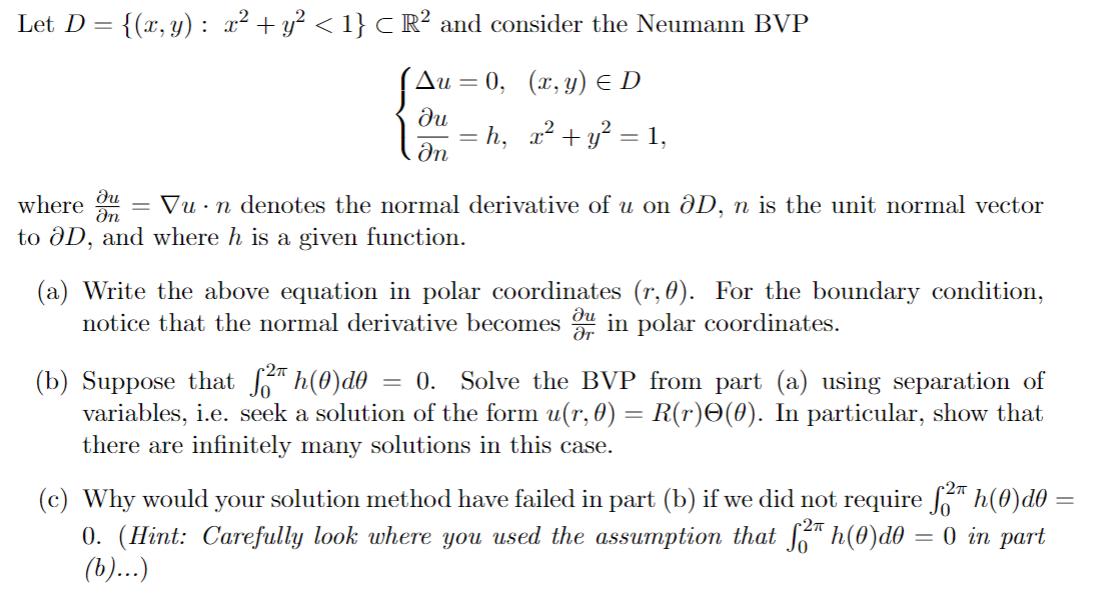
Let D= {(x, y): x + y < 1} C R and consider the Neumann BVP Au=0, (x,y) E D h, x + y = 1, where - Vu n denotes the normal derivative of u on OD, n is the unit normal vector to OD, and where h is a given function. (a) Write the above equation in polar coordinates (r, 0). For the boundary condition, notice that the normal derivative becomes in polar coordinates. (b) Suppose that 2 h(0)d0 = 0. Solve the BVP from part (a) using separation of variables, i.e. seek a solution of the form u(r, 0) = R(r)O(0). In particular, show that there are infinitely many solutions in this case. (c) Why would your solution method have failed in part (b) if we did not require f2" h(0)do 0. (Hint: Carefully look where you used the assumption that 2 h(0)d0 = 0 in part (b)...) =