Question: Let f (x) be a function that is differentiable everywhere and has a derivative f'(x) = 3x + 14z + 6. Verify that the

a derivative f'(x) = 3x + 14z + 6. Verify that the

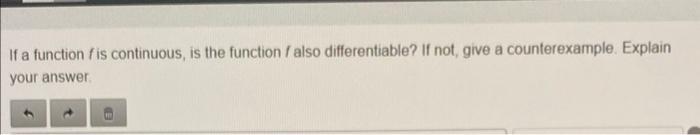
Let f (x) be a function that is differentiable everywhere and has a derivative f'(x) = 3x + 14z + 6. Verify that the Intermediate Value Theorem for Derivatives applies to the function f'(x) on the interval [-6,-3], and find the value of c guaranteed by the theorem such that f'(c) = -2 If a function fis continuous, is the function / also differentiable? If not, give a counterexample. Explain your answer

Step by Step Solution

★★★★★

3.36 Rating (149 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

4. Dependable Rentals Ltd consists of two divisions. The Equipment Rental Division rents machinery, such as cement mixers and scissor lifts, to building contractors. The Truck Rental Division rents...

Let f(x) be a function that possesses at least n derivatives at x = a and let Pn(x) be the Taylor polynomial of order n based at a. Show that Pn(a) = f(a), P'n(a) = f'(a), P"n(a) = f"(a), ... P(n)n...

Let f(x) be a function that is defined and has a continuous derivative on the interval (2,). Assume also that f(4) = 14, |f(x)|

Please write the answer in a way I can copy it, not on paper. Let f (x) be a function that is differentiable everywhere and has a derivative f' (x) = 3x2 + 14x + 6. Verify that the Intermediate Value...

Let $\varphi$ be in $C_{b}^{1}$. Prove that $\left(\varphi\left(L_{t}^{0} ight)-\left|B_{t} ight| \varphi^{\prime}\left(L_{t}^{0} ight), t \geq 0 ight)$ is a martingale. Let \(T_{a}^{*}=\inf...

3 2 points Let f x y 1 a Find a direction u such that Duf 0 1 0 b At which point s x y is the function s direction of greatest growth parallel the line y x

3-67 (Every fourth, and also 61, 65, 69; pp.309-310 ) SECTION 3.3 . Dividing Polynomials 309 EXAMPLE 5 = Factoring a Polynomial Using the Factor Theorem 1/10 -7 6 Let P(x) = x - 7x + 6. Show that P(...

CONCORDIA UNIVERSITY Department of Mathematics & Statistics Course Number Sections Mathematics 203 All Examination Date Pages Final April 2019 3 Instructors: A. Butaev, C. David, U. Mgbemena, B....

Need Help? Read It Watch It [0/1 Points] DETAILS PREVIOUS ANSWER Let y = f(x), where f(x) = 6x3/2 + x1/2. Find the differential of the function. 19 dy = 2 X Need Help? Read It Watch ItRelated Rates...

Question 1 1 pts (EXPLANATION) A student is trying to find the following limit: Vac2 lim -0 4x - The student simplifies the numerator to x and replaces the denominator with the leading term 4x. lim...

Assignment 9 Harassment, OSHA, Workers' Compensation (Materials in Week 13 Folder) Sexual harassment is a form of discrimination and covered under Title VII, as well as California statutes. And let's...

Within the northeastern control systems industry, Middleton knew of no company that had conducted formal market research. Should he conduct further research himself, use the consultant or the student...

Check billing and accounts recordsPlease review the following list of billing and account records from Table 4: Gelos Enterprises bank statement for its Small Purchases account. Check these billing...

3-37. In a motion capture study of a runner, one frame shows the subject support- ing her weight on one leg, as shown in Fig. P3.37. The length of the foot seg- ment (from ankle to toe) is 8 in. and...

Exhibit 12-1 A square of side 2 is removed from one corner of a square sandwich that has sides of length L. The center of mass of th remainder of the sandwich moves from C to C'. Use this exhibit to...

5. A consumer decides not to buy a VCR when her income is $20,000. However, when her income rises to $30,000, she decides to buy one. In a single diagram, draw the budget lines and indifference...

Simon Layman is a trust investment officer at a bank in a small affluent town. He enjoys lunching every day with friends at the country club, where his clients have observed him having numerous...

Reconsider Prob. 1474. In order to drain the tank faster, a pump is installed near the tank exit as in Fig. P1475. Determine how much pump power input is necessary to establish an average water...

Find b such that (2,1), (3, 2), and (b, 5) lie on a line

Find a constant c for which the velocity field Of a fluid is incompressible [meaning that div(v) = 0]? cry

For f (x) with derivative as in Figure 12: (a) Is f (c) a local minimum or maximum? (b) Is f (x) a decreasing function? FIGURE 12 Graph of derivative f'(x)

The following data are the population of ages of students who have recently purchased a sports video game: a. Compute the population variance. b. Compute the population standard deviation.

Consider the following two separate samples: a. Calculate the range, variance, standard deviation, and interquartile range for each data set. b. Which data set is most spread out based on these...

A European cereal maker recently sampled 20 of its medium-size oat cereal packages to determine the weights of the cereal in each package. These sample data, measured in ounces, are as follows:...