Question
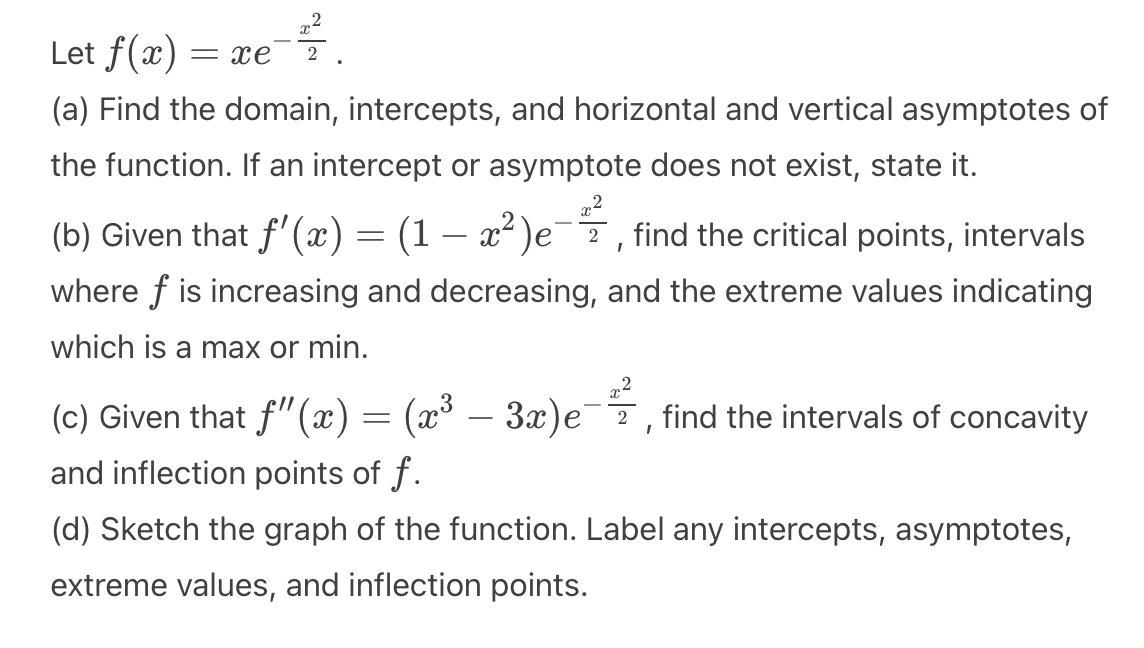
Let f(x)=xex22f(x)=xex22. (a) Find the domain, intercepts, and horizontal and vertical asymptotes of the function. If an intercept or asymptote does not exist, state it.

Let f(x)=xex22f(x)=xex22.
(a) Find the domain, intercepts, and horizontal and vertical asymptotes of the function. If an intercept or asymptote does not exist, state it.
(b) Given that f(x)=(1x2)ex22f(x)=(1x2)ex22, find the critical points, intervals where ff is increasing and decreasing, and the extreme values indicating which is a max or min.
(c) Given that f(x)=(x33x)ex22f(x)=(x33x)ex22, find the intervals of concavity and inflection points of ff.
(d) Sketch the graph of the function. Label any intercepts, asymptotes, extreme values, and inflection points.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


