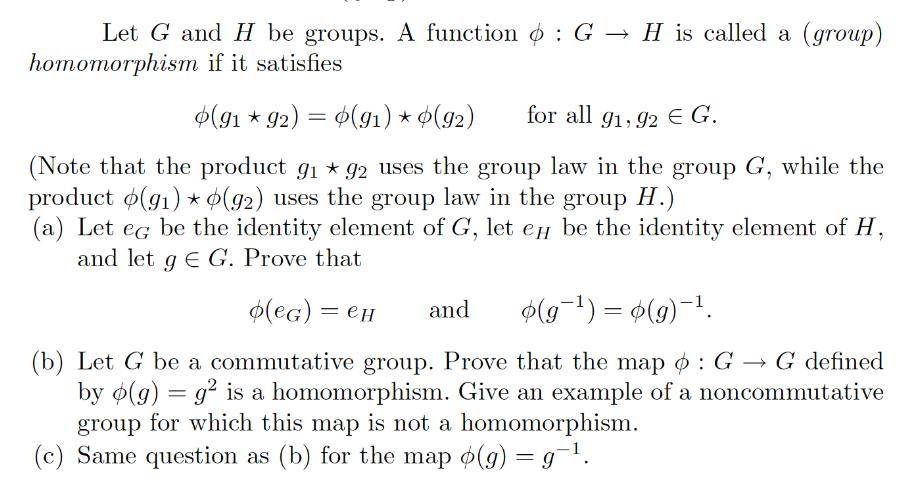
-> Let G and H be groups. A function : G H is called a (group) homomorphism if it satisfies (9192) = (91) * (92) for all 91, 92 G. (Note that the product 91*92 uses the group law in the group G, while the product (91) (92) uses the group law in the group H.) (a) Let eg be the identity element of G, let be the identity element of H, and let g G. Prove that (G) = H and (9)=6(9). (b) Let G be a commutative group. Prove that the map : G G defined = by (g) g is a homomorphism. Give an example of a noncommutative group for which this map is not a homomorphism. (c) Same question as (b) for the map (g) = g.