Let G1 and G2 be two graphs of order n>=2

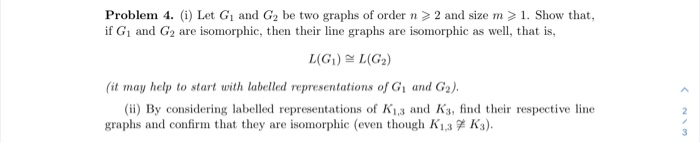
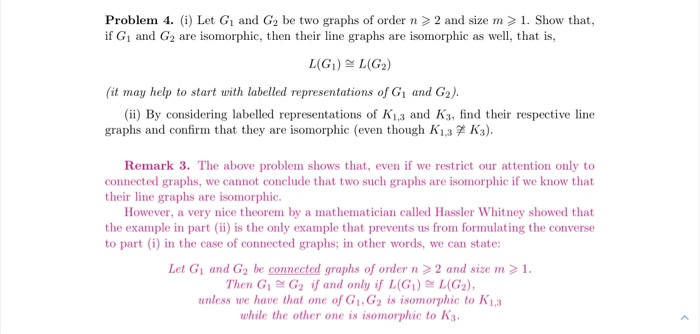
Problem 4. (i) Let G1 and G2 be two graphs of order n > 2 and size m > 1. Show that, if G and G2 are isomorphic, then their line graphs are isomorphic as well, that is, L(G) L(G2) (it may help to start with labelled representations of G1 and G2). (ii) By considering labelled representations of K1,3 and K3, find their respective line graphs and confirm that they are isomorphic (even though K1,3 K3). N> Problem 4. (i) Let G1 and Go be two graphs of order n > 2 and size m > 1. Show that, if G and G2 are isomorphic, then their line graphs are isomorphic as well, that is, L(G) L(G2) (it may help to start with labelled representations of G1 and G2). (ii) By considering labelled representations of K1,3 and K3, find their respective line graphs and confirm that they are isomorphic (even though K1,3 K3). Remark 3. The above problem shows that, even if we restrict our attention only to connected graphs, we cannot conclude that two such graphs are isomorphic if we know that their line graphs are isomorphic. However, a very nice theorem by a mathematician called Hassler Whitney showed that the example in part (ii) is the only example that prevents us from formulating the converse to part (i) in the case of connected graphs; in other words, we can state: Let G and G2 be connected graphs of order n > 2 and size m > 1. Then G2 G2 if and only if L(G) L(G2), unless we have that one of G,G2 is isomorphic to R1,3 while the other one is isomorphic to K3. Problem 4. (i) Let G1 and G2 be two graphs of order n > 2 and size m > 1. Show that, if G and G2 are isomorphic, then their line graphs are isomorphic as well, that is, L(G) L(G2) (it may help to start with labelled representations of G1 and G2). (ii) By considering labelled representations of K1,3 and K3, find their respective line graphs and confirm that they are isomorphic (even though K1,3 K3). N> Problem 4. (i) Let G1 and Go be two graphs of order n > 2 and size m > 1. Show that, if G and G2 are isomorphic, then their line graphs are isomorphic as well, that is, L(G) L(G2) (it may help to start with labelled representations of G1 and G2). (ii) By considering labelled representations of K1,3 and K3, find their respective line graphs and confirm that they are isomorphic (even though K1,3 K3). Remark 3. The above problem shows that, even if we restrict our attention only to connected graphs, we cannot conclude that two such graphs are isomorphic if we know that their line graphs are isomorphic. However, a very nice theorem by a mathematician called Hassler Whitney showed that the example in part (ii) is the only example that prevents us from formulating the converse to part (i) in the case of connected graphs; in other words, we can state: Let G and G2 be connected graphs of order n > 2 and size m > 1. Then G2 G2 if and only if L(G) L(G2), unless we have that one of G,G2 is isomorphic to R1,3 while the other one is isomorphic to K3