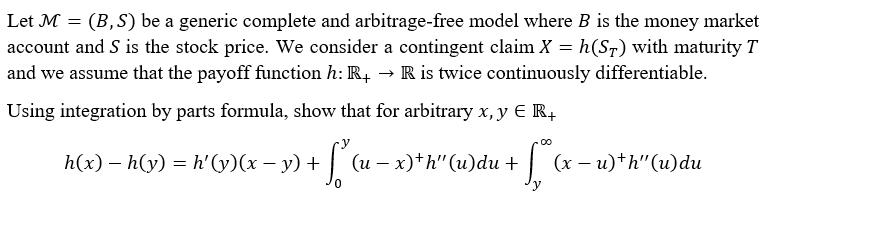
Let M = (B, S) be a generic complete and arbitrage-free model where B is the money market account and S is the stock price. We consider a contingent claim X = h(ST) with maturity T and we assume that the payoff function h: R+ R is twice continuously differentiable. Using integration by parts formula, show that for arbitrary x, y E R+ h(x) h(y) = h'(y)(x y) + f ( - -00 (u-x)+h" (u)du + S (x-u)+h" (u) du