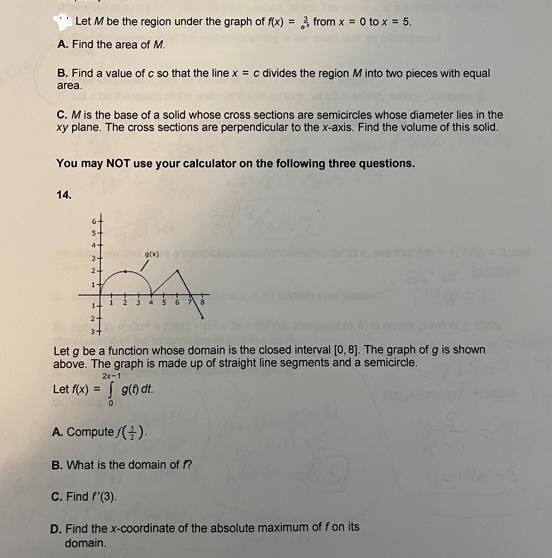
Let M be the region under the graph of f(x) = from x = 0 to x = 5. A. Find the area of M. B. Find a value of c so that the line x = c divides the region M into two pieces with equal area. C. M is the base of a solid whose cross sections are semicircles whose diameter lies in the xy plane. The cross sections are perpendicular to the x-axis. Find the volume of this solid. You may NOT use your calculator on the following three questions. 14. 1- 1. 1 900 Let g be a function whose domain is the closed interval [0, 8]. The graph of g is shown above. The graph is made up of straight line segments and a semicircle. 2x-1 Let f(x) = { A. Compute/(+). A g(t) dt. B. What is the domain off? C. Find f'(3). D. Find the x-coordinate of the absolute maximum of f on its domain.