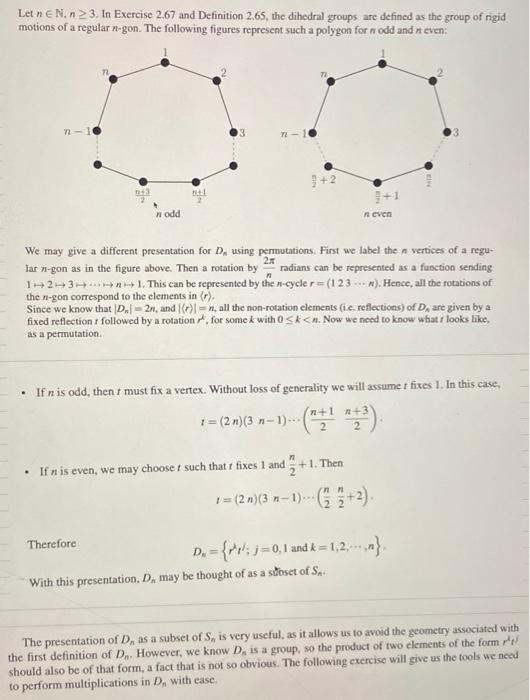
Let n E N. n 23. In Exercise 2.67 and Definition 2.65, the dihedral groups are defined as the group of rigid motions of a regular n-gon. The following figures represent such a polygon form odd and even: n-10 n odd 84 71-10 Therefore i +2 We may give a different presentation for D, using permutations. First we label the vertices of a regu- 2x lar n-gon as in the figure above. Then a rotation by radians can be represented as a function sending 1+2+3+1. This can be represented by the n-cycle r= (1 2 3 ..n). Hence, all the rotations of the n-gon correspond to the elements in (r). n Since we know that ID,|- 2n, and ((r)]=n, all the non-rotation elements (i.c. reflections) of D, are given by a fixed reflection followed by a rotation, for some k with 0 5k Letr and t be as defined above. (a) Describe the product trt geometrically to conclude that tr7 is a rotation. (b) Prove that trt=r. (c) Prove that tr] =rt, for all j=1,2,...,n.