Question: Let P(x | C;) ~ N(;, ) for a two-category, one-dimensional classification problem with classes C1 and C2, P(C) = P(C2) = 1/2, and

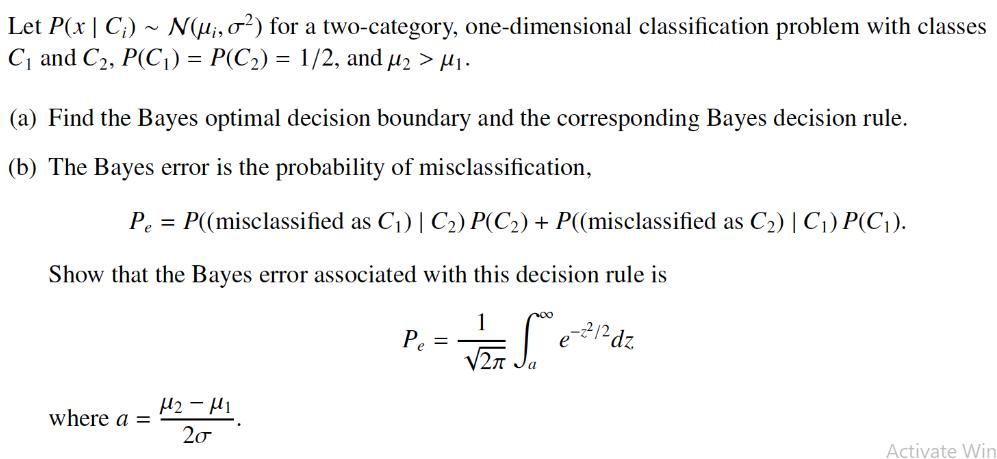
Let P(x | C;) ~ N(;, ) for a two-category, one-dimensional classification

Let P(x | C;) ~ N(;, ) for a two-category, one-dimensional classification problem with classes C1 and C2, P(C) = P(C2) = 1/2, and > . (a) Find the Bayes optimal decision boundary and the corresponding Bayes decision rule. (b) The Bayes error is the probability of misclassification, Pe = P((misclassified as C) | C2) P(C2) + P((misclassified as C2) | C) P(C). Show that the Bayes error associated with this decision rule is where a = M2-M1 20 Pe= e 212 dz Activate Win

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Programming Questions!

Let the random variable X represent the number of defective smartphones in a shipment of 4 smartphones to a local appliance store. Assume that each smartphone is equally likely to be defective or...

Q1. You have identified a market opportunity for home media players that would cater for older members of the population. Many older people have difficulty in understanding the operating principles...

Give the typing rules for Peano natural numbers and their eliminator.(ii) Using the rules given above, define the addition function.] (iii) Let a binary tree be either a leaf Leaf or a node...

9 Consider a continuous-time Markov chain {X(t)} whose jump chain is the success run chain from Problem 16. Give a condition on the holding time parameters ?(k), k = 0, 1, ... guaranteeing that...

1 Gaussan Classification Let P(x | Ci) ~ Ni,2) for a two-category, one-dimensional classification problem with classes C1 and C2, PC) P(C2 1/2, and 2 > (a) Find the Bayes optimal decision boundary...

Consider the symmetric simple random walk at two timepoints: n and n + m. Find ?(Sn, Sn+m). What happens as m ? ? for fixed n and as n ? ? for fixed m? Explain intuitively. 23 Consider the simple...

1 1. (6+4+4+6) Let P3 denote the vector space of all third degree or less polynomials. (a) Find two linearly independent vectors in P3 that interpolate the data points (1, 2), (2, 1) and (3, 2). (b)...

Help me... 1. Answer all questions: (a) ASHEEKA LTD. has annual turnover of exp(-pt)u(c.) 1= 0 subject to the constraints: It+1 = exp(r + out -o'/2)(1 - G), up iid and the ~ N(0, 1), G E [0, If], To...

HHelp. me to solve the following attached questions. 4 Labor market equilibrium with proportional taxation Consider a model in which a household derives utility from consumption c and leisure 1...

QUIZ... Let D be a poset and let f : D D be a monotone function. (i) Give the definition of the least pre-fixed point, fix (f), of f. Show that fix (f) is a fixed point of f. [5 marks] (ii) Show that...

Name four steps in the design and implementation of an ABC system.

If the joint probability density of X and Y is given by Find (a) The marginal density of X; (b) The marginal density of Y. Also determine whether the two random variables are independent. f(x, y)...

1 . Find the Laplace transforms of the following functions. Indicate which theorem on Laplace transforms you used, where applicable. a ) ( ) = 5 5 b ) ( ) = 2 + 2 c ) ( ) = 2 2 2 d ) ( ) = 2 2

For each ratio listed, identify whether the change in ratio value from 2016 to 2017 is usually regarded as favorable or unfavorable. 2017 2016 8% 42% Ratio Ratio 2017 2016 1. Profit margin. 9% 47%...

C.S. Lewis Company had the following transactions involving notes payable. July 1, 2019 Borrows $50,000 from First National Bank by signing a 9-month, 8% note. Nov. 1, 2019 Borrows $60,000 from Lyon...

The Pew Research Center's Social & Demographic Trends project found that 46% of U.S. adults would rather live in a different type of community than the one where they are living now (Pew Research...

The major shopping areas in the community of Springdale include Springdale Mall, West Mall, and the downtown area on Main Street. A telephone survey has been conducted to identify strengths and...

Calculate the internal and sustainable growth rate for Lake of Egypt Marina,Inc. Lake of Egypt Marina, Inc Balance Sheet as of December 31,2015 and 2014 in millions of dollars Assets 2015 2014...

Can we put that she will be making weekly payroll deductions inthe amount of $52.75 to the housing equalling $211.00 monthly

Let p equal the proportion of college students who favor a new policy for alcohol consumption on campus. How large a sample is required to estimate p so that the maximum error of the estimate of p is...

Let F(x) be the cdf of the continuous-type random variable X, and assume that F(x) = 0 for x 0 and 0 x + y | X > x) = P(X > y), Then F(x) = 1 ex, 0

In nuclear physics, detectors are often used to measure the energy of a particle. To calibrate a detector, particles of known energy are directed into it. The values of signals from 15 different...

A $100,000 mortgage at 7.1% compounded semiannually with a 30-year amortization requires monthly payments. How much will the amortization period be shortened if a $10,000 lump payment is made along...

The MacLellans originally chose to make payments of $1600 per month on a $138,000 mortgage written at 7.4% compounded semiannually for the first five years. After three years they exercised their...

The monthly payments on the Wolskis $166,000 mortgage were originally based on a 25-year amortization and an interest rate of 7% compounded semiannually for a five-year term. After two years, they...