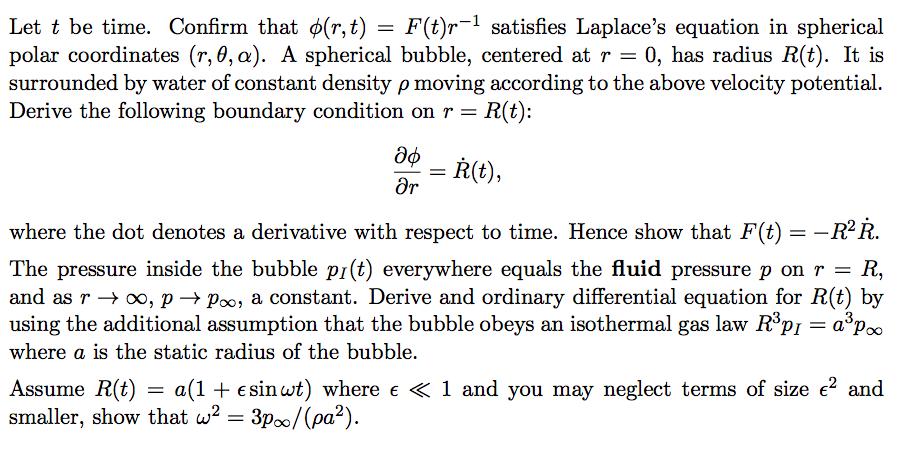
Let t be time. Confirm that o(r,t) = F(t)r-1 satisfies Laplace's equation in spherical polar coordinates (r, 0, a). A spherical bubble, centered at r = 0, has radius R(t). It is surrounded by water of constant density p moving according to the above velocity potential. Derive the following boundary condition on r = R(t): = (t), ar where the dot denotes a derivative with respect to time. Hence show that F(t) = -R R. The pressure inside the bubble p1(t) everywhere equals the fluid pressure p on r = R, and as r 0, p Poo, a constant. Derive and ordinary differential equation for R(t) by using the additional assumption that the bubble obeys an isothermal gas law Rp1 = a p 3. where a is the static radius of the bubble. Assume R(t) smaller, show that w? = 3po0/(pa?). a(1 + esin wt) where e 1 and you may neglect terms of size e? and %3D