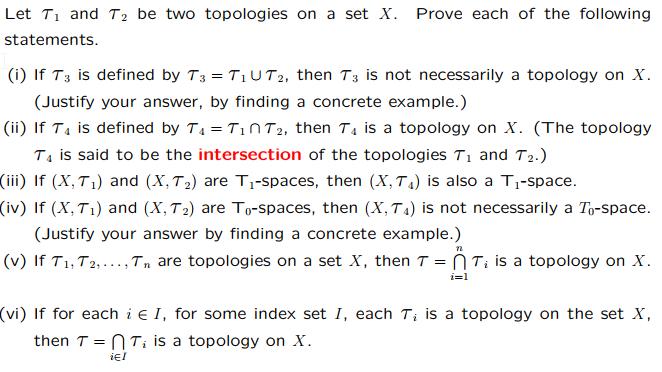
Let Ti and T, be two topologies on a set X. Prove each of the following statements. (i) If T3 is defined by T3 = T1UT2, then T3 is not necessarily a topology on X. (Justify your answer, by finding a concrete example.) (ii) If Ta is defined by T4 = T1n T2, then T4 is a topology on X. (The topology Ta is said to be the intersection of the topologies T1 and T2.) (iii) If (X, T1) and (X, T2) are T,-spaces, then (X,T4) is also a T,-space. (iv) If (X, T1) and (X, T2) are To-spaces, then (X, T4) is not necessarily a To-space. (Justify your answer by finding a concrete example.) (v) If T1, 2,.. ., Tn are topologies on a set X, then T = NT; is a topology on X. i=1 (vi) If for each i e I, for some index set I, each T; is a topology on the set X, then T = N T, is a topology on X. iel