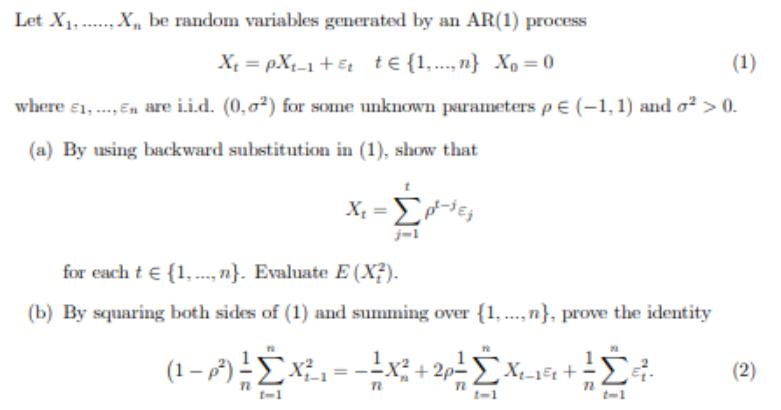
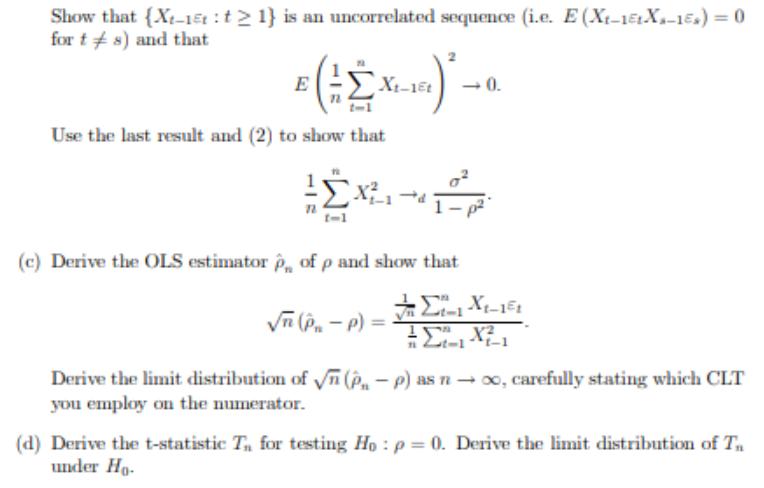
Let X, X, be random variables generated by an AR(1) process X = p X-1 + te {1,..n} Xp=0 (1) where 1,.... En are i.i.d. (0,02) for some unknown parameters p (-1,1) and o2 > 0. (a) By using backward substitutionin (1), show that X=p-1 for each t E (1,..., n}. Evaluate E (X). (b) By squaring both sides of (1) and summing over {1,.... n}, prove the identity (1 P) - X_ = X + 2p XG+ _-1 + 72 72 I-1 WI (2) Show that {X-1t: t > 1} is an uncorrelated sequence (i.e. E(X-11X-15s) = 0 for ts) and that E () = [X-181) Use the last result and (2) to show that X-81 (c) Derive the OLS estimator p of p and show that 0. -1-X-151 n (P-P) = 2-11 :EX Derive the limit distribution of n (pp) as noo, carefully stating which CLT you employ on the numerator. (d) Derive the t-statistic T for testing Ho: p = 0. Derive the limit distribution of T under Ho