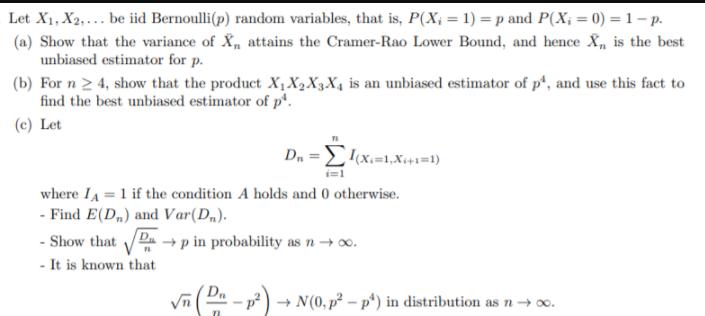
Let X, X2,... be iid Bernoulli(p) random variables, that is, P(X = 1) = p and P(X = 0) = 1 - p. (a) Show that the variance of X, attains the Cramer-Rao Lower Bound, and hence X, is the best unbiased estimator for p. (b) For n 4, show that the product X X X3X is an unbiased estimator of p, and use this fact to find the best unbiased estimator of p. (c) Let D = (x=1, X+1=1) i=1 where IA 1 if the condition A holds and 0 otherwise. - Find E(D) and Var(D). - Show that p in probability as n o. - It is known that n (D N(0, p-p) in distribution as n 0.