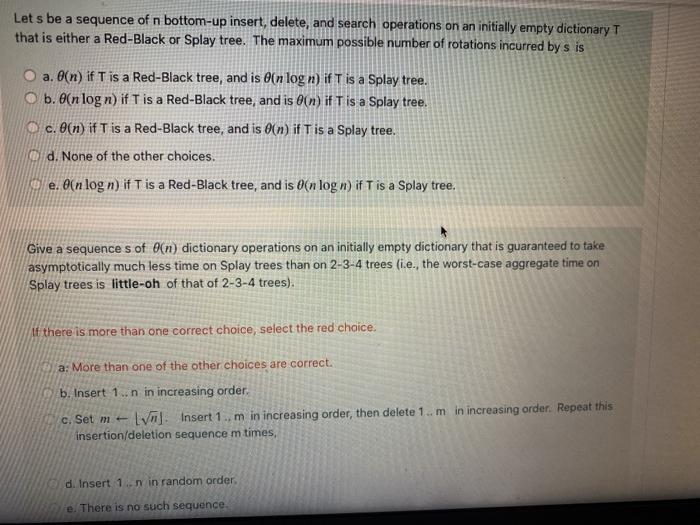
Lets be a sequence of n bottom-up insert, delete, and search operations on an initially empty dictionary T that is either a Red-Black or Splay tree. The maximum possible number of rotations incurred by s is a. O(n) if T is a Red-Black tree, and is in log n) if T is a Splay tree. O b. O(n log n) if T is a Red-Black tree, and is 06n) if T is a Splay tree. c. O(n) if T is a Red-Black tree, and is 0(n) if T is a Splay tree. d. None of the other choices. e. O(n log n) if Tis a Red-Black tree, and is on log n) if Tis a Splay tree. Give a sequence s of O(n) dictionary operations on an initially empty dictionary that is guaranteed to take asymptotically much less time on Splay trees than on 2-3-4 trees (.e., the worst-case aggregate time on Splay trees is little-oh of that of 2-3-4 trees). At there is more than one correct choice, select the red choice. a: More than one of the other choices are correct. b. Insert 1..n in increasing order. c. Set m Lol. Insert 1. m in increasing order, then delete 1. m in increasing order. Repeat this insertion/deletion sequence m times, d. Insert 1. in random order. e. There is no such sequence Lets be a sequence of n bottom-up insert, delete, and search operations on an initially empty dictionary T that is either a Red-Black or Splay tree. The maximum possible number of rotations incurred by s is a. O(n) if T is a Red-Black tree, and is in log n) if T is a Splay tree. O b. O(n log n) if T is a Red-Black tree, and is 06n) if T is a Splay tree. c. O(n) if T is a Red-Black tree, and is 0(n) if T is a Splay tree. d. None of the other choices. e. O(n log n) if Tis a Red-Black tree, and is on log n) if Tis a Splay tree. Give a sequence s of O(n) dictionary operations on an initially empty dictionary that is guaranteed to take asymptotically much less time on Splay trees than on 2-3-4 trees (.e., the worst-case aggregate time on Splay trees is little-oh of that of 2-3-4 trees). At there is more than one correct choice, select the red choice. a: More than one of the other choices are correct. b. Insert 1..n in increasing order. c. Set m Lol. Insert 1. m in increasing order, then delete 1. m in increasing order. Repeat this insertion/deletion sequence m times, d. Insert 1. in random order. e. There is no such sequence