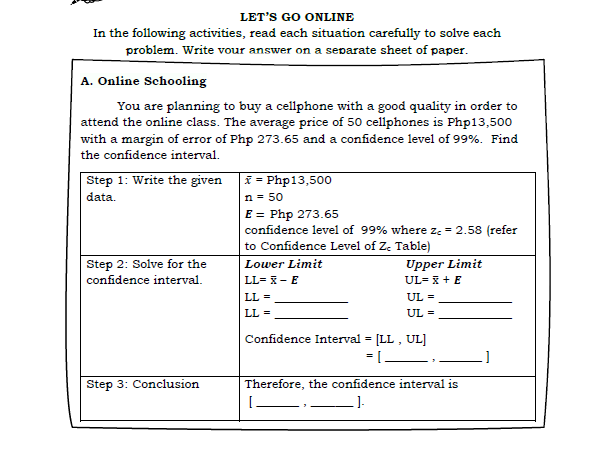
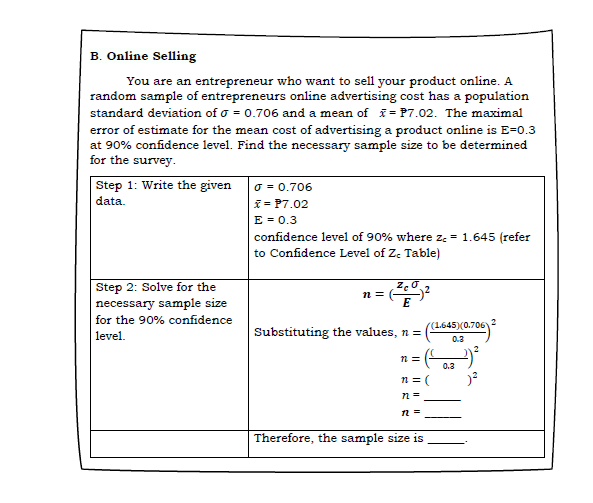
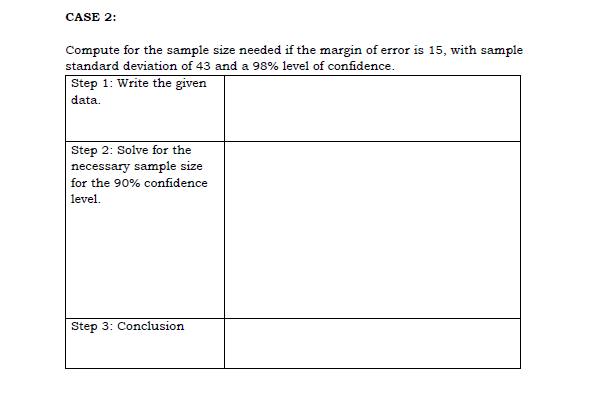
LET'S GO ONLINE In the following activities, read each situation carefully to solve each problem. Write vour answer on a separate sheet of paper. A. Online Schooling You are planning to buy a cellphone with a good quality in order to attend the online class. The average price of 50 cellphones is Php13,500 with a margin of error of Php 273.65 and a confidence level of 99%. Find the confidence interval. Step 1: Write the given $ = Php13,500 data. n = 50 E = Php 273.65 confidence level of 99% where zz = 2.58 (refer to Confidence Level of Z. Table) Step 2: Solve for the Lower Limit Upper Limit confidence interval. LL= X - E UL= X + E LL = UL = LL = UL = Confidence Interval = [LL , UL] = Step 3: Conclusion Therefore, the confidence interval is-B. Online Selling You are an entrepreneur who want to sell your product online. A random sample of entrepreneurs online advertising cost has a population standard deviation of 0 = 0.706 and a mean of # =$7.02. The maximal error of estimate for the mean cost of advertising a product online is E=0.3 at 90% confidence level. Find the necessary sample size to be determined for the survey. Step 1: Write the given T = 0.706 data. F = P7.02 E = 0.3 confidence level of 90% where Z. = 1.645 (refer to Confidence Level of Z. Table) Step 2: Solve for the Zc n = ( necessary sample size for the 90% confidence (1.645)(0.706) 2 level. Substituting the values, n = 0.3 2 1 = 0.2 2 Therefore, the sample size isHAVE CONFIDENCE AND BE THE BEST! Study and analyze each case. Apply the formula necessary to solve the stated problem. Use a separate sheet for your answers. CASE 1: Mr. V wanted to know the average weight (in kilos) of students in LAO University. He took a random sample of 300 students and discovered that their average weight is 55kg with a standard deviation of 0.8kg and the margin of error is 1.32kg. Construct a 95% confidence interval for the mean weight of students in the University. Step 1: Write the given data. Step 2: Solve for the confidence interval. Step 3: ConclusionCASE 2: Compute for the sample size needed if the margin of error is 15, with sample standard deviation of 43 and a 98% level of confidence. Step 1: Write the given data. Step 2: Solve for the necessary sample size for the 90% confidence level. Step 3: Conclusion