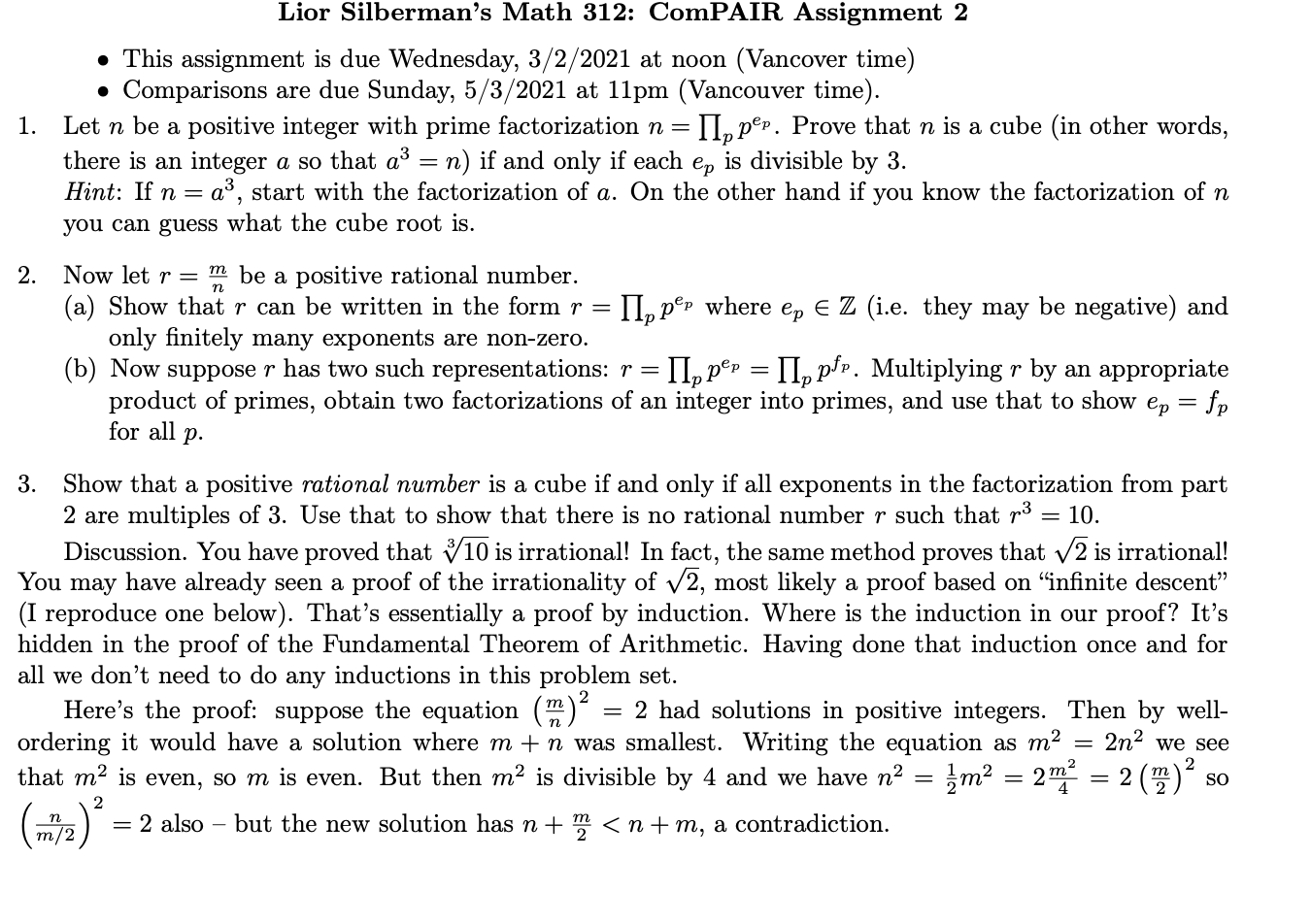
Lior Silberman's Math 312: ComPAIR Assignment 2 This assignment is due Wednesday, 3/2/2021 at noon (Vancover time) Comparisons are due Sunday, 5/3/2021 at 11pm (Vancouver time). 1. Let n be a positive integer with prime factorization n = pp. Prove that n is a cube (in other words, there is an integer a so that a = n) if and only if each ep is divisible by 3. Hint: If n = a, start with the factorization of a. On the other hand if you know the factorization of n you can guess what the cube root is. 2. Now let r = m be a positive rational number. (a) Show that r can be written in the form r = p where p Z (i.e. they may be negative) and only finitely many exponents are non-zero. (b) Now suppose r has two such representations: r = Ipp = I pp. Multiplying r by an appropriate product of primes, obtain two factorizations of an integer into primes, and use that to show = fp for all p. 3. Show that a positive rational number is a cube if and only if all exponents in the factorization from part 2 are multiples of 3. Use that to show that there is no rational number r such that r = 10. Discussion. You have proved that 10 is irrational! In fact, the same method proves that 2 is irrational! You may have already seen a proof of the irrationality of 2, most likely a proof based on "infinite descent" (I reproduce one below). That's essentially a proof by induction. Where is the induction in our proof? It's hidden in the proof of the Fundamental Theorem of Arithmetic. Having done that induction once and for all we don't need to do any inductions in this problem set. Here's the proof: suppose the equation (m) 2 had solutions in positive integers. Then by well- ordering it would have a solution where m + n was smallest. Writing the equation as m 2n we see that m is even, so m is even. But then m is divisible by 4 and we have n : m = 2m = 2 (m) s 2 = = SO = 2 also but the new solution has n + m