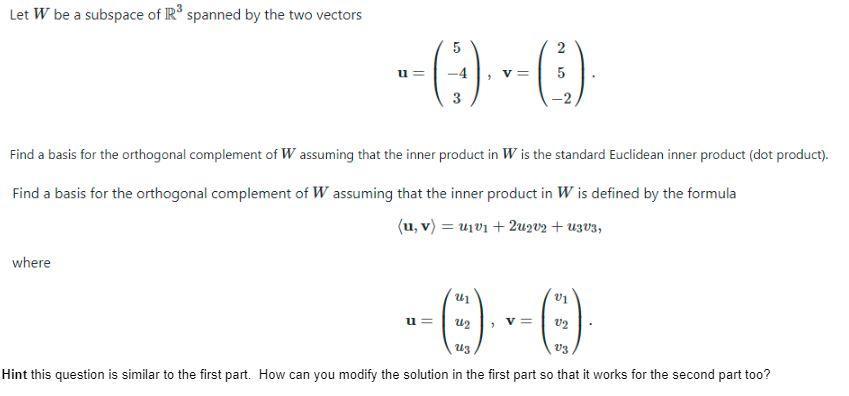
Let W be a subspace of R spanned by the two vectors 5 -0-0 where 2 5 Find a basis for the orthogonal complement of W assuming that the inner product in W is the standard Euclidean inner product (dot product). Find a basis for the orthogonal complement of W assuming that the inner product in W is defined by the formula (u, v) = 1v1 +2u2v2 + U3v3, 241 u= 22 -() Uz Hint this question is similar to the first part. How can you modify the solution in the first part so that it works for the second part too?