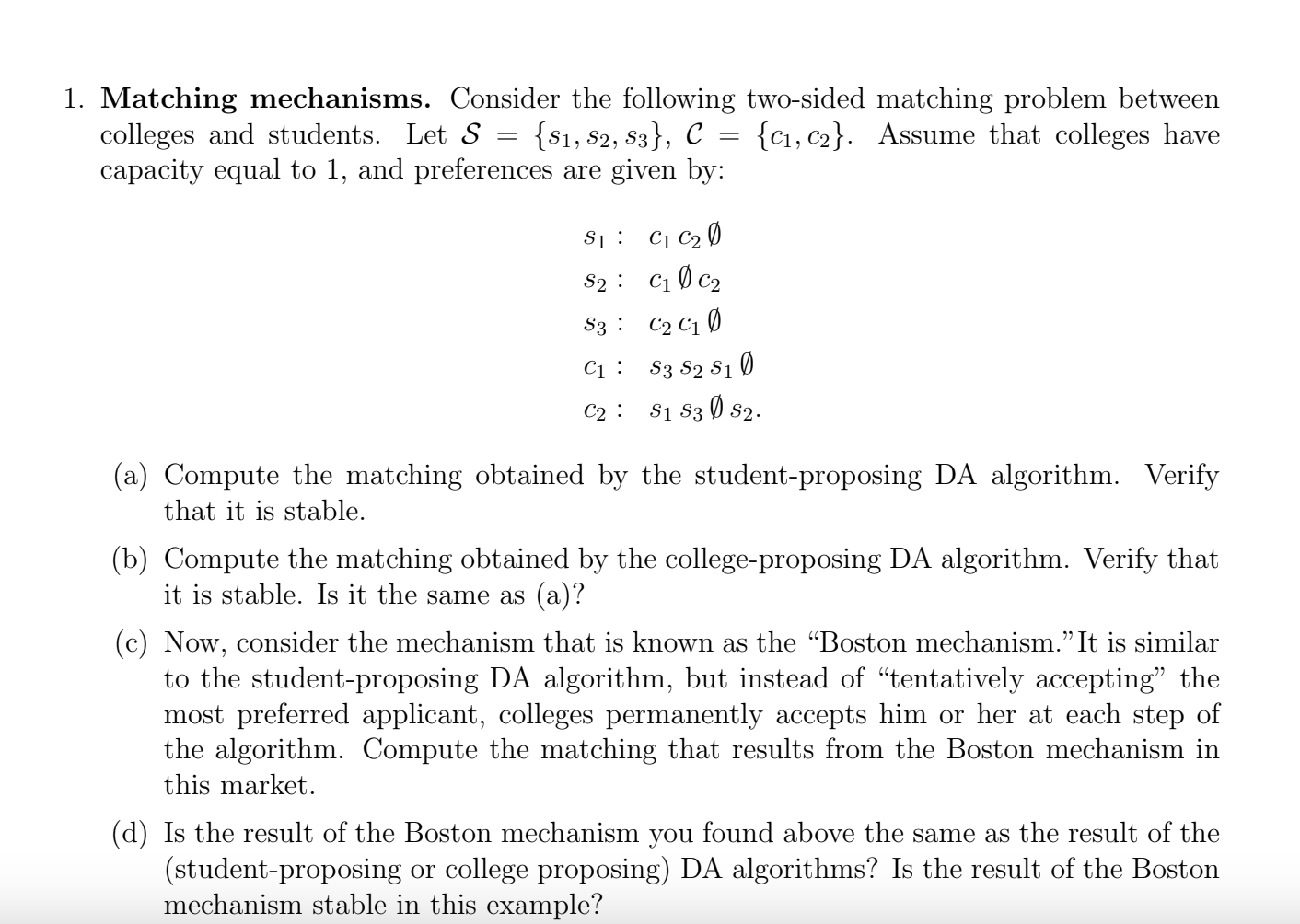Matching mechanisms. Consider the following two-sided matching problem between colleges and students. Let S={s1,s2,s3},C={c1,c2}. Assume that colleges have capacity equal to 1 , and preferences are given by: s1:s2:s3:c1:c2:c1c2c1c2c2c1s3s2s1s1s3s2. (a) Compute the matching obtained by the student-proposing DA algorithm. Verify that it is stable. (b) Compute the matching obtained by the college-proposing DA algorithm. Verify that it is stable. Is it the same as (a)? (c) Now, consider the mechanism that is known as the "Boston mechanism." It is similar to the student-proposing DA algorithm, but instead of "tentatively accepting" the most preferred applicant, colleges permanently accepts him or her at each step of the algorithm. Compute the matching that results from the Boston mechanism in this market. (d) Is the result of the Boston mechanism you found above the same as the result of the (student-proposing or college proposing) DA algorithms? Is the result of the Boston mechanism stable in this example