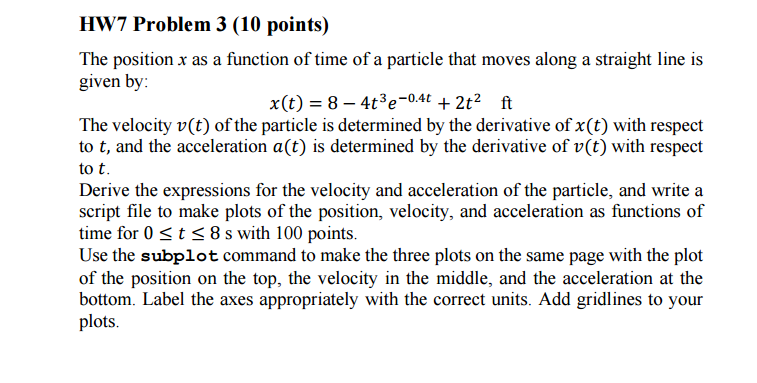
MATLAB Code Please!

The position x as a function of time of a particle that moves along a straight line is given by: x(t) = 8 - 4t^3e^-0.;4t + 2t^2 ft The velocity v(t) of the particle is determined by the derivative of x(t) with respect to t, and the acceleration a(t) is determined by the derivative of v(t) with respect to t. Derive the expressions for the velocity and acceleration of the particle, and write a script file to make plots of the position, velocity, and acceleration as functions of time for 0 lessthanorequalto t lessthanorequalto 8 s with 100 points. Use the subplot command to make the three plots on the same page with the plot of the position on the top, the velocity in the middle, and the acceleration at the bottom. Label the axes appropriately with the correct units. Add gridlines to your plots