MATLAB

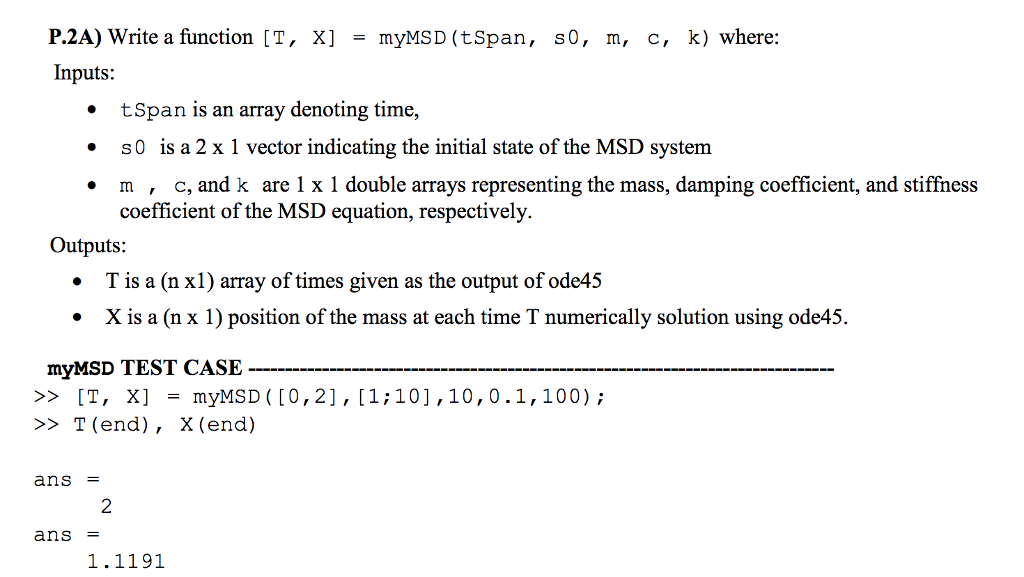
Please do not use any tools not included in the basic student MATLAB package.

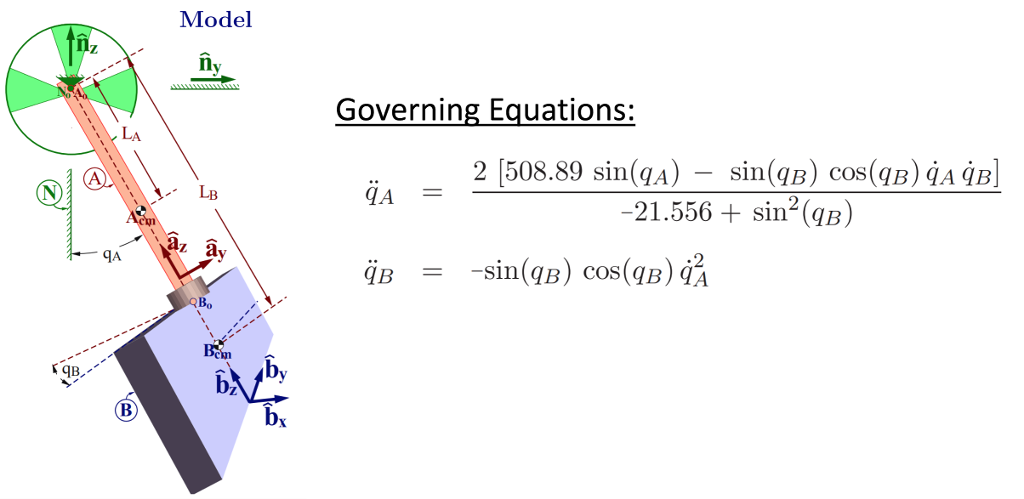
I believe this is the lecture slide that should be refered to.

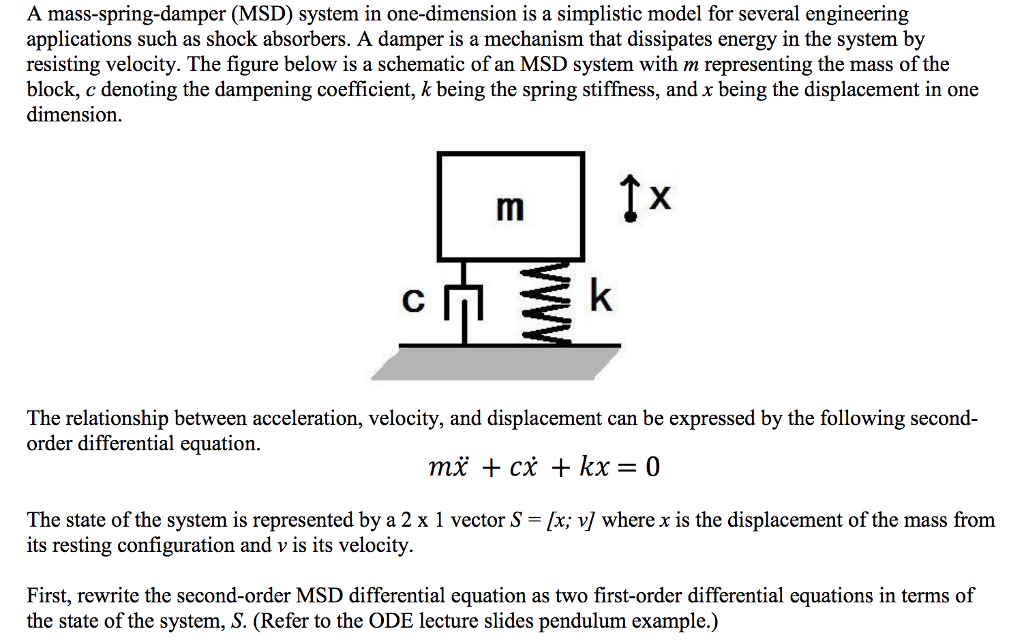
A mass-spring-damper (MSD) system in one-dimension is a simplistic model for several engineering applications such as shock absorbers. A damper is a mechanism that dissipates energy in the system by resisting velocity. The figure below is a schematic of an MSD system with m representing the mass of the block, c denoting the dampening coefficient, k being the spring stiffness, and x being the displacement in one dimensiorn The relationship between acceleration, velocity, and displacement can be expressed by the following second- order differential equation The state of the system is represented by a 2 x 1 vector S-[x; v/ where x is the displacement of the mass from its resting configuration and v is its velocity First, rewrite the second-order MSD differential equation as two first-order differential equations in terms of the state of the system, S. (Refer to the ODE lecture slides pendulum example.) A mass-spring-damper (MSD) system in one-dimension is a simplistic model for several engineering applications such as shock absorbers. A damper is a mechanism that dissipates energy in the system by resisting velocity. The figure below is a schematic of an MSD system with m representing the mass of the block, c denoting the dampening coefficient, k being the spring stiffness, and x being the displacement in one dimensiorn The relationship between acceleration, velocity, and displacement can be expressed by the following second- order differential equation The state of the system is represented by a 2 x 1 vector S-[x; v/ where x is the displacement of the mass from its resting configuration and v is its velocity First, rewrite the second-order MSD differential equation as two first-order differential equations in terms of the state of the system, S. (Refer to the ODE lecture slides pendulum example.)