Answered step by step
Verified Expert Solution
Question
1 Approved Answer
MATLAB PLS The evolution of the Sierpinski triangle Introduction The Sierpinski triangle can be implemented in MATLAB by plotting points iteratively according to one of
MATLAB PLS
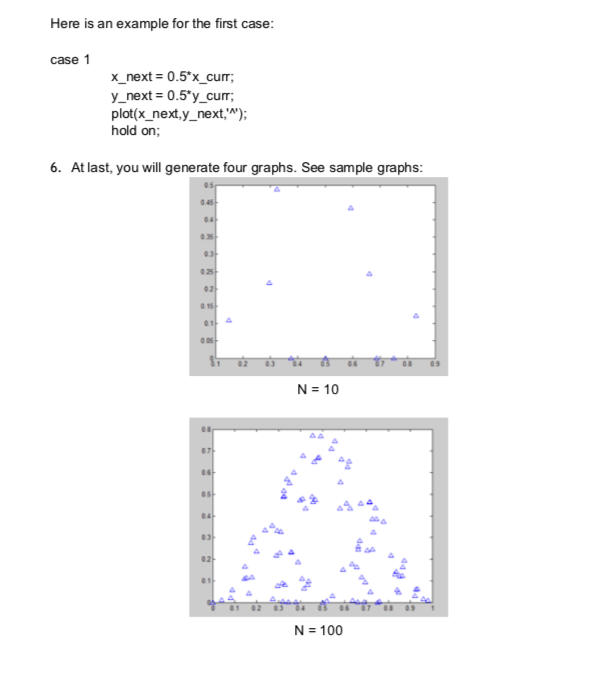
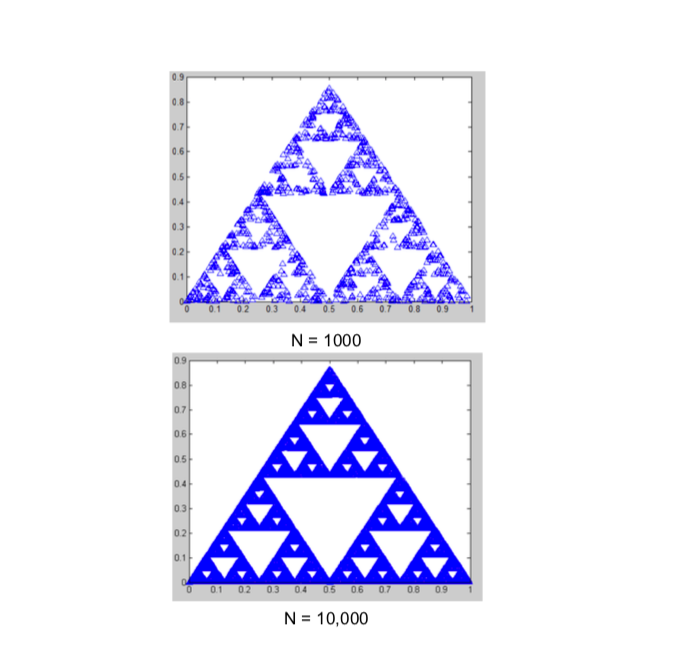
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


