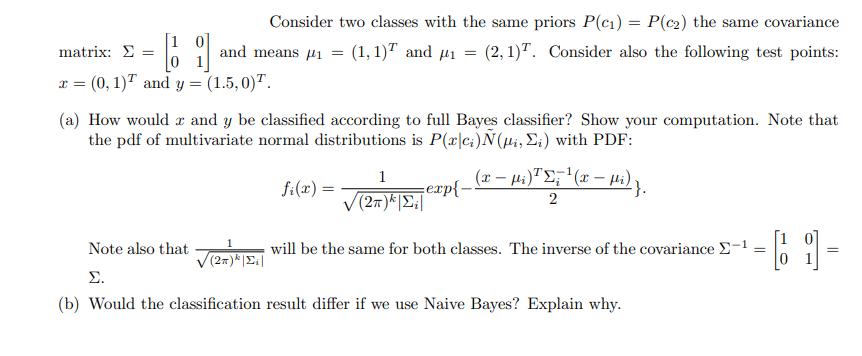
matrix: = = Consider two classes with the same priors P(c) = P(c2) the same covariance and means = (1,1) and (2, 1). Consider also the following test points: x=(0, 1) and y = (1.5,0). (a) How would x and y be classified according to full Bayes classifier? Show your computation. Note that the pdf of multivariate normal distributions is P(x|c)N(pi, Ei) with PDF: 1 f(x) = (2) exp{- (2)* - - (x i) (x Hi)}. 2 Note also that (2) will be the same for both classes. The inverse of the covariance -1. . (b) Would the classification result differ if we use Naive Bayes? Explain why. === =