MCQ: A) Here is a finite automaton:

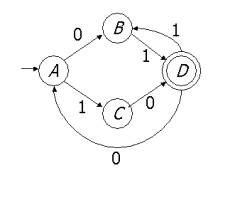
Which of the following regular expressions defines the same language as the finite automaton? Hint: each of the correct choices uses component expressions. Some of these components are:

1. The ways to get from A to D without going through D.

2. The ways to get from D to itself, without going through D.

3. The ways to get from A to itself, without going through A.

a) (01+10)(11+0(01+10))*

b) ((01+10)(11)*0)*(01+10)

c) (01+10)(11*+0(01+10))*

d) (01+10)(0(01+10))*(11)*

--

B) Here is an epsilon-NFA:

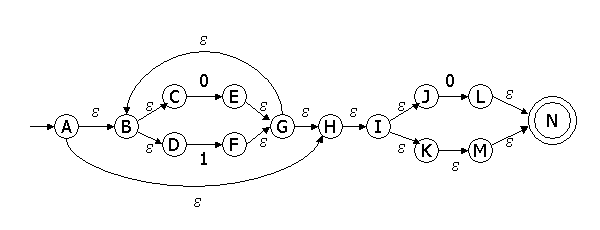
Suppose we construct an equivalent DFA by the construction of Section 2.5.5 (p. 77). That is, start with the epsilon-closure of the start state A. For each set of states S we construct (which becomes one state of the DFA), look at the transitions from this set of states on input symbol 0. See where those transitions lead, and take the union of the epsilon-closures of all the states reached on 0. This set of states becomes a state of the DFA. Do the same for the transitions out of S on input 1. When we have found all the sets of epsilon-NFA states that are constructed in this way, we have the DFA and its transitions.

Carry out this construction of a DFA, and identify one of the states of this DFA (as a subset of the epsilon-NFA's states) from the list below.

a) IJKMN

b) BCDEGHIJKMN

c) BCDEGHIJKLMN

d) BCDFGHIJK

1 0 1 c) 0 1 0 A