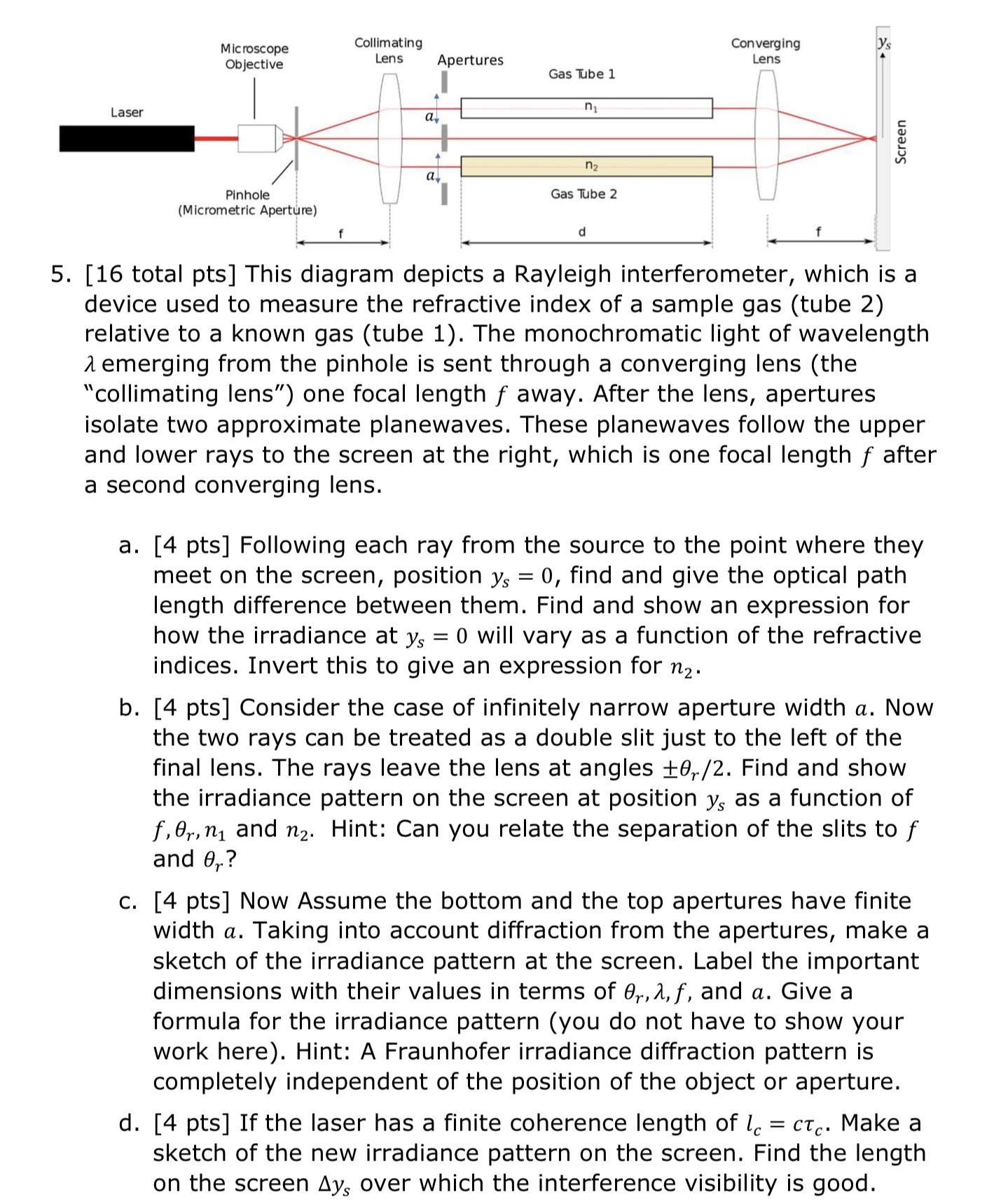
Microscope Colllmatlng Converging 3:,- Objective Lens APEFIUFES Lens . Gas 'Dbe 1 .' \"1 Laser a. . C . ! 'U i / a. Pinhole Gas Tube 2 (M icrometric Aperture) f d f 5. [16 total pts] This diagram depicts a Rayleigh interferometer, which is a device used to measure the refractive index of a sample gas (tube 2) relative to a known gas (tube 1). The monochromatic light of wavelength Aemerging from the pinhole is sent through a converging lens (the \"collimating lens") one focal length f away. After the lens, apertures isolate two approximate planewaves. These planewaves follow the upper and lower rays to the screen at the right, which is one focal length f after a second converging lens. a. [4 pts] Following each ray from the source to the point where they meet on the screen, position y, = 0, find and give the optical path length difference between them. Find and show an expression for how the irradiance at y, = 0 will vary as a function of the refractive indices. Invert this to give an expression for 712. b. [4 pts] Consider the case of infinitely narrow aperture width a. Now the two rays can be treated as a double slit just to the left of the final lens. The rays leave the lens at angles -_l6,/2. Find and show the irradiance pattern on the screen at position ys as a function of f,6,,n1 and n2. Hint: Can you relate the separation of the slits to f and 6,? c. [4 pts] Now Assume the bottom and the top apertures have finite width a. Taking into account diffraction from the apertures, make a sketch of the irradiance pattern at the screen. Label the important dimensions with their values in terms of 9,,/1,f, and a. Give a formula for the irradiance pattern (you do not have to show your work here). Hint: A Fraunhofer irradiance diffraction pattern is completely independent of the position of the object or aperture. d. [4 pts] If the laser has a finite coherence length of l, : etc. Make a sketch of the new irradiance pattern on the screen. Find the length on the screen Ays over which the interference visibility is good