
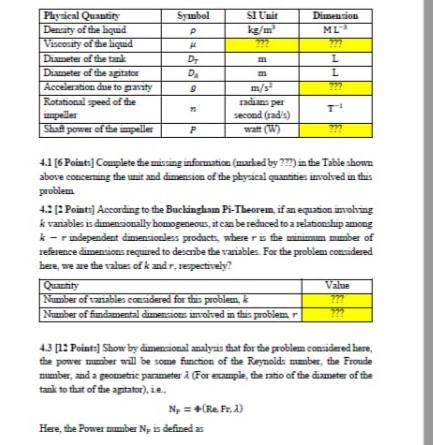
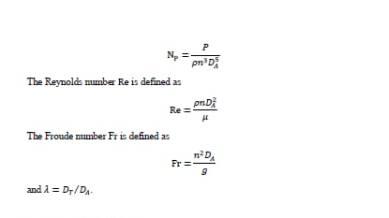
Mixing is a common operation in the chemical process industry. A sketch of a generic mixer used for handling low to medium viscosty fluids is given below. Shaft Liquid Baffle Level Dr Blade HH ID Figure 4. System Sketch of a generic mizer used for handling low to medium viscosity fluids Typically, a mixer is a hollow cylinder filled with the substances to be mixed to some appropriate level. An agitator consisting of a shaft connected to a motor at one end and a set of blades at the other is mounted as shown. In the absence of baffles on the wall, a simple rotating flow, sometimes called a vortex flow, can result that would not cause mixing. In addition, such a simple flow would cause a dip in the surface, and this can exacerbate the entrainment of air into the liquid. Therefore, miters typically have baffles that are attached to the wall to break up this vortex flow. Here, we focus on the application of dimensional analysis to find the important dimensionless groups. A limited list of the important physical quantities is given here. Several other peometric parameters are omitted. Symbol SI Unit Dimension Physical Quantity Density of the liquid Viscosity of the liquid P kg/m MLA H Diameter of the tank Dy m L Diameter of the agitator D m L Acceleration due to gravity 9 m/s radians per Rotational speed of the impeller 721 T second (rad/s) Shaft power of the impeller P watt (W) ??? 4.1 [6 Points] Complete the missing information (marked by ???) in the Table shown above concerning the unit and dimension of the physical quantities involved in this problem 4.2 [2 Points] According to the Buckingham Pi-Theorem, if an equation involving k vanables is dimensionally homogeneous, it can be reduced to a relationship among k - r independent dimensionless products, where r is the minimum number of reference dimensions required to describe the variables. For the problem considered here, we are the values of k and r, respectively? Quantity Value Number of variables considered for this problem, k Number of fundamental dimensions involved in this problem, r 4.3 [12 Point:] Show by dimensional analysis that for the problem considered here, the power number will be some function of the Reynold: mamber, the Froude number, and a geometric parameter & (For example, the ratio of the diameter of the tank to that of the agitator), ie.. Ny = + (Re. Fr. 1) Here, the Power number Ny is defined as N The Reynolds number Re is defined as Re The Froude number Fr is defined as and = DT/DA- P pnD prD H nD 9 Fr=









