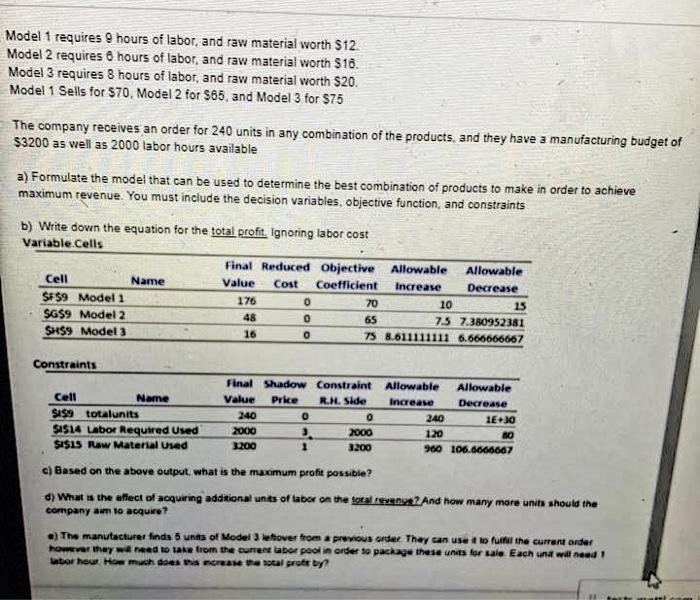
Model 1 requires 9 hours of labor, and raw material worth $12 Model 2 requires 8 hours of labor and raw material worth $16. Model 3 requires 8 hours of labor, and raw material worth $20. Model 1 Sells for $70. Model 2 for $65, and Model 3 for $75 The company receives an order for 240 units in any combination of the products, and they have a manufacturing budget of $3200 as well as 2000 labor hours available a) Formulate the model that can be used to determine the best combination of products to make in order to achieve maximum revenue. You must include the decision variables, objective function and constraints b) Write down the equation for the total profit. Ignoring labor cost Variable.Cells Final Reduced Objective Allowable Allowable Cell Name Value Cost Coefficient Increase Decrease $F$9 Model 1 176 0 10 15 SG$9 Model 2 48 65 7.5 7.380952381 SH$9 Model 3 16 0 75 8.611111111 6.666666667 70 0 Constraints Final Shadow Constraint Allowable Allowable Cell Name Value Price R.H. Side Increase Decrease Sisg totalunits 240 0 0 240 1E.30 S1514 Labor Required Used 2000 3 2000 120 60 $1$15 Raw Material Used 2200 1 3200 960 106.6666667 c) Based on the above output what is the maximum profit possible? a) What is the effect of acquiring additional unts of labor on the total revenue? And how many more units should the company awm to acquire? .) The manutacturer finds 5 unts of Model 3 leftover from a previous order. They can use it to the current order however they will need to take from the current labor pool in order to package these units for sale. Each und will need! bor hour How much does this ncrease the focal pretty Model 1 requires 9 hours of labor, and raw material worth $12 Model 2 requires 8 hours of labor and raw material worth $16. Model 3 requires 8 hours of labor, and raw material worth $20. Model 1 Sells for $70. Model 2 for $65, and Model 3 for $75 The company receives an order for 240 units in any combination of the products, and they have a manufacturing budget of $3200 as well as 2000 labor hours available a) Formulate the model that can be used to determine the best combination of products to make in order to achieve maximum revenue. You must include the decision variables, objective function and constraints b) Write down the equation for the total profit. Ignoring labor cost Variable.Cells Final Reduced Objective Allowable Allowable Cell Name Value Cost Coefficient Increase Decrease $F$9 Model 1 176 0 10 15 SG$9 Model 2 48 65 7.5 7.380952381 SH$9 Model 3 16 0 75 8.611111111 6.666666667 70 0 Constraints Final Shadow Constraint Allowable Allowable Cell Name Value Price R.H. Side Increase Decrease Sisg totalunits 240 0 0 240 1E.30 S1514 Labor Required Used 2000 3 2000 120 60 $1$15 Raw Material Used 2200 1 3200 960 106.6666667 c) Based on the above output what is the maximum profit possible? a) What is the effect of acquiring additional unts of labor on the total revenue? And how many more units should the company awm to acquire? .) The manutacturer finds 5 unts of Model 3 leftover from a previous order. They can use it to the current order however they will need to take from the current labor pool in order to package these units for sale. Each und will need! bor hour How much does this ncrease the focal pretty