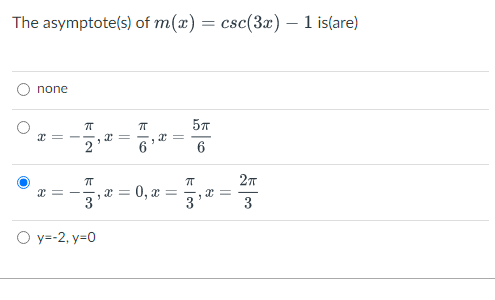
m(x) = csc(3x) - 1 is increasing on (, ) ...()u(0)(.) U... ...U (3,0) U(0, 1) U ( 3 O...U()()()u... U ... () (0) (,)..... U 3 none The amplitude of m(x) = csc(3x) - 1 is -1 2T 3 none 03 m(x) = csc(3x) - 1 is decreasing on U ... () () ().... none 3 U ...u (0) (0) ()u... U U 6 3 ...u ()u(a)) ... 3 m(x) = csc(3x) - 1 has local maximum(s) at none 7 ...(.). (..). (..).... (-)(2)(-2).... m(x) = csc(3x) - 1 has local minimum(s) at none (0) (0) (0).... (-2) (2) (-2).... (0) (0).(). The asymptote(s) of m(x) = = csc(3x) - 1 is(are) none T T 5 x = -- x = x = 2 6 6 T T 2T x = x = = 0, x = x = 3 3 y=-2, y=0 The domain of m(x) = csc(3x) - 1 is (-00,00) or R (-,-2] U [0,0) ...u (0) (0) () ... U 3' 3 none of these The range of m(x) = csc(3x) - 1 is (-0,-2] U [0,) none of these O(-0,-2)) U (0,00) (-00,00) or R The left-hand end behavior of m(x) = csc(3x) - 1 is Oas oo, m (x) 0 as oo, m (x) none as a , m (x) - The right-hand behavior of m(x) = csc(3x) - 1 is as ao, m (x) as ao, m (x) 0 none as a, m (x)