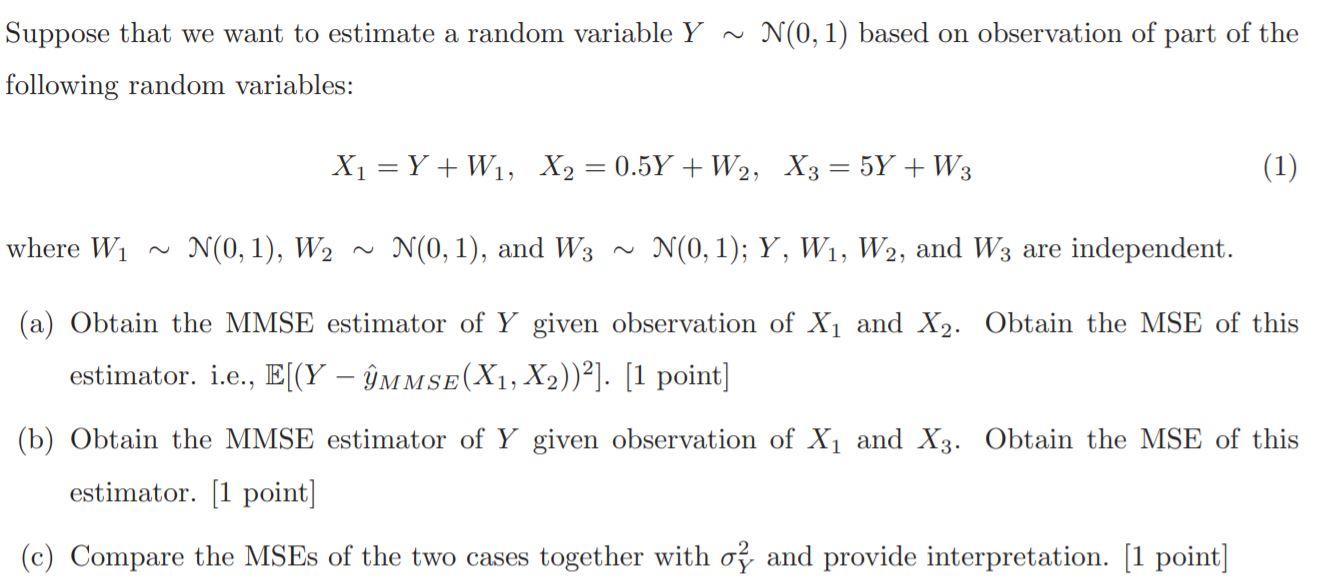
Suppose that we want to estimate a random variable Y N(0, 1) based on observation of part of the following random variables: X1 = Y + W1, X2 = 0.5Y + W2, X3 = 5Y + W3 (1) where W1 N(0, 1), W2 N(0, 1), and W3 N(0, 1); Y, W1, W2, and W3 are independent. (a) Obtain the MMSE estimator of Y given observation of X1 and X2. Obtain the MSE of this estimator. i.e., E[(Y MMSE(X1, X2))]. [1 point] (b) Obtain the MMSE estimator of Y given observation of X1 and X3. Obtain the MSE of this estimator. [1 point] (c) Compare the MSES of the two cases together with o and provide interpretation. [1 point]