Need answer to 1d
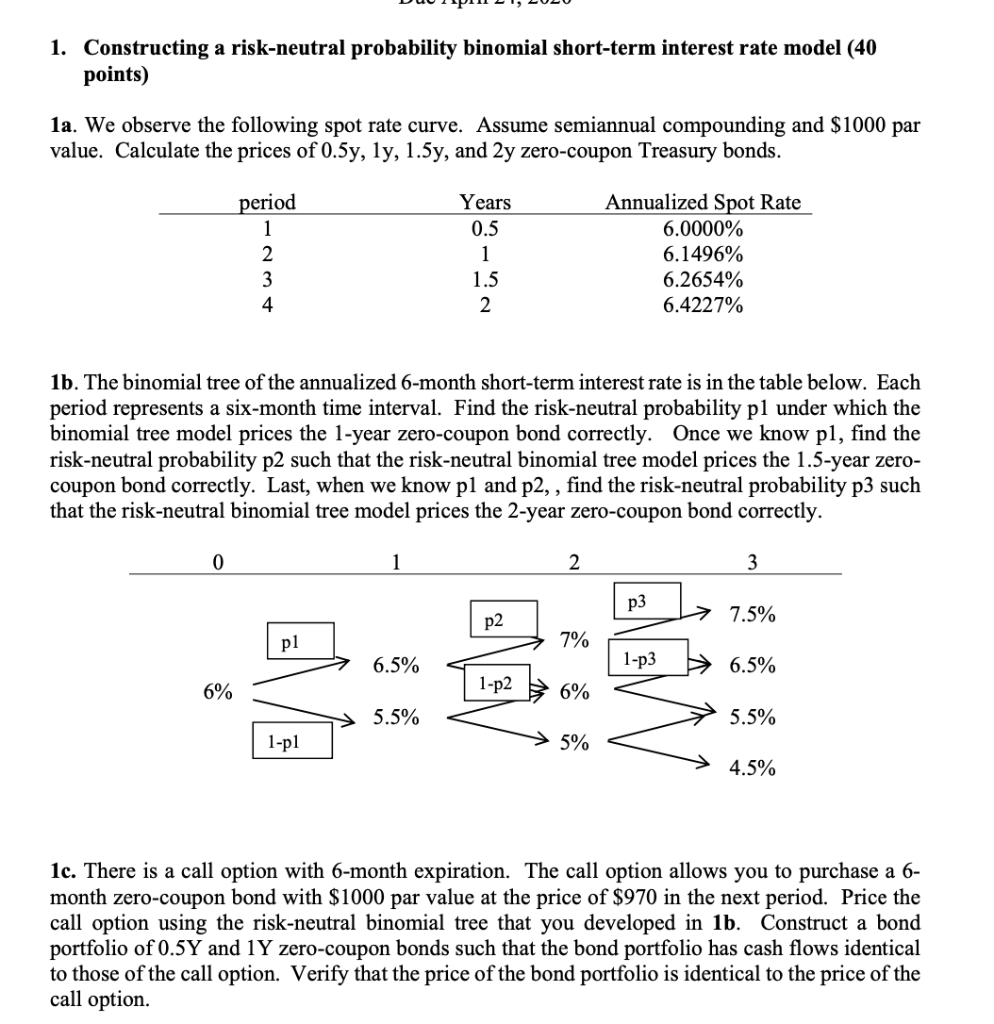


DUI PIII, LULU 1. Constructing a risk-neutral probability binomial short-term interest rate model (40 points) 1a. We observe the following spot rate curve. Assume semiannual compounding and $1000 par value. Calculate the prices of 0.5y, ly, 1.5y, and 2y zero-coupon Treasury bonds. period Years 0.5 Annualized Spot Rate 6.0000% 6.1496% 6.2654% 6.4227% 1.5 1b. The binomial tree of the annualized 6-month short-term interest rate is in the table below. Each period represents a six-month time interval. Find the risk-neutral probability pl under which the binomial tree model prices the 1-year zero-coupon bond correctly. Once we know pl, find the risk-neutral probability p2 such that the risk-neutral binomial tree model prices the 1.5-year zero- coupon bond correctly. Last, when we know pl and p2, , find the risk-neutral probability p3 such that the risk-neutral binomial tree model prices the 2-year zero-coupon bond correctly. 17 7.5% p2 pl 6.5% 1-p3 6.5% 6% 1-p2 > 5.5% 5% = 5.5% 1-pl > - > 4.5% 1c. There is a call option with 6-month expiration. The call option allows you to purchase a 6- month zero-coupon bond with $1000 par value at the price of $970 in the next period. Price the call option using the risk-neutral binomial tree that you developed in 1b. Construct a bond portfolio of 0.54 and 1Y zero-coupon bonds such that the bond portfolio has cash flows identical to those of the call option. Verify that the price of the bond portfolio is identical to the price of the call option. 1d. There is a 2-year 8% coupon bond with the par value of $1000. Price the bond using (1) the spot rate curve provided in la and the risk-neutral binomial tree developed in lb. Verify that prices obtained from the two methods are identical. 1A.) Price of 0.5y zero coupon bond = $1000/(1+0.06/2) = $970.8738 Price of 1y zero coupon bond = $1000/(1+0.061496/2)^2 = $941.2283 Price of 1.5y zero coupon bond = $1000/(1+0.062654/2)^3 = $911.6137 Price of 2y zero coupon bond = $1000/(1+0.064227/2)^4 = $881.2318 1B.) p = (ert -d)/(u - d) Period 1 u = 1.083334 d = 0.916667 Rp = 0.06 P1 = ((0.06*0.5) - 0.91667)/(1.083334 -0.916667) =0.68272 Probability doesn't change so P1 = P2 = P3 1C.) Fu = St - X = 1030 - 1000 = 30 Fd = 0 Call price: f = e-{ piFu + (1-21)Fd} = -0.06*0.5(0.68272*30 + (1-0.68272)*0) = 19.8762 DUI PIII, LULU 1. Constructing a risk-neutral probability binomial short-term interest rate model (40 points) 1a. We observe the following spot rate curve. Assume semiannual compounding and $1000 par value. Calculate the prices of 0.5y, ly, 1.5y, and 2y zero-coupon Treasury bonds. period Years 0.5 Annualized Spot Rate 6.0000% 6.1496% 6.2654% 6.4227% 1.5 1b. The binomial tree of the annualized 6-month short-term interest rate is in the table below. Each period represents a six-month time interval. Find the risk-neutral probability pl under which the binomial tree model prices the 1-year zero-coupon bond correctly. Once we know pl, find the risk-neutral probability p2 such that the risk-neutral binomial tree model prices the 1.5-year zero- coupon bond correctly. Last, when we know pl and p2, , find the risk-neutral probability p3 such that the risk-neutral binomial tree model prices the 2-year zero-coupon bond correctly. 17 7.5% p2 pl 6.5% 1-p3 6.5% 6% 1-p2 > 5.5% 5% = 5.5% 1-pl > - > 4.5% 1c. There is a call option with 6-month expiration. The call option allows you to purchase a 6- month zero-coupon bond with $1000 par value at the price of $970 in the next period. Price the call option using the risk-neutral binomial tree that you developed in 1b. Construct a bond portfolio of 0.54 and 1Y zero-coupon bonds such that the bond portfolio has cash flows identical to those of the call option. Verify that the price of the bond portfolio is identical to the price of the call option. 1d. There is a 2-year 8% coupon bond with the par value of $1000. Price the bond using (1) the spot rate curve provided in la and the risk-neutral binomial tree developed in lb. Verify that prices obtained from the two methods are identical. 1A.) Price of 0.5y zero coupon bond = $1000/(1+0.06/2) = $970.8738 Price of 1y zero coupon bond = $1000/(1+0.061496/2)^2 = $941.2283 Price of 1.5y zero coupon bond = $1000/(1+0.062654/2)^3 = $911.6137 Price of 2y zero coupon bond = $1000/(1+0.064227/2)^4 = $881.2318 1B.) p = (ert -d)/(u - d) Period 1 u = 1.083334 d = 0.916667 Rp = 0.06 P1 = ((0.06*0.5) - 0.91667)/(1.083334 -0.916667) =0.68272 Probability doesn't change so P1 = P2 = P3 1C.) Fu = St - X = 1030 - 1000 = 30 Fd = 0 Call price: f = e-{ piFu + (1-21)Fd} = -0.06*0.5(0.68272*30 + (1-0.68272)*0) = 19.8762









