need help answering this question asap ! plese help me get the answer and to understand it ! i'd appreciate it if steps were shown please. i provided some additional information on how to get some values. Please help !
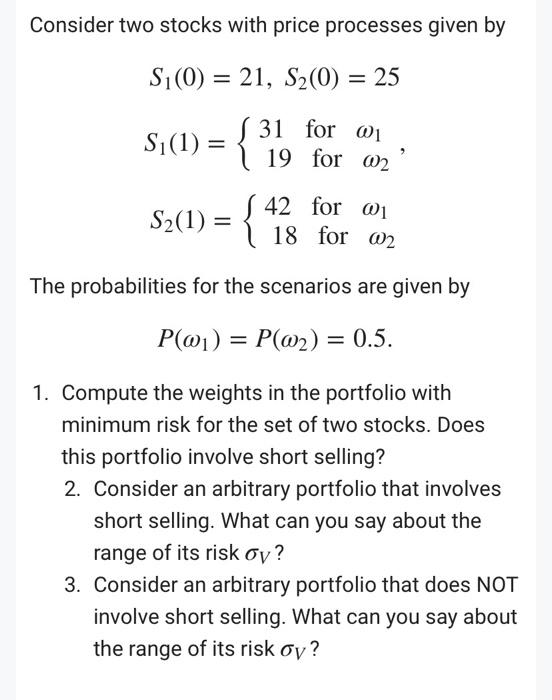
Consider two stocks with price processes given by Si(0) = 21, S2(0) = 25 \ 31 for 01 19 for m2 S2(1) = 42 for 01 18 for 02 The probabilities for the scenarios are given by P(01) = P(@2) = 0.5. 1. Compute the weights in the portfolio with minimum risk for the set of two stocks. Does this portfolio involve short selling? 2. Consider an arbitrary portfolio that involves short selling. What can you say about the range of its risk oy? 3. Consider an arbitrary portfolio that does NOT involve short selling. What can you say about the range of its risk oy? 2. Two Securities Proposition 2.1. Consider a portfolio consist- ing of N = 2 securities. Then, the expected return on the portfolio is E[Rv] = E[wRi+w2R2] = w1 E[R]+w2E[R2). The variance of the return on the portfolio is VAR[Rv] VAR[w1R1 + w2R2] wV AR[Ru] + w2V AR[R2] +2w1w2COV[R1, R2). Notation 2.1. We denote = = = 11 E[Ru] 12 E[R2] E[Ry] 01 SD[Ru] = VAR[R1] 02 SD[R2) = VVAR[R2] ov SD[Rv] = VVAR[Ry] C12 COV[R1, R2] The correlation coefficient is defined by C12 P12 := 0102 Remark 2.1. The correlation coefficient is undefined if 0102 = 0. This condition means that at least one of the assets is risk free. For two risky securities, 01 > 0 and 02 > 0. Thus, P12 is well defined for two risky securi- Proposition 2.2. Suppose that short sales are not allowed. Then, of s max(c1,02). Consider two stocks with price processes given by Si(0) = 21, S2(0) = 25 \ 31 for 01 19 for m2 S2(1) = 42 for 01 18 for 02 The probabilities for the scenarios are given by P(01) = P(@2) = 0.5. 1. Compute the weights in the portfolio with minimum risk for the set of two stocks. Does this portfolio involve short selling? 2. Consider an arbitrary portfolio that involves short selling. What can you say about the range of its risk oy? 3. Consider an arbitrary portfolio that does NOT involve short selling. What can you say about the range of its risk oy? 2. Two Securities Proposition 2.1. Consider a portfolio consist- ing of N = 2 securities. Then, the expected return on the portfolio is E[Rv] = E[wRi+w2R2] = w1 E[R]+w2E[R2). The variance of the return on the portfolio is VAR[Rv] VAR[w1R1 + w2R2] wV AR[Ru] + w2V AR[R2] +2w1w2COV[R1, R2). Notation 2.1. We denote = = = 11 E[Ru] 12 E[R2] E[Ry] 01 SD[Ru] = VAR[R1] 02 SD[R2) = VVAR[R2] ov SD[Rv] = VVAR[Ry] C12 COV[R1, R2] The correlation coefficient is defined by C12 P12 := 0102 Remark 2.1. The correlation coefficient is undefined if 0102 = 0. This condition means that at least one of the assets is risk free. For two risky securities, 01 > 0 and 02 > 0. Thus, P12 is well defined for two risky securi- Proposition 2.2. Suppose that short sales are not allowed. Then, of s max(c1,02)











