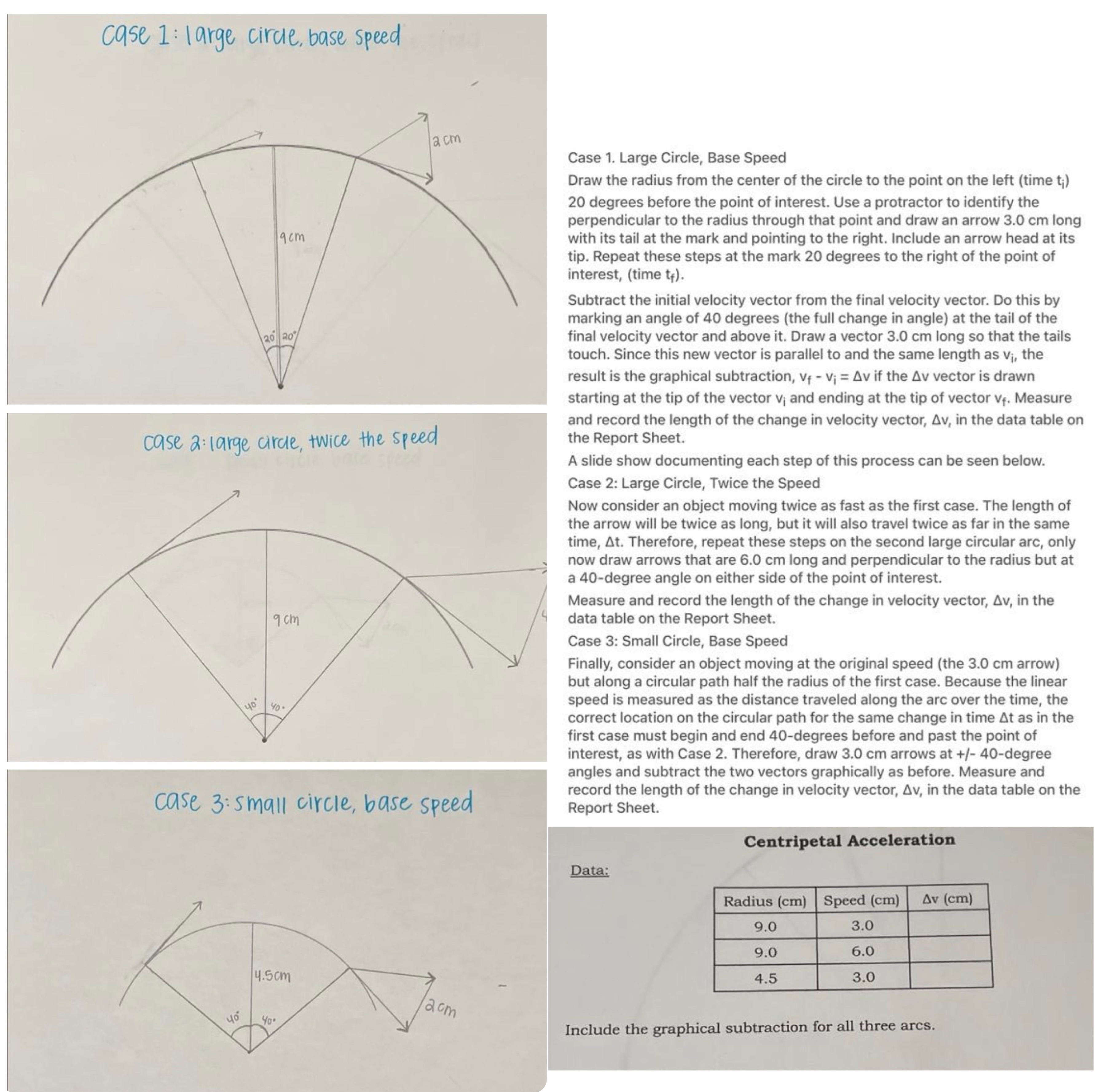
Need help filling in the data table including the graphical subtraction for all three arcs.

case 1 : large circle, base speed acm Case 1. Large Circle, Base Speed Draw the radius from the center of the circle to the point on the left (time t;) 20 degrees before the point of interest. Use a protractor to identify the perpendicular to the radius through that point and draw an arrow 3.0 cm long 9 cm with its tail at the mark and pointing to the right. Include an arrow head at its tip. Repeat these steps at the mark 20 degrees to the right of the point of interest, (time t). Subtract the initial velocity vector from the final velocity vector. Do this by marking an angle of 40 degrees (the full change in angle) at the tail of the 20 2 final velocity vector and above it. Draw a vector 3.0 cm long so that the tails touch. Since this new vector is parallel to and the same length as vi, the result is the graphical subtraction, V - Vi = Av if the Av vector is drawn starting at the tip of the vector vi and ending at the tip of vector v. Measure and record the length of the change in velocity vector, Av, in the data table on case 2 : large circle, twice the speed the Report Sheet. A slide show documenting each step of this process can be seen below. Case 2: Large Circle, Twice the Speed Now consider an object moving twice as fast as the first case. The length of the arrow will be twice as long, but it will also travel twice as far in the same time, At. Therefore, repeat these steps on the second large circular arc, only now draw arrows that are 6.0 cm long and perpendicular to the radius but at a 40-degree angle on either side of the point of interest. Measure and record the length of the change in velocity vector, Av, in the 9 cm data table on the Report Sheet. Case 3: Small Circle, Base Speed Finally, consider an object moving at the original speed (the 3.0 cm arrow) but along a circular path half the radius of the first case. Because the linear 10 40 speed is measured as the distance traveled along the arc over the time, the correct location on the circular path for the same change in time At as in the first case must begin and end 40-degrees before and past the point of interest, as with Case 2. Therefore, draw 3.0 cm arrows at +/- 40-degree angles and subtract the two vectors graphically as before. Measure and case 3:small circle, base speed record the length of the change in velocity vector, Av, in the data table on the Report Sheet. Centripetal Acceleration Data: Radius (cm) Speed (cm) Av (cm) 9.0 3.0 9.0 5.0 14.5 0m 4.5 3.0 2cm 40 Include the graphical subtraction for all three arcs