need help with this question asap . i attached some information that may be helpful.
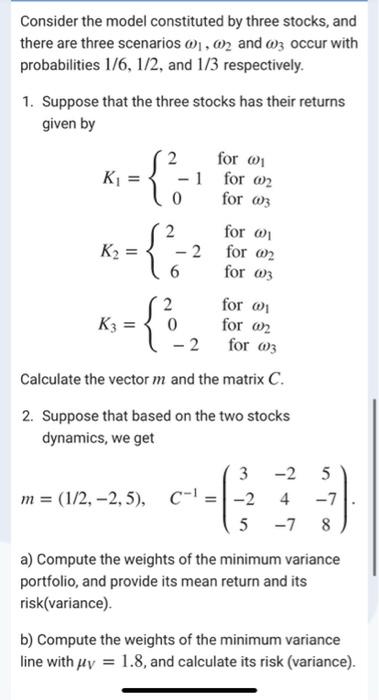
Consider the model constituted by three stocks, and there are three scenarios 0,02 and 03 occur with probabilities 1/6, 1/2, and 1/3 respectively. 1. Suppose that the three stocks has their returns given by for a K = 1 for 2 0 for 63 K2 = = 2 -2 6 for 1 for 02 for 03 2 for K3 = 0 for an 2 for 63 Calculate the vector m and the matrix C. { 2. Suppose that based on the two stocks dynamics, we get 4 -7 3 -2 5 m = (1/2, -2,5), c-' = -2 4 -7 5 8 a) Compute the weights of the minimum variance portfolio, and provide its mean return and its risk(variance) b) Compute the weights of the minimum variance line with My = 1.8, and calculate its risk (variance). 3. Several Securities Remark 3.1. The weights W1, W2, ...,WN of a portfolio constructed from N different risky securities can be arranged in a one-row matrix w = (w1, W2, ..., WN). The weights must satisfy N w; = 1, i=1 which is equivalent to wut = 1. Here, u= (1,1,...,1), and uT is the transpose of u. Definition 3.1.[Feasible Set] The feasible set consists of all portfolios with weights w satis- fying wut = 1. These portfolios are the feasible portfolios. Notation 3.1. The returns on the N risky securities are denoted by R1, R2, ..., Rn. The expected returns will be arranged into a one-row matrix m = (M1, M2,...,un). That is, Hi = E[R] Notation 3.2. The covariances between re- turns will be denoted by Cij = COV[Ri, R;]. They can be arranged in the covariance matrix C11 C12 CIN C21 C22 C2N C = : : CN1 CN2 CNN We note that the diagonal elements are COV[Ri, R;] = VAR[R;] = 0?. The covariance matrix is symmetric and non- negative definite. Assumption 3.1. We will assume that det(C) = 0, which implies that has an inverse C-1. It also imnlies that both cand c-l are nositive Definition 3.2.[MVP] The minimum variance portfolio (MVP) is the portfolio with the small- est variance among all feasible portfolios. Proposition 3.1. Consider the return Ry = N wnRn. n=1 on a portfolio with value V and weights w = (w1, W2, ...,wN). Then, 03 E[Ry] =wmt VAR[Ry] =wCwT. 26 Theorem 3.1.[MVP) Assume det(C) = 0. Then, the minimum variance portfolio has weights UC-1 WMVP = UC-14T Definition 3.6.[MVL) The minimum variance line is the family of portfolios with value V, parameterized by u ER, such that HV = and for every portfolio with hi =u: of so Theorem 3.2.[MVL] Assume that det(C) E 0, and m and u are linearly independent vec- tors. Then, we RN represents a portfolio (with value V) on the minimum variance line if and only if 2w = limc-1 + 12uc-1. Here, 11 and 12 are given by (??)-(??) with | = ly, Equivalently, we RN represents a portfolio (with value V) on the minimum variance line V) if and only if w=pa +b, where a and b depend only on C, m and u. Consider the model constituted by three stocks, and there are three scenarios 0,02 and 03 occur with probabilities 1/6, 1/2, and 1/3 respectively. 1. Suppose that the three stocks has their returns given by for a K = 1 for 2 0 for 63 K2 = = 2 -2 6 for 1 for 02 for 03 2 for K3 = 0 for an 2 for 63 Calculate the vector m and the matrix C. { 2. Suppose that based on the two stocks dynamics, we get 4 -7 3 -2 5 m = (1/2, -2,5), c-' = -2 4 -7 5 8 a) Compute the weights of the minimum variance portfolio, and provide its mean return and its risk(variance) b) Compute the weights of the minimum variance line with My = 1.8, and calculate its risk (variance). 3. Several Securities Remark 3.1. The weights W1, W2, ...,WN of a portfolio constructed from N different risky securities can be arranged in a one-row matrix w = (w1, W2, ..., WN). The weights must satisfy N w; = 1, i=1 which is equivalent to wut = 1. Here, u= (1,1,...,1), and uT is the transpose of u. Definition 3.1.[Feasible Set] The feasible set consists of all portfolios with weights w satis- fying wut = 1. These portfolios are the feasible portfolios. Notation 3.1. The returns on the N risky securities are denoted by R1, R2, ..., Rn. The expected returns will be arranged into a one-row matrix m = (M1, M2,...,un). That is, Hi = E[R] Notation 3.2. The covariances between re- turns will be denoted by Cij = COV[Ri, R;]. They can be arranged in the covariance matrix C11 C12 CIN C21 C22 C2N C = : : CN1 CN2 CNN We note that the diagonal elements are COV[Ri, R;] = VAR[R;] = 0?. The covariance matrix is symmetric and non- negative definite. Assumption 3.1. We will assume that det(C) = 0, which implies that has an inverse C-1. It also imnlies that both cand c-l are nositive Definition 3.2.[MVP] The minimum variance portfolio (MVP) is the portfolio with the small- est variance among all feasible portfolios. Proposition 3.1. Consider the return Ry = N wnRn. n=1 on a portfolio with value V and weights w = (w1, W2, ...,wN). Then, 03 E[Ry] =wmt VAR[Ry] =wCwT. 26 Theorem 3.1.[MVP) Assume det(C) = 0. Then, the minimum variance portfolio has weights UC-1 WMVP = UC-14T Definition 3.6.[MVL) The minimum variance line is the family of portfolios with value V, parameterized by u ER, such that HV = and for every portfolio with hi =u: of so Theorem 3.2.[MVL] Assume that det(C) E 0, and m and u are linearly independent vec- tors. Then, we RN represents a portfolio (with value V) on the minimum variance line if and only if 2w = limc-1 + 12uc-1. Here, 11 and 12 are given by (??)-(??) with | = ly, Equivalently, we RN represents a portfolio (with value V) on the minimum variance line V) if and only if w=pa +b, where a and b depend only on C, m and u

















