Need urgent help with this economics problem. Will appreciate all of your help.
Please show all the necessary work. Thank you.
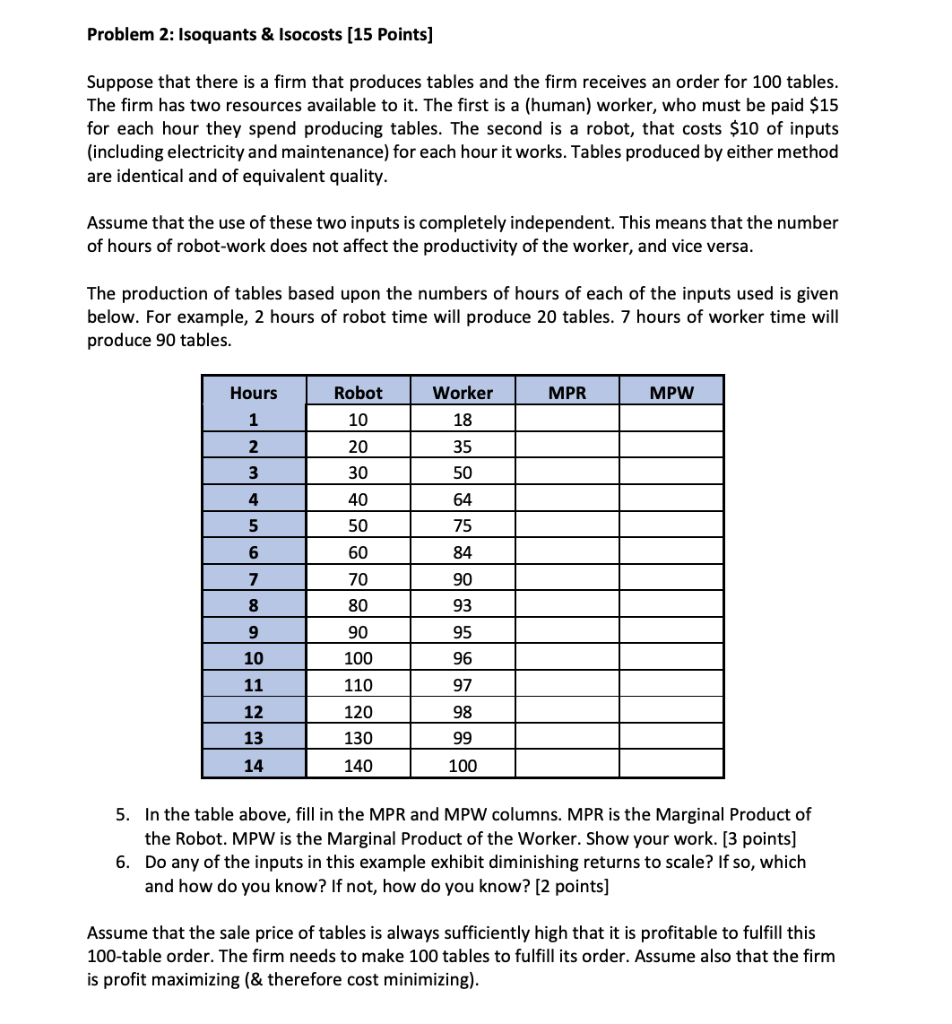

Problem 2: Isoquants & Isocosts (15 Points) Suppose that there is a firm that produces tables and the firm receives an order for 100 tables. The firm has two resources available to it. The first is a (human) worker, who must be paid $15 for each hour they spend producing tables. The second is a robot, that costs $10 of inputs (including electricity and maintenance) for each hour it works. Tables produced by either method are identical and of equivalent quality. Assume that the use of these two inputs is completely independent. This means that the number of hours of robot-work does not affect the productivity of the worker, and vice versa. The production of tables based upon the numbers of hours of each of the inputs used is given below. For example, 2 hours of robot time will produce 20 tables. 7 hours of worker time will produce 90 tables. Robot Worker MPR MPW Hours 1 10 18 2 20 35 3 30 50 64 4 40 5 50 75 84 6 60 7 70 90 8 93 80 90 9 95 10 100 110 96 97 11 98 12 13 120 130 99 14 140 100 5. In the table above, fill in the MPR and MPW columns. MPR is the Marginal Product of the Robot. MPW is the Marginal Product of the Worker. Show your work. [3 points) 6. Do any of the inputs in this example exhibit diminishing returns to scale? If so, which and how do you know? If not, how do you know? [2 points) Assume that the sale price of tables is always sufficiently high that it is profitable to fulfill this 100-table order. The firm needs to make 100 tables to fulfill its order. Assume also that the firm is profit maximizing (& therefore cost minimizing). 7. What combinations of robot and worker hours must they use to minimize costs? Show your work. [Use the equation that must be true for cost minimization for full credit] [4 points) Now suppose that there is a major shortage of mechanics in the local economy, and the price of an hour of robot work increases (dramatically) from $10 to $50. 8. What does the principle of substitution say should happen to the firm's use of (i) worker hours and (ii) robot hours? Explain your answer. [2 points] Continue to assume that it will be profitable to produce the 100 tables and that the firm is profit-maximizing. 9. With this new price for robot hours, what is the new combination of robot and worker hours that will minimize the cost of producing 100 tables? Show your work. [Use the equation that must be true for cost minimization for full credit] [4 points] Problem 2: Isoquants & Isocosts (15 Points) Suppose that there is a firm that produces tables and the firm receives an order for 100 tables. The firm has two resources available to it. The first is a (human) worker, who must be paid $15 for each hour they spend producing tables. The second is a robot, that costs $10 of inputs (including electricity and maintenance) for each hour it works. Tables produced by either method are identical and of equivalent quality. Assume that the use of these two inputs is completely independent. This means that the number of hours of robot-work does not affect the productivity of the worker, and vice versa. The production of tables based upon the numbers of hours of each of the inputs used is given below. For example, 2 hours of robot time will produce 20 tables. 7 hours of worker time will produce 90 tables. Robot Worker MPR MPW Hours 1 10 18 2 20 35 3 30 50 64 4 40 5 50 75 84 6 60 7 70 90 8 93 80 90 9 95 10 100 110 96 97 11 98 12 13 120 130 99 14 140 100 5. In the table above, fill in the MPR and MPW columns. MPR is the Marginal Product of the Robot. MPW is the Marginal Product of the Worker. Show your work. [3 points) 6. Do any of the inputs in this example exhibit diminishing returns to scale? If so, which and how do you know? If not, how do you know? [2 points) Assume that the sale price of tables is always sufficiently high that it is profitable to fulfill this 100-table order. The firm needs to make 100 tables to fulfill its order. Assume also that the firm is profit maximizing (& therefore cost minimizing). 7. What combinations of robot and worker hours must they use to minimize costs? Show your work. [Use the equation that must be true for cost minimization for full credit] [4 points) Now suppose that there is a major shortage of mechanics in the local economy, and the price of an hour of robot work increases (dramatically) from $10 to $50. 8. What does the principle of substitution say should happen to the firm's use of (i) worker hours and (ii) robot hours? Explain your answer. [2 points] Continue to assume that it will be profitable to produce the 100 tables and that the firm is profit-maximizing. 9. With this new price for robot hours, what is the new combination of robot and worker hours that will minimize the cost of producing 100 tables? Show your work. [Use the equation that must be true for cost minimization for full credit] [4 points]








