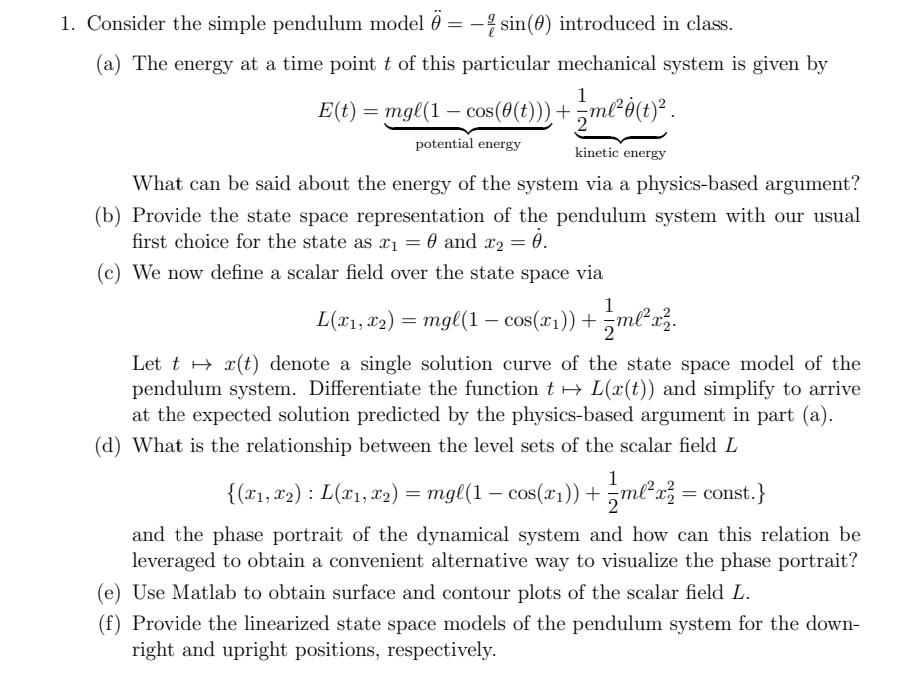
1. Consider the simple pendulum model = - sin(0) introduced in class. (a) The energy at a time point t of this particular mechanical system is given by 1 = mgl(1 cos(0(t)) + ml8(t). potential energy E(t) = kinetic energy What can be said about the energy of the system via a physics-based argument? (b) Provide the state space representation of the pendulum system with our usual first choice for the state as x= 0 and x2 = = 0. (c) We now define a scalar field over the state space via 1 L(x, x2) = mgl(1 cos(x)) + - mlx. Let tx(t) denote a single solution curve of the state space model of the pendulum system. Differentiate the function t L(x(t)) and simplify to arrive at the expected solution predicted by the physics-based argument in part (a). (d) What is the relationship between the level sets of the scalar field L 1 {(x1, x2) : L(x1, x2) = mgl (1 cos(x)) + =mlx = const.} and the phase portrait of the dynamical system and how can this relation be leveraged to obtain a convenient alternative way to visualize the phase portrait? (e) Use Matlab to obtain surface and contour plots of the scalar field L. (f) Provide the linearized state space models of the pendulum system for the down- right and upright positions, respectively.