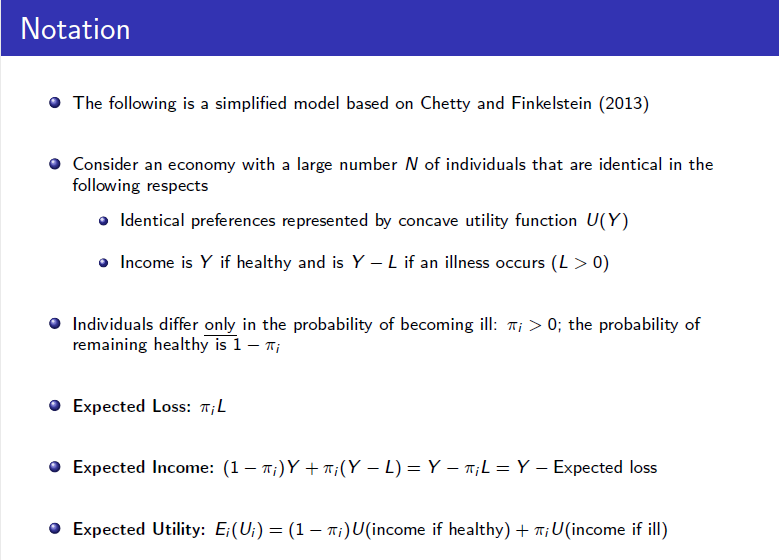
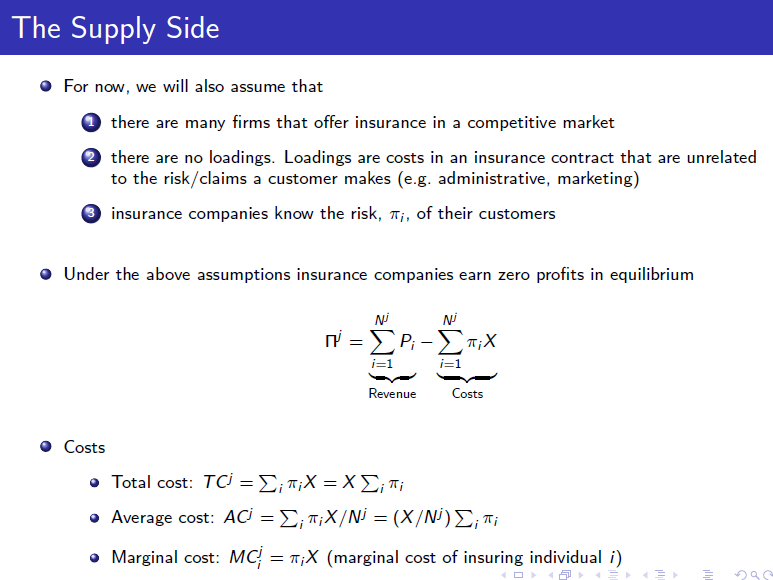
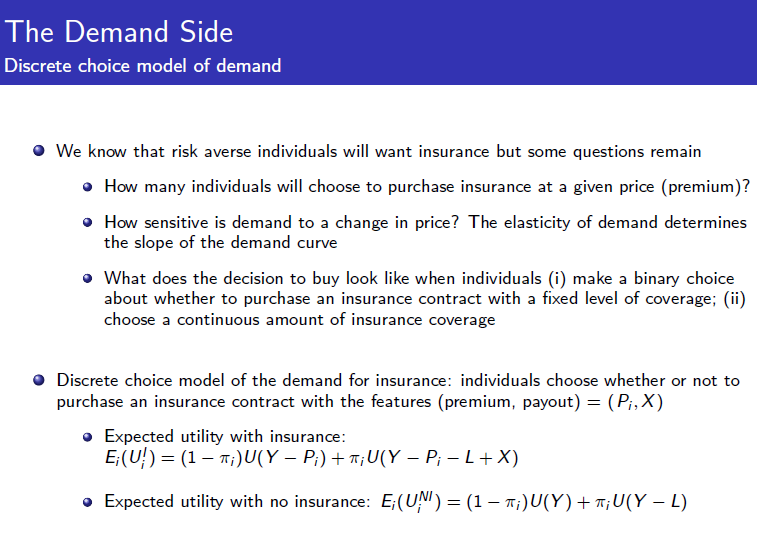
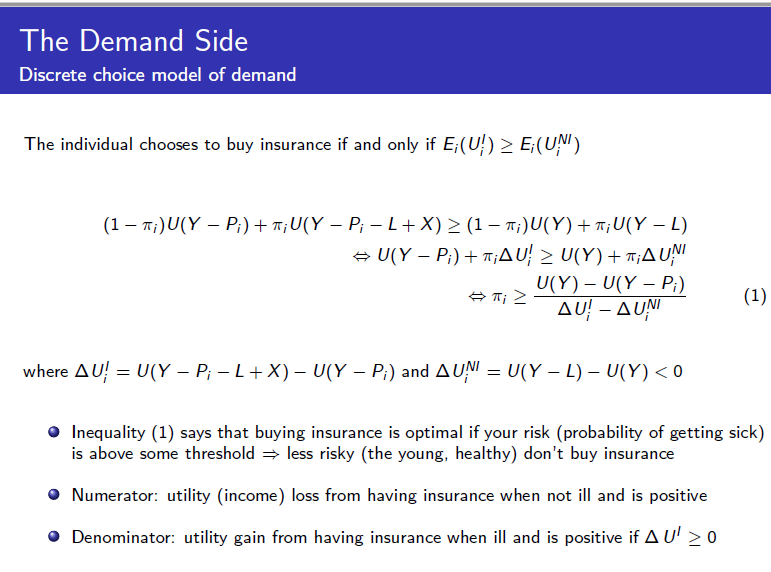
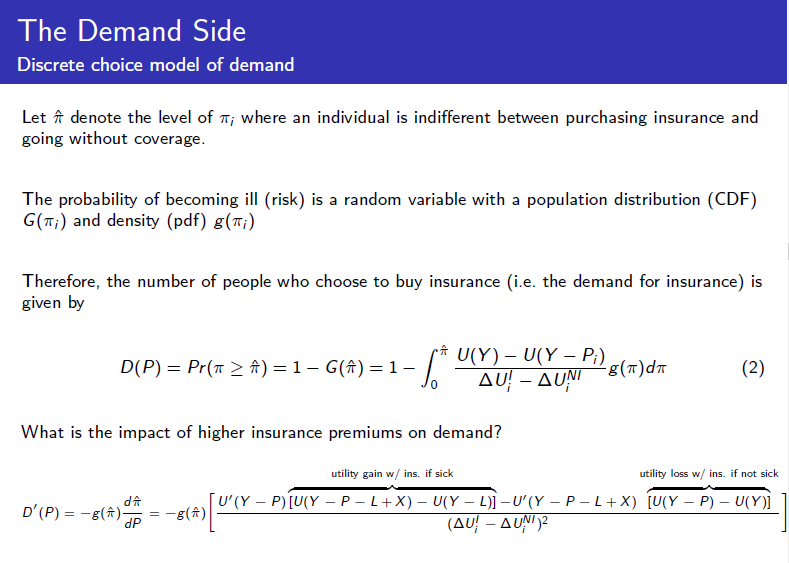
Notation The following is a simplified model based on Chetty and Finkelstein (2013) Consider an economy with a large number N of individuals that are identical in the following respects Identical preferences represented by concave utility function U(Y) Income is Y if healthy and is Y - L if an illness occurs (L > 0) Individuals differ only in the probability of becoming ill: ti >0; the probability of remaining healthy is 1 - 1; Expected Loss: T;L Expected Income: (1 ;) Y +T;(Y L) = Y 7;L= Y - Expected loss Expected Utility: E;(Ui) = (1 Ti)U(income if healthy) + ;U(income if ill) The Supply Side . For now, we will also assume that there are many firms that offer insurance in a competitive market there are no loadings. Loadings are costs in an insurance contract that are unrelated to the risk/claims a customer makes (e.g. administrative, marketing) insurance companies know the risk, T;, of their customers Under the above assumptions insurance companies earn zero profits in equilibrium N t= : - ; WIJ T;X i=1 i=1 Revenue Costs Costs Total cost: TCI = ;;X = Xin; . Average cost: AC) = L;4;X/N} = (X/N) ;T; Marginal cost: MC = 7;X (marginal cost of insuring individual i) = = = The Demand Side Discrete choice model of demand We know that risk averse individuals will want insurance but some questions remain How many individuals will choose to purchase insurance at a given price (premium)? How sensitive is demand to a change in price? The elasticity of demand determines the slope of the demand curve What does the decision to buy look like when individuals (i) make a binary choice about whether to purchase an insurance contract with a fixed level of coverage; (ii) choose a continuous amount of insurance coverage Discrete choice model of the demand for insurance: individuals choose whether or not to purchase an insurance contract with the features (premium, payout) = (Pi, X) Expected utility with insurance: E;(UA) = (1 T;)U(Y P;) +7;U(Y - P;-[+X) Expected utility with no insurance: E;(UN) = (1 7;)U(Y) + 7;U(Y L) = The Demand Side Discrete choice model of demand The individual chooses to buy insurance if and only if E;(U!) 2 E;(UN) (1 7;)U(Y P;) + 7;U(Y P; -L+X) (1 7;)U(Y) +7;U(Y L) U(Y P;) + ;AU! > N(Y)+7;AUN U(Y)- U(Y P;) i? U! 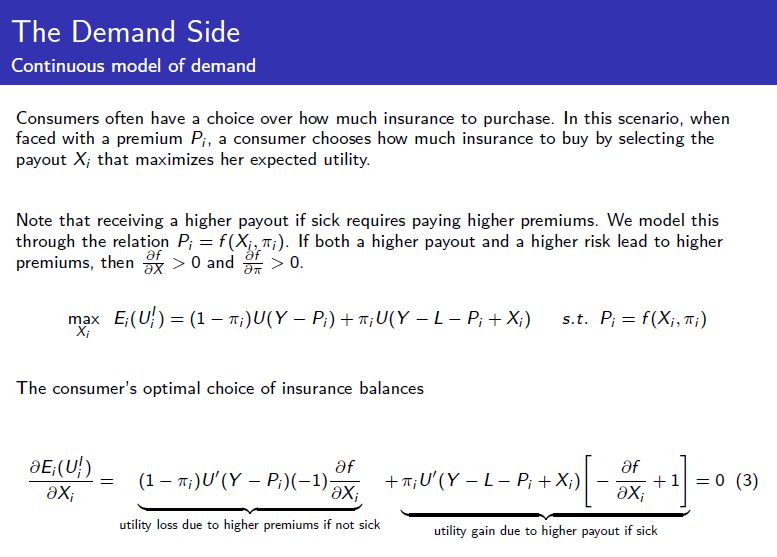UNI (1) where AV} = U(Y P; - [+X) U(Y P;) and AUN = U(Y L) U(Y) )=1 - G(A)=1- -6* U(Y) - U(Y - P:) -g(7) U! UNI (2) What is the impact of higher insurance premiums on demand? dA D'(P) = -g(A) utility gain w/ ins. if sick utility loss w/ ins. if not sick U'(Y P) (U(Y - P-[+X) - U(Y L)] - U'(Y - P-[+x) (U(Y P) U(Y)] (AU - AUN)2 [ur = -8(A) dP The Demand Side Continuous model of demand Consumers often have a choice over how much insurance to purchase. In this scenario, when faced with a premium Pi, a consumer chooses how much insurance to buy by selecting the payout X; that maximizes her expected utility. Note that receiving a higher payout if sick requires paying higher premiums. We model this through the relation P; =f(X, T;). If both a higher payout and a higher risk lead to higher af premiums, then a > 0 and > 0. max E;(UX) = (1 7;)U(Y P;) + ;U(Y - [ Pi + X;) - s.t. P; = f(X;, ;) Xi The consumer's optimal choice of insurance balances E (U) ax; = af (1 7;)U'(Y P:)(-1), axi +7;U'(Y -L-P; +X;) af +1 ax; = 0 (3) utility loss due to higher premiums if not sick utility gain due to higher payout if sick Consider an economy with N (large number) individuals that each face the possibility of a negative income shock. In the good state of the world in- come is y and if a negative shock occurs income is y - L; L> 0 is the in- come shock. Assume that individuals have the same preferences over in- come/consumption that can be represented by the strictly concave utility yl- function u(y) 4-6. Some individuals are more likely than others to experience a negative income shock. The probability that individual i be- comes experiences a negative income shock is is Tia E 0,1); i does not face an income shock with probability 1 - T. The random variable is distributed continuously across the population. Let g(1) denote the density of and G() the CDF. Assume that the probability that one individual experiences a negative income shock is independent of the probability that any other individual experiences an income shock. A large number of perfectly competitive insurance companies offer contracts that feature full insurance to individuals. Insurance contracts have two fea- tures: a premium p a payout x = L that is paid to the individual in the event of an income shock. The potential risk of individuals is private information and is not observed by insurers. Individuals choose whether or not to purchase insurance taking features of the insurance contract offered by the insurance companies as given. (a) Consider the choice facing individual j with risk tj. She is offered a contract with a premium p and a payout r= L and must choose whether or not to purchase insurance. (i) Write down her expected utility for the insurance and no insurance cases; (ii) Under what condition will she decide to purchase insurance? [2 marks] (b) What is the demand for insurance in this economy? In other words, how many individuals choose to purchase insurance? [1 mark] (c) Show that the demand for insurance is decreasing in the insurance premium p. Derive an expression of the elasticity of demand for insurance with respect to p. Your answers should be expressed in terms of the model's primitives (i.e. 7,90.), G.). [2 marks] (d) What is the marginal cost to the insurance company from insuring individual j? [1 mark (e) Suppose that the number of individuals that choose to purchase insurance is Dp). Because of perfect competition, all insurance companies offer contracts that charge the same premium. Use the above two facts to derive the profit maximizing premium/price of insurance. [1 mark] (f) Show (analytically) that a social planner would insure more people than a competitive market. [3 marks Notation The following is a simplified model based on Chetty and Finkelstein (2013) Consider an economy with a large number N of individuals that are identical in the following respects Identical preferences represented by concave utility function U(Y) Income is Y if healthy and is Y - L if an illness occurs (L > 0) Individuals differ only in the probability of becoming ill: ti >0; the probability of remaining healthy is 1 - 1; Expected Loss: T;L Expected Income: (1 ;) Y +T;(Y L) = Y 7;L= Y - Expected loss Expected Utility: E;(Ui) = (1 Ti)U(income if healthy) + ;U(income if ill) The Supply Side . For now, we will also assume that there are many firms that offer insurance in a competitive market there are no loadings. Loadings are costs in an insurance contract that are unrelated to the risk/claims a customer makes (e.g. administrative, marketing) insurance companies know the risk, T;, of their customers Under the above assumptions insurance companies earn zero profits in equilibrium N t= : - ; WIJ T;X i=1 i=1 Revenue Costs Costs Total cost: TCI = ;;X = Xin; . Average cost: AC) = L;4;X/N} = (X/N) ;T; Marginal cost: MC = 7;X (marginal cost of insuring individual i) = = = The Demand Side Discrete choice model of demand We know that risk averse individuals will want insurance but some questions remain How many individuals will choose to purchase insurance at a given price (premium)? How sensitive is demand to a change in price? The elasticity of demand determines the slope of the demand curve What does the decision to buy look like when individuals (i) make a binary choice about whether to purchase an insurance contract with a fixed level of coverage; (ii) choose a continuous amount of insurance coverage Discrete choice model of the demand for insurance: individuals choose whether or not to purchase an insurance contract with the features (premium, payout) = (Pi, X) Expected utility with insurance: E;(UA) = (1 T;)U(Y P;) +7;U(Y - P;-[+X) Expected utility with no insurance: E;(UN) = (1 7;)U(Y) + 7;U(Y L) = The Demand Side Discrete choice model of demand The individual chooses to buy insurance if and only if E;(U!) 2 E;(UN) (1 7;)U(Y P;) + 7;U(Y P; -L+X) (1 7;)U(Y) +7;U(Y L) U(Y P;) + ;AU! > N(Y)+7;AUN U(Y)- U(Y P;) i? U! UNI (1) where AV} = U(Y P; - [+X) U(Y P;) and AUN = U(Y L) U(Y) )=1 - G(A)=1- -6* U(Y) - U(Y - P:) -g(7) U! UNI (2) What is the impact of higher insurance premiums on demand? dA D'(P) = -g(A) utility gain w/ ins. if sick utility loss w/ ins. if not sick U'(Y P) (U(Y - P-[+X) - U(Y L)] - U'(Y - P-[+x) (U(Y P) U(Y)] (AU - AUN)2 [ur = -8(A) dP The Demand Side Continuous model of demand Consumers often have a choice over how much insurance to purchase. In this scenario, when faced with a premium Pi, a consumer chooses how much insurance to buy by selecting the payout X; that maximizes her expected utility. Note that receiving a higher payout if sick requires paying higher premiums. We model this through the relation P; =f(X, T;). If both a higher payout and a higher risk lead to higher af premiums, then a > 0 and > 0. max E;(UX) = (1 7;)U(Y P;) + ;U(Y - [ Pi + X;) - s.t. P; = f(X;, ;) Xi The consumer's optimal choice of insurance balances E (U) ax; = af (1 7;)U'(Y P:)(-1), axi +7;U'(Y -L-P; +X;) af +1 ax; = 0 (3) utility loss due to higher premiums if not sick utility gain due to higher payout if sick Consider an economy with N (large number) individuals that each face the possibility of a negative income shock. In the good state of the world in- come is y and if a negative shock occurs income is y - L; L> 0 is the in- come shock. Assume that individuals have the same preferences over in- come/consumption that can be represented by the strictly concave utility yl- function u(y) 4-6. Some individuals are more likely than others to experience a negative income shock. The probability that individual i be- comes experiences a negative income shock is is Tia E 0,1); i does not face an income shock with probability 1 - T. The random variable is distributed continuously across the population. Let g(1) denote the density of and G() the CDF. Assume that the probability that one individual experiences a negative income shock is independent of the probability that any other individual experiences an income shock. A large number of perfectly competitive insurance companies offer contracts that feature full insurance to individuals. Insurance contracts have two fea- tures: a premium p a payout x = L that is paid to the individual in the event of an income shock. The potential risk of individuals is private information and is not observed by insurers. Individuals choose whether or not to purchase insurance taking features of the insurance contract offered by the insurance companies as given. (a) Consider the choice facing individual j with risk tj. She is offered a contract with a premium p and a payout r= L and must choose whether or not to purchase insurance. (i) Write down her expected utility for the insurance and no insurance cases; (ii) Under what condition will she decide to purchase insurance? [2 marks] (b) What is the demand for insurance in this economy? In other words, how many individuals choose to purchase insurance? [1 mark] (c) Show that the demand for insurance is decreasing in the insurance premium p. Derive an expression of the elasticity of demand for insurance with respect to p. Your answers should be expressed in terms of the model's primitives (i.e. 7,90.), G.). [2 marks] (d) What is the marginal cost to the insurance company from insuring individual j? [1 mark (e) Suppose that the number of individuals that choose to purchase insurance is Dp). Because of perfect competition, all insurance companies offer contracts that charge the same premium. Use the above two facts to derive the profit maximizing premium/price of insurance. [1 mark] (f) Show (analytically) that a social planner would insure more people than a competitive market. [3 marks