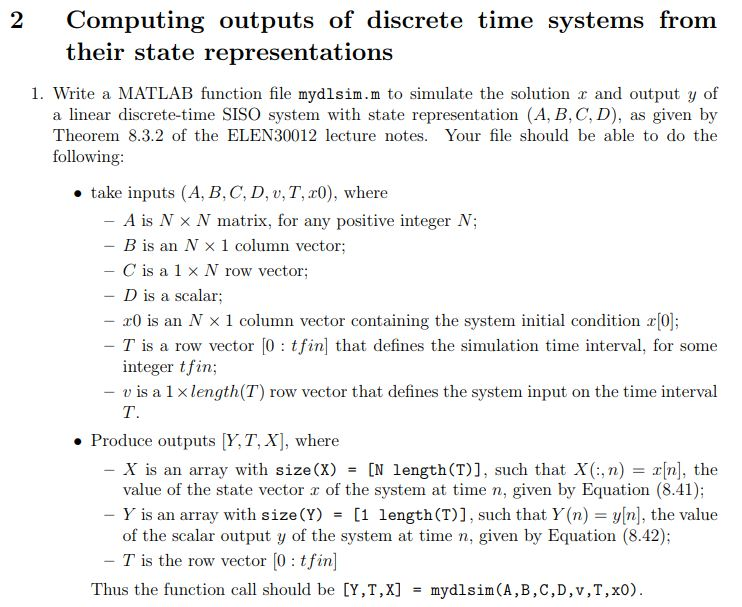
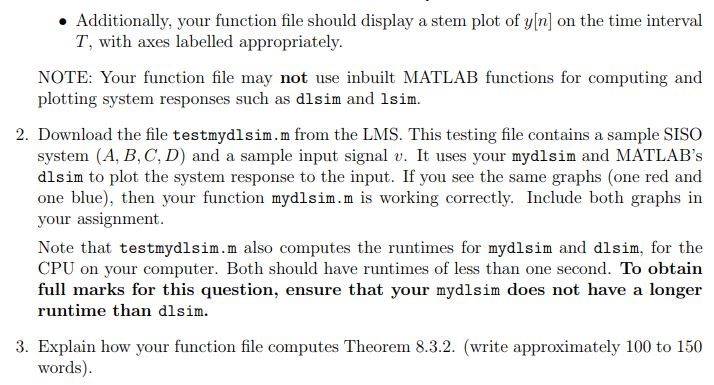
NOTE: DO Q1, Q2,

REFERENCE:

The matlab script file mydlsim.m :-

% Testing file for Question 1, Assignment 3, 2018. clear all close all A = [-.6 1; 0 0.2 ]; B = [1 ;0 ]; C = [1 0 ]; D = 3;

x0 = [ -1 -1]' ; tfin = 50 T = [0:tfin]; v= cos(T/10); tic [Y,T,X] = mydlsim(A,B,C,D,v,T,x0); mydlsim_runtime = toc

tic [Y1,X1] = dlsim(A,B,C,D,v,x0) ; figure(2) T1 = [0:length(v)-1]; stem(T1,Y1,'r') xlabel('n') ylabel('y[n] using dlsim') dlsim_runtime= toc

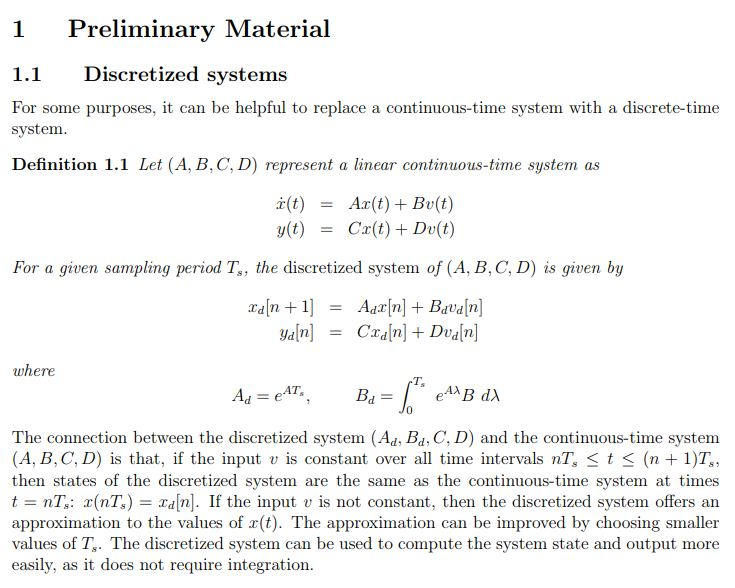
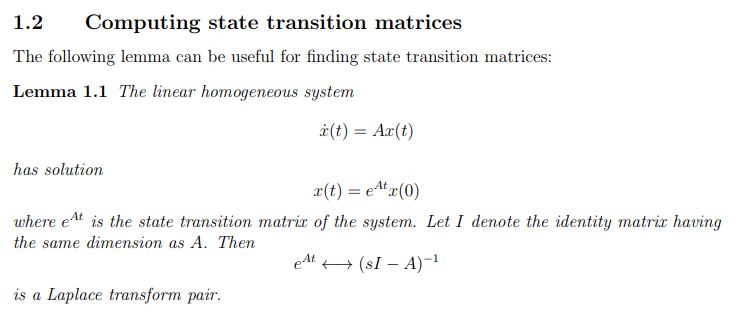
1 Preliminary Material 1.1 Discretized systems For some purposes, it can be helpful to replace a continuous-time system with a discrete-time system Definition 1.1 Let (A, B, C, D) represent a linear continuous-time system as (t) = A2(t)+Bu(t) y(t) - Ca(t) D(t) For a given sampling period Ts, the discretized system of (A, B,C, D) is given by where Ts de-4T 0 The connection between the discretized system (Ad, Bd, C, D) and the continuous-time system (A, B, C, D) is that, if the input v is constant over all time intervals nT, t (n 1)T, then states of the discretized system are the same as the continuous-time system at times t = nT,: x(nT,) = xd[n]. If the input u is not constant, then the discretized system offers an approximation to the values of r(t). The approximation can be improved by choosing smaller values of T,. The discretized system can be used to compute the system state and output more easily, as it does not require integration 1 Preliminary Material 1.1 Discretized systems For some purposes, it can be helpful to replace a continuous-time system with a discrete-time system Definition 1.1 Let (A, B, C, D) represent a linear continuous-time system as (t) = A2(t)+Bu(t) y(t) - Ca(t) D(t) For a given sampling period Ts, the discretized system of (A, B,C, D) is given by where Ts de-4T 0 The connection between the discretized system (Ad, Bd, C, D) and the continuous-time system (A, B, C, D) is that, if the input v is constant over all time intervals nT, t (n 1)T, then states of the discretized system are the same as the continuous-time system at times t = nT,: x(nT,) = xd[n]. If the input u is not constant, then the discretized system offers an approximation to the values of r(t). The approximation can be improved by choosing smaller values of T,. The discretized system can be used to compute the system state and output more easily, as it does not require integration